
Illustrative Math Alignment: Grade 6 Unit 1
Dividing Fractions
Lesson 17: Fitting Boxes into Boxes
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Nodes |
|---|---|---|---|

|
Math Example: Fraction Operations--Multiplying Fractions and Whole Numbers Using Models: Example 3 | Multiplying Fractions and Whole Numbers Using Models: Example 3TopicFraction Operations |
Fractions and Mixed Numbers |
|
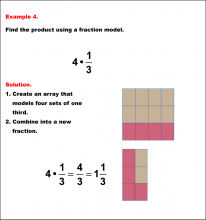
Math Example: Fraction Operations--Multiplying Fractions and Whole Numbers Using Models: Example 4 | Multiplying Fractions and Whole Numbers Using Models: Example 4TopicFraction Operations |
Fractions and Mixed Numbers |
|
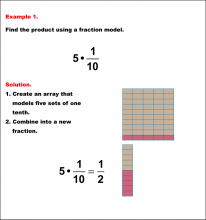
Math Example: Fraction Operations--Multiplying Fractions and Whole Numbers Using Models: Example 1 | Multiplying Fractions and Whole Numbers Using Models: Example 1TopicFraction Operations |
Fractions and Mixed Numbers |

|
Math Example: Fraction Operations--Multiplying Fractions and Whole Numbers Using Models: Example 5 | Math Example: Fraction Operations--Multiplying Fractions and Whole Numbers Using Models: Example 5TopicFraction Operations |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 2 | Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 2
This is part of a collection of math examples that explore different properties of fractions. This includes modeling fractions, simplifying fractions, and comparing them. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 3 | Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 3
This is part of a collection of math examples that explore different properties of fractions. This includes modeling fractions, simplifying fractions, and comparing them. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 4 | Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 4
This is part of a collection of math examples that explore different properties of fractions. This includes modeling fractions, simplifying fractions, and comparing them. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 7 | Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 7
This is part of a collection of math examples that explore different properties of fractions. This includes modeling fractions, simplifying fractions, and comparing them. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 5 | Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 5
This is part of a collection of math examples that explore different properties of fractions. This includes modeling fractions, simplifying fractions, and comparing them. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 6 | Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 6
This is part of a collection of math examples that explore different properties of fractions. This includes modeling fractions, simplifying fractions, and comparing them. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 1 | Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 1
This is part of a collection of math examples that explore different properties of fractions. This includes modeling fractions, simplifying fractions, and comparing them. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 8 | Math Example--Fraction Properties--Adding Mixed Numbers with Like Denominators: Example 8
This is part of a collection of math examples that explore different properties of fractions. This includes modeling fractions, simplifying fractions, and comparing them. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 7 | Generating Equivalent Fractions: Example 7TopicFractions DescriptionThis example focuses on the method of generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By multiplying by the same number, the fraction is effectively multiplied by 1, maintaining its original value. This technique is crucial for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and logical reasoning to ensure the fractions remain equivalent. This understanding is foundational for more advanced mathematical concepts such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 3 | Generating Equivalent Fractions: Example 3TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 7 | Generating Equivalent Fractions: Example 7TopicFractions DescriptionThis example focuses on the method of generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By multiplying by the same number, the fraction is effectively multiplied by 1, maintaining its original value. This technique is crucial for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and logical reasoning to ensure the fractions remain equivalent. This understanding is foundational for more advanced mathematical concepts such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 10 | Generating Equivalent Fractions: Example 10TopicFractions DescriptionThis example focuses on the method of generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By multiplying by the same number, the fraction is effectively multiplied by 1, maintaining its original value. This technique is crucial for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and logical reasoning to ensure the fractions remain equivalent. This understanding is foundational for more advanced mathematical concepts such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 1 | Generating Equivalent Fractions: Example 1TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 2 | Generating Equivalent Fractions: Example 2TopicFractions DescriptionThis example focuses on generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By doing so, the fraction is effectively multiplied by 1, preserving its original value while altering its form. This method is essential for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and applying logical reasoning to ensure the fractions remain equivalent. Mastery of this concept is vital for more advanced mathematical topics such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 6 | Generating Equivalent Fractions: Example 6TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 9 | Generating Equivalent Fractions: Example 9TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 10 | Generating Equivalent Fractions: Example 10TopicFractions DescriptionThis example focuses on the method of generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By multiplying by the same number, the fraction is effectively multiplied by 1, maintaining its original value. This technique is crucial for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and logical reasoning to ensure the fractions remain equivalent. This understanding is foundational for more advanced mathematical concepts such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 12 | Generating Equivalent Fractions: Example 12TopicFractions DescriptionThis example focuses on the method of generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By multiplying by the same number, the fraction is effectively multiplied by 1, maintaining its original value. This technique is crucial for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and logical reasoning to ensure the fractions remain equivalent. This understanding is foundational for more advanced mathematical concepts such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 3 | Generating Equivalent Fractions: Example 3TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 13 | Generating Equivalent Fractions: Example 13TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 2 | Generating Equivalent Fractions: Example 2TopicFractions DescriptionThis example focuses on generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By doing so, the fraction is effectively multiplied by 1, preserving its original value while altering its form. This method is essential for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and applying logical reasoning to ensure the fractions remain equivalent. Mastery of this concept is vital for more advanced mathematical topics such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 4 | Generating Equivalent Fractions: Example 4TopicFractions DescriptionThis example focuses on the method of generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By multiplying by the same number, the fraction is effectively multiplied by 1, maintaining its original value. This technique is crucial for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and logical reasoning to ensure the fractions remain equivalent. This understanding is foundational for more advanced mathematical concepts such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 8 | Generating Equivalent Fractions: Example 8TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 8 | Generating Equivalent Fractions: Example 8TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 6 | Generating Equivalent Fractions: Example 6TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 11 | Generating Equivalent Fractions: Example 11TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 4 | Generating Equivalent Fractions: Example 4TopicFractions DescriptionThis example focuses on the method of generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By multiplying by the same number, the fraction is effectively multiplied by 1, maintaining its original value. This technique is crucial for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and logical reasoning to ensure the fractions remain equivalent. This understanding is foundational for more advanced mathematical concepts such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 12 | Generating Equivalent Fractions: Example 12TopicFractions DescriptionThis example focuses on the method of generating equivalent fractions by applying a scale factor to both the numerator and the denominator. By multiplying by the same number, the fraction is effectively multiplied by 1, maintaining its original value. This technique is crucial for simplifying fractions and understanding their properties. The skills involved include multiplication, recognizing equivalent fractions, and logical reasoning to ensure the fractions remain equivalent. This understanding is foundational for more advanced mathematical concepts such as algebra and calculus. |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 9 | Generating Equivalent Fractions: Example 9TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 5 | Generating Equivalent Fractions: Example 5TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 11 | Generating Equivalent Fractions: Example 11TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 13 | Generating Equivalent Fractions: Example 13TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 5 | Generating Equivalent Fractions: Example 5TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Generating Equivalent Fractions: Example 1 | Generating Equivalent Fractions: Example 1TopicFractions |
Find Equivalent Fractions and Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Comparing Fraction Products: Example 6 | Comparing Fraction Products: Example 6TopicComparing Fractions DescriptionThis example focuses on comparing a fraction product to a whole number. The image depicts an area calculation problem. This concept involves understanding how to multiply fractions and compare the results. Skills required include fraction multiplication, simplification, and comparison. The problem can be solved using an algorithmic approach, where each fraction is multiplied and the products are compared, or through a visual model to illustrate the relative sizes of the products. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Comparing Fraction Products: Example 7 | Comparing Fraction Products: Example 7TopicComparing Fractions DescriptionThis example illustrates the concept of comparing a fraction product to a whole number. The image shows an improper fraction product. This involves understanding fraction multiplication and comparison. Skills involved include multiplying fractions, simplifying them, and comparing the outcomes. The problem is typically solved using an algorithmic approach, where fractions are multiplied and compared, or through a visual model that helps visualize the relative sizes of the products. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Comparing Fraction Products: Example 8 | Comparing Fraction Products: Example 8TopicComparing Fractions DescriptionThis example demonstrates the process of identifying a fraction product in a word problem. The image illustrates a scale model situation. This involves understanding fraction multiplication and comparison. Skills required include multiplying fractions, simplifying them, and comparing the results. The problem is solved using an algorithmic method, where fractions are multiplied and compared, or through a visual model that helps visualize the relative sizes of the products. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Comparing Fraction Products: Example 3 | Comparing Fraction Products: Example 3TopicComparing Fractions DescriptionThis example focuses on comparing the products of fractions. The image depicts a fraction product compared to a whole number. The concept involves multiplying fractions and comparing the results to see which is larger or smaller. Skills required include multiplying fractions, simplifying them, and comparing the numerical outcomes. This problem can be solved using a straightforward algorithm where each fraction is multiplied, and the products are compared, or through a visual model that helps illustrate the relative sizes and relationships between the products. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Comparing Fraction Products: Example 5 | Comparing Fraction Products: Example 5TopicComparing Fractions DescriptionThis example demonstrates how to compare the products of fractions. The image shows a fraction product compared to a whole number. The concept requires understanding fraction multiplication and comparison. Skills involved include multiplying fractions, simplifying them, and comparing the results. The problem can be solved using an algorithmic method, where fractions are multiplied and compared, or through a visual model that illustrates the relative sizes of the products. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Comparing Fraction Products: Example 4 | Comparing Fraction Products: Example 4TopicComparing Fractions DescriptionThis example highlights the process of comparing products of fractions. The image illustrates a fraction product compared to a whole number, with the resulting products compared to see which is larger. This concept involves understanding the multiplication of fractions and the comparison of their products. Skills required include multiplying fractions, simplifying them, and comparing the outcomes. The problem is solved using an algorithmic approach, where each fraction is multiplied and the products are compared, or through a visual model that helps visualize the relative sizes of the products. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Comparing Fraction Products: Example 2 | Comparing Fraction Products: Example 2TopicComparing Fractions DescriptionThis example illustrates the process of comparing the products of fractions. The image shows a scenario where two fractions are multiplied and their products are compared to determine which is greater. The mathematical concept involves understanding how to multiply fractions and compare the resulting products. Key skills include fraction multiplication, simplification, and comparison. Solving this problem often requires an algorithmic method where fractions are multiplied and then compared, or a visual model might be employed to provide a clearer understanding of the size of each product relative to the other. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Comparing Fraction Products: Example 1 | Comparing Fraction Products: Example 1TopicComparing Fractions |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Comparing Fraction Products: Example 9 | Comparing Fraction Products: Example 9TopicComparing Fractions DescriptionThis example focuses on generating a fraction product in a word problem situation. This concept involves understanding how to multiply fractions and compare the results. Skills required include fraction multiplication, simplification, and comparison. The problem can be solved using an algorithmic approach, where each fraction is multiplied and the products are compared, or through a visual model to illustrate the relative sizes of the products. |
Fractions and Mixed Numbers |

|
Math Example--Fraction Properties--Decomposing Fractions: Example 1 | Decomposing Fractions: Example 1TopicFraction Properties DescriptionThis example demonstrates the concept of decomposing fractions, which involves breaking down a fraction into a sum of fractions with the same denominator. The visual representation helps in understanding how a single fraction can be expressed as a combination of smaller fractions. Skills involved include recognizing equivalent fractions, understanding fraction addition, and applying decomposition techniques, which are essential for mastering more complex fraction operations. |
Add and Subtract Fractions |

|
Math Example--Fraction Properties--Decomposing Fractions: Example 2 | Decomposing Fractions: Example 2TopicFraction Properties DescriptionThis example illustrates decomposing fractions by breaking a fraction into a sum of smaller fractions with the same denominator. The image aids in visualizing how complex fractions can be simplified into simpler components. Key skills include understanding fraction equivalence, performing fraction addition, and applying decomposition strategies, which are foundational for more advanced fraction concepts. |
Add and Subtract Fractions |

|
Math Example--Fraction Properties--Decomposing Fractions: Example 7 | Decomposing Fractions: Example 7TopicFraction Properties DescriptionThis example focuses on decomposing fractions, showing how a fraction can be divided into a sum of fractions with the same denominator. The visual representation helps in understanding the breakdown of complex fractions into simpler parts. Essential skills include recognizing equivalent fractions, performing fraction addition, and applying decomposition techniques, which are crucial for mastering advanced fraction operations. |
Add and Subtract Fractions |