Illustrative Math Alignment: Grade 7 Unit 2

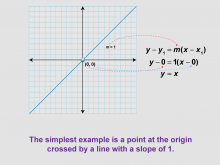
Topic

Linear Functions

Description

This image presents a graph crossing the origin and the corresponding point-slope form. It provides the simplest example of using the point-slope form, where a line passes through the origin (0,0) with a slope of 1.

By using this visual aid, teachers can help students understand the point-slope form in its most basic application. This image is particularly useful for building confidence before moving on to more complex examples.

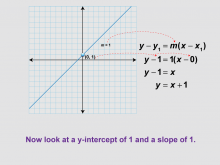
Topic

Linear Functions

Description

This image presents a variation of the previous example, now with the coordinate (0, 1) and a slope of 1. It demonstrates how to use the point-slope form when the y-intercept is not at the origin.

By incorporating this visual aid, teachers can help students understand how the point-slope form can be used with different y-intercepts. This image is particularly useful for showing how the form adapts to different scenarios while maintaining its basic structure.

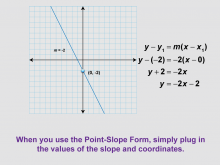
Topic

Linear Functions

Description

This image shows a variation of the previous example, now with a line having a negative slope of -2 and crossing the y-axis at (0, -2). It demonstrates how to use the point-slope form with negative slopes and y-intercepts.

By using this visual aid, teachers can help students understand how the point-slope form accommodates negative values. This image is particularly useful for expanding students' understanding of the form's versatility.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = 7x + 1, where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = 9x + (-2), where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = 6x + (-10), where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = -x + (-8), where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = -x + (-3), where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = -6x + (-3), where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = -3x + 1, where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = -10x + (-3), where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = 7x + (-9), where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates how to evaluate a linear function at a specific value. The function in this example is the function f(x) = 7x + (-3), where x is replaced with a given value to determine the corresponding f(x) value. The process includes substitution and arithmetic simplification.

Topic

Linear Functions

Description

This example demonstrates the process of converting a linear equation from standard form to slope-intercept form. The equation 2x + 4y = 8 is solved step-by-step, isolating y and dividing by its coefficient. The result is y = -1/2 x + 2, clearly showing the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example illustrates the conversion of the linear equation x + y = 1 from standard form to slope-intercept form. The process involves isolating y, resulting in y = -x + 1. This simple transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example showcases the transformation of the linear equation x + y = -1 from standard form to slope-intercept form. The process involves isolating y, resulting in y = -x - 1. This step-by-step solution clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example demonstrates the conversion of the linear equation x - y = 1 from standard form to slope-intercept form. The solution process involves isolating y and changing the sign of both sides, resulting in y = x - 1. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example illustrates the process of converting the linear equation -x + y = 1 from standard form to slope-intercept form. The solution involves rearranging the equation to isolate y, resulting in y = x + 1. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example demonstrates the conversion of the linear equation -x - y = -1 from standard form to slope-intercept form. The process involves manipulating the equation to solve for y, yielding y = -x + 1. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example showcases the transformation of the linear equation -x - y = 1 from standard form to slope-intercept form. The solution process involves isolating y, resulting in y = -x - 1. This step-by-step conversion clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example demonstrates the conversion of the linear equation -x + y = -1 from standard form to slope-intercept form. The process involves isolating y, resulting in y = x - 1. This transformation clearly reveals the slope and y-intercept of the line.

Linear functions are fundamental mathematical concepts that describe relationships between two variables. The examples in this collection, such as showing step-by-step transformations from standard form to slope-intercept form, help in understanding how each part of the equation affects the graph and the relationship itself.

Topic

Linear Functions

Description

This example illustrates the conversion of the linear equation x - y = -1 from standard form to slope-intercept form. The solution involves isolating y, resulting in y = x + 1. This process clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example demonstrates the conversion of the linear equation -x - y = 1 from standard form to slope-intercept form. The process involves rearranging the equation to isolate y, resulting in y = -x - 1. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example illustrates the conversion of the linear equation 12x + 28y = 0 from standard form to slope-intercept form. The solution involves isolating y and dividing by its coefficient, resulting in y = -3/7 x. This process clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example illustrates the conversion of the linear equation 3x + 6y = -18 from standard form to slope-intercept form. The solution involves isolating y and dividing by its coefficient, resulting in y = -1/2 x - 3. This process clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example demonstrates the conversion of the linear equation -14x - 35y = 0 from standard form to slope-intercept form. The process involves isolating y and dividing by its coefficient, resulting in y = -2/5 x. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example showcases the transformation of the linear equation 28x - 21y = 0 from standard form to slope-intercept form. The process involves isolating y and dividing by its coefficient, resulting in y = 4/3 x. This step-by-step solution clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example illustrates the conversion of the linear equation -13x + 39y = 0 from standard form to slope-intercept form. The solution involves isolating y and dividing by its coefficient, resulting in y = 1/3 x. This process clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example showcases the transformation of the linear equation 5x - 2y = 12 from standard form to slope-intercept form. The process involves isolating y and dividing by its coefficient, resulting in y = 2.5x - 6. This step-by-step solution clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example demonstrates the conversion of the linear equation -6x + 10y = 20 from standard form to slope-intercept form. The solution process involves isolating y and dividing by its coefficient, resulting in y = 0.6x + 2. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example illustrates the process of converting the linear equation -9x - 15y = -45 from standard form to slope-intercept form. The solution involves rearranging the equation to isolate y, resulting in y = 3/5 x + 3. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example demonstrates the conversion of the linear equation -8x - 12y = 25 from standard form to slope-intercept form. The process involves manipulating the equation to solve for y, yielding y = -2/3 x - 25/12. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example illustrates the transformation of the linear equation -2x + 16y = -36 from standard form to slope-intercept form. The solution process involves solving for y, resulting in y = 1/8 x - 9/4. This step-by-step conversion clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example showcases the conversion of the linear equation -7x + 21y = 63 from standard form to slope-intercept form. The process involves rearranging the equation to isolate y, resulting in y = 1/3 x + 3. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

This example demonstrates the process of converting the linear equation 10x - 28y = 56 from standard form to slope-intercept form. The solution involves isolating y and dividing by its coefficient, resulting in y = (5/14)x - 2. This transformation clearly reveals the slope and y-intercept of the line.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using a function machine with the rule f(x) = 2x. This involves creating a table of values for x and f(x) using f(x) = 2x. The output for each x is calculated by multiplying x by 2. The results are f(x) = -4, -2, 0, 2, and 4 for x = -2, -1, 0, 1, and 2, respectively.

Linear Functions are a key concept in mathematics that involves understanding the relationship between input and output values based on a given rule. Examples like this one help students visualize and analyze patterns, making it easier to comprehend linear relationships.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using a function machine with the rule f(x) = 3x + 1. This involves creating a table of values for x and f(x) using f(x) = 3x + 1. The output for each x is calculated by tripling x and then adding 1. The results are f(x) = -5, -2, 1, 4, and 7 for x = -2, -1, 0, 1, and 2, respectively.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using the function machine represented by the equation f(x) = 4 * x + 1. This involves a table created for x and f(x) values. By substituting each x value into f(x) = 4 * x + 1, the outputs are calculated as follows: -2 gives -7, -1 gives -3, 0 gives 1, 1 gives 5, and 2 gives 9. The common difference between successive outputs is +4, confirming a linear function.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using the function machine represented by the equation f(x) = 5 * x + 1. This involves a table created for x and f(x) values. substituting each x into f(x) = 5 * x + 1, the outputs are calculated: -2 gives -9, -1 gives -4, 0 gives 1, 1 gives 6, and 2 gives 11. the common difference between outputs is +5, confirming a linear function.

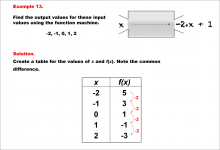
Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using the function machine represented by the equation f(x) = -2 * x + 1. This involves a table created for x and f(x) values. Substituting each x into f(x) = -2 * x + 1, the outputs are: -2 gives 5, -1 gives 3, 0 gives 1, 1 gives -1, and 2 gives -3. The common difference is -2, indicating a linear function.

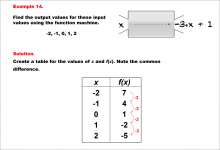
Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using the function machine represented by the equation f(x) = -3 * x + 1. This involves a table created for x and f(x) values. Each x substituted into f(x) = -3 * x + 1 yields: -2 gives 7, -1 gives 4, 0 gives 1, 1 gives -2, and 2 gives -5. The common difference is -3, confirming linearity.

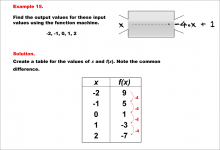
Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using the function machine represented by the equation f(x) = -4 * x + 1. This involves a table is created for x and f(x) values. Calculating for each x: -2 gives 9, -1 gives 5, 0 gives 1, 1 gives -3, and 2 gives -7. The common difference is -4, indicating linearity.

Linear Functions are a key concept in mathematics that involves understanding the relationship between input and output values based on a given rule. Examples like this one help students visualize and analyze patterns, making it easier to comprehend linear relationships.

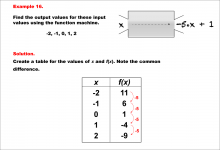
Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using the function machine represented by the equation f(x) = -5 * x + 1. This involves a table created for x and f(x) values. Substituting into f(x) = -5 * x + 1, the outputs are: -2 gives 11, -1 gives 6, 0 gives 1, 1 gives -4, and 2 gives -9. The common difference is -5, confirming linearity.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using the function machine represented by the constant function f(x) = 2. This involves a table created for x and f(x) values, where f(x) = 2 for all x values, resulting in outputs of 2, 2, 2, 2, and 2. The common difference is 0, indicating a constant function: y = 2.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using the function machine represented by the constant function f(x) = 0. This involves a table created for x and f(x) values, where f(x) = 0 for all x values, giving outputs of 0, 0, 0, 0, and 0. The common difference is 0, indicating a constant function: y = 0.

Linear Functions are a key concept in mathematics that involves understanding the relationship between input and output values based on a given rule. Examples like this one help students visualize and analyze patterns, making it easier to comprehend linear relationships.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using the function machine represented by the constant function f(x) = -1. This involves a table created for x and f(x) values, where f(x) = -1 for all x values, resulting in outputs of -1, -1, -1, -1, and -1. The common difference is 0, indicating a constant function: y = -1.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using a function machine with the rule f(x) = 3x. This involves creating a table of values for x and f(x) using f(x) = 3x. The output for each x is calculated by multiplying x by 3. the results are f(x) = -6, -3, 0, 3, and 6 for x = -2, -1, 0, 1, and 2, respectively.

Linear Functions are a key concept in mathematics that involves understanding the relationship between input and output values based on a given rule. Examples like this one help students visualize and analyze patterns, making it easier to comprehend linear relationships.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using a function machine with the rule f(x) = 4x. This involves creating a table of values for x and f(x) using f(x) = 4x. The output for each x is calculated by multiplying x by 4. The results are f(x) = -8, -4, 0, 4, and 8 for x = -2, -1, 0, 1, and 2, respectively.

Linear Functions are a key concept in mathematics that involves understanding the relationship between input and output values based on a given rule. Examples like this one help students visualize and analyze patterns, making it easier to comprehend linear relationships.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using a function machine with the rule f(x) = 5x. This involves creating a table of values for x and f(x) using f(x) = 5x. The output for each x is calculated by multiplying x by 5. The results are f(x) = -10, -5, 0, 5, and 10 for x = -2, -1, 0, 1, and 2, respectively.

Linear Functions are a key concept in mathematics that involves understanding the relationship between input and output values based on a given rule. Examples like this one help students visualize and analyze patterns, making it easier to comprehend linear relationships.

Topic

Linear Functions

Description

Find the output values for the input values -2, -1, 0, 1, 2 using a function machine with the rule f(x) = -2x. This involves creating a table of values for x and f(x) using f(x) = -2x. The output for each x is calculated by multiplying x by -2. The results are f(x) = 4, 2, 0, -2, and -4 for x = -2, -1, 0, 1, and 2, respectively.