Illustrative Math Alignment: Grade 7 Unit 3

Measuring Circles

Lesson 2: Exploring Circles

Use the following Media4Math resources with this Illustrative Math lesson.

Title	Body	Curriculum Nodes
Math Video Collection: Geometry Applications Video Series: Circles	Overview This collection aggregates all the math videos and resources in this series: Geometry Applications Video Series: Circles. There are a total of 13 resources. This collection of resources is made up of downloadable MP4, transcripts, and other resources files that you can easily incorporate into a presentation.	Applications of Circles and Definition of a Circle
Math Video Collection: Geometry Applications Video Series: Circles	Overview This collection aggregates all the math videos and resources in this series: Geometry Applications Video Series: Circles. There are a total of 13 resources. This collection of resources is made up of downloadable MP4, transcripts, and other resources files that you can easily incorporate into a presentation.	Applications of Circles and Definition of a Circle
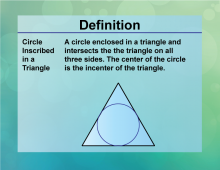
Definition--Circle Concepts--Circle Inscribed in a Triangle	Circle Inscribed in a Triangle Topic Circles Definition An inscribed circle in a triangle is tangent to each of the triangle's sides.	Definition of a Circle
Definition--Circle Concepts--Circle Inscribed in a Triangle	Circle Inscribed in a Triangle Topic Circles Definition An inscribed circle in a triangle is tangent to each of the triangle's sides.	Definition of a Circle
Definition--Circle Concepts--Circle Inscribed in a Triangle	Circle Inscribed in a Triangle Topic Circles Definition An inscribed circle in a triangle is tangent to each of the triangle's sides.	Definition of a Circle
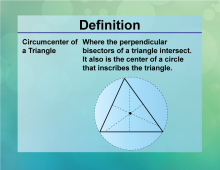
Definition--Circle Concepts--Circumcenter of Triangle	Circumcenter of Triangle Topic Circles Definition The circumcenter of a triangle is the point where the perpendicular bisectors of the sides intersect, equidistant from the vertices.	Definition of a Circle
Definition--Circle Concepts--Circumcenter of Triangle	Circumcenter of Triangle Topic Circles Definition The circumcenter of a triangle is the point where the perpendicular bisectors of the sides intersect, equidistant from the vertices.	Definition of a Circle
Definition--Circle Concepts--Circumcenter of Triangle	Circumcenter of Triangle Topic Circles Definition The circumcenter of a triangle is the point where the perpendicular bisectors of the sides intersect, equidistant from the vertices.	Definition of a Circle
Definition--Circle Concepts--Incenter of a Triangle	Incenter of a Triangle Topic Circles Definition The incenter of a triangle is the point where the angle bisectors intersect, equidistant from the sides.	Definition of a Circle
Definition--Circle Concepts--Incenter of a Triangle	Incenter of a Triangle Topic Circles Definition The incenter of a triangle is the point where the angle bisectors intersect, equidistant from the sides.	Definition of a Circle
Definition--Circle Concepts--Incenter of a Triangle	Incenter of a Triangle Topic Circles Definition The incenter of a triangle is the point where the angle bisectors intersect, equidistant from the sides.	Definition of a Circle
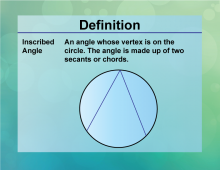
Definition--Circle Concepts--Inscribed Angle	Inscribed Angle Topic Circles Definition An inscribed angle is an angle formed by two chords in a circle that share an endpoint.	Definition of a Circle
Definition--Circle Concepts--Inscribed Angle	Inscribed Angle Topic Circles Definition An inscribed angle is an angle formed by two chords in a circle that share an endpoint.	Definition of a Circle
Definition--Circle Concepts--Inscribed Angle	Inscribed Angle Topic Circles Definition An inscribed angle is an angle formed by two chords in a circle that share an endpoint.	Definition of a Circle
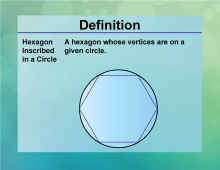
Definition--Circle Concepts--Hexagon Inscribed in a Circle	Hexagon Inscribed in a Circle Topic Circles Definition A hexagon inscribed in a circle is a six-sided polygon where each vertex touches the circle.	Definition of a Circle
Definition--Circle Concepts--Hexagon Inscribed in a Circle	Hexagon Inscribed in a Circle Topic Circles Definition A hexagon inscribed in a circle is a six-sided polygon where each vertex touches the circle.	Definition of a Circle
Definition--Circle Concepts--Hexagon Inscribed in a Circle	Hexagon Inscribed in a Circle Topic Circles Definition A hexagon inscribed in a circle is a six-sided polygon where each vertex touches the circle.	Definition of a Circle
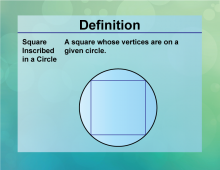
Definition--Circle Concepts--Square Inscribed in a Circle	Square Inscribed in a Circle Topic Circles Definition A square inscribed in a circle has its vertices touching the circle.	Definition of a Circle
Definition--Circle Concepts--Square Inscribed in a Circle	Square Inscribed in a Circle Topic Circles Definition A square inscribed in a circle has its vertices touching the circle.	Definition of a Circle
Definition--Circle Concepts--Square Inscribed in a Circle	Square Inscribed in a Circle Topic Circles Definition A square inscribed in a circle has its vertices touching the circle.	Definition of a Circle
Definition--Circle Concepts--Triangle Inscribed in a Circle	Triangle Inscribed in a Circle Topic Circles Definition A triangle inscribed in a circle has its vertices on the circle.	Definition of a Circle
Definition--Circle Concepts--Triangle Inscribed in a Circle	Triangle Inscribed in a Circle Topic Circles Definition A triangle inscribed in a circle has its vertices on the circle.	Definition of a Circle
Definition--Circle Concepts--Triangle Inscribed in a Circle	Triangle Inscribed in a Circle Topic Circles Definition A triangle inscribed in a circle has its vertices on the circle.	Definition of a Circle
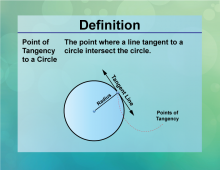
Definition--Circle Concepts--Point of Tangency to a Circle	Point of Tangency to a Circle Topic Circles Definition The point of tangency is the point where a tangent line touches the circle.	Definition of a Circle
Definition--Circle Concepts--Point of Tangency to a Circle	Point of Tangency to a Circle Topic Circles Definition The point of tangency is the point where a tangent line touches the circle.	Definition of a Circle
Definition--Circle Concepts--Point of Tangency to a Circle	Point of Tangency to a Circle Topic Circles Definition The point of tangency is the point where a tangent line touches the circle.	Definition of a Circle
Definition--Circle Concepts--Concentric Circles	Concentric Circles Topic Circles Definition Concentric circles are circles that share the same center but have different radii.	Definition of a Circle
Definition--Circle Concepts--Concentric Circles	Concentric Circles Topic Circles Definition Concentric circles are circles that share the same center but have different radii.	Definition of a Circle
Definition--Circle Concepts--Concentric Circles	Concentric Circles Topic Circles Definition Concentric circles are circles that share the same center but have different radii.	Definition of a Circle
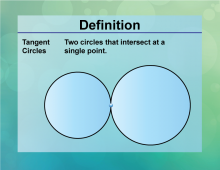
Definition--Circle Concepts--Tangent Circles	Tangent Circles Topic Circles Definition Tangent circles are two or more circles that intersect at exactly one point.	Definition of a Circle
Definition--Circle Concepts--Tangent Circles	Tangent Circles Topic Circles Definition Tangent circles are two or more circles that intersect at exactly one point.	Definition of a Circle
Definition--Circle Concepts--Tangent Circles	Tangent Circles Topic Circles Definition Tangent circles are two or more circles that intersect at exactly one point.	Definition of a Circle
Definition--Circle Concepts--Tangent Circles	Tangent Circles Topic Circles Definition Tangent circles are two or more circles that intersect at exactly one point.	Definition of a Circle
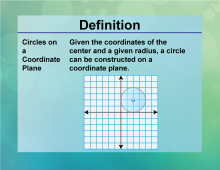
Definition--Circle Concepts--Circles on a Coordinate Plane	Circles on a Coordinate Plane Topic Circles Definition Circles on a coordinate plane are defined by their center coordinates and radius. Description Understanding circles on a coordinate plane is essential for analyzing geometric shapes in a mathematical context. This concept is widely used in computer graphics, navigation systems, and physics simulations, where precise positioning and movement of circular objects are required. The standard equation of a circle in the coordinate plane is (x − h)2 + (y − k)2 = r2	Definition of a Circle
Definition--Circle Concepts--Circles on a Coordinate Plane	Circles on a Coordinate Plane Topic Circles Definition Circles on a coordinate plane are defined by their center coordinates and radius. Description Understanding circles on a coordinate plane is essential for analyzing geometric shapes in a mathematical context. This concept is widely used in computer graphics, navigation systems, and physics simulations, where precise positioning and movement of circular objects are required. The standard equation of a circle in the coordinate plane is (x − h)2 + (y − k)2 = r2	Definition of a Circle
Definition--Circle Concepts--Circles on a Coordinate Plane	Circles on a Coordinate Plane Topic Circles Definition Circles on a coordinate plane are defined by their center coordinates and radius. Description Understanding circles on a coordinate plane is essential for analyzing geometric shapes in a mathematical context. This concept is widely used in computer graphics, navigation systems, and physics simulations, where precise positioning and movement of circular objects are required. The standard equation of a circle in the coordinate plane is (x − h)2 + (y − k)2 = r2	Definition of a Circle
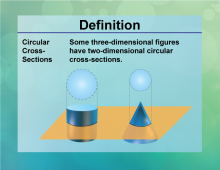
Definition--Circle Concepts--Circular Cross-Sections	Circular Cross-Sections Topic Circles Definition Circular cross-sections are the intersections of a plane with a solid that result in a circle.	Definition of a Circle
Definition--Circle Concepts--Circular Cross-Sections	Circular Cross-Sections Topic Circles Definition Circular cross-sections are the intersections of a plane with a solid that result in a circle.	Definition of a Circle
Definition--Circle Concepts--Circular Cross-Sections	Circular Cross-Sections Topic Circles Definition Circular cross-sections are the intersections of a plane with a solid that result in a circle.	Definition of a Circle
Definition--Circle Concepts--Equation of a Circles	Equation of a Circle Topic Circles Definition The equation of a circle in a plane is (x − h)2 + (y − k)2 = r2, where (h , k) is the center and r is the radius.	Definition of a Circle
Definition--Circle Concepts--Equation of a Circles	Equation of a Circle Topic Circles Definition The equation of a circle in a plane is (x − h)2 + (y − k)2 = r2, where (h , k) is the center and r is the radius.	Definition of a Circle
Definition--Circle Concepts--Equation of a Circles	Equation of a Circle Topic Circles Definition The equation of a circle in a plane is (x − h)2 + (y − k)2 = r2, where (h , k) is the center and r is the radius.	Definition of a Circle
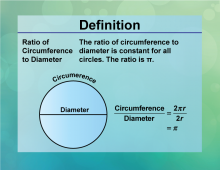
Definition--Circle Concepts--Ratio of Circumference to Diameter	Ratio of Circumference to Diameter Topic Circles Definition The ratio of the circumference to the diameter of a circle is the constant π.	Definition of a Circle
Definition--Circle Concepts--Ratio of Circumference to Diameter	Ratio of Circumference to Diameter Topic Circles Definition The ratio of the circumference to the diameter of a circle is the constant π.	Definition of a Circle
Definition--Circle Concepts--Ratio of Circumference to Diameter	Ratio of Circumference to Diameter Topic Circles Definition The ratio of the circumference to the diameter of a circle is the constant π.	Definition of a Circle
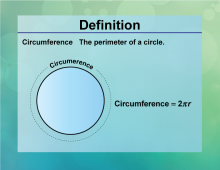
Definition--Circle Concepts--Circumference	Circumference Topic Circles Definition The circumference of a circle is the distance around the circle, calculated as C = 2πr. Description The circumference is a fundamental concept in geometry, representing the perimeter of a circle. It is widely used in fields such as engineering, design, and manufacturing, where precise measurements of circular objects are required. The formula C = 2πr	Definition of a Circle
Definition--Circle Concepts--Circumference	Circumference Topic Circles Definition The circumference of a circle is the distance around the circle, calculated as C = 2πr. Description The circumference is a fundamental concept in geometry, representing the perimeter of a circle. It is widely used in fields such as engineering, design, and manufacturing, where precise measurements of circular objects are required. The formula C = 2πr	Definition of a Circle
Definition--Circle Concepts--Circumference	Circumference Topic Circles Definition The circumference of a circle is the distance around the circle, calculated as C = 2πr. Description The circumference is a fundamental concept in geometry, representing the perimeter of a circle. It is widely used in fields such as engineering, design, and manufacturing, where precise measurements of circular objects are required. The formula C = 2πr	Definition of a Circle
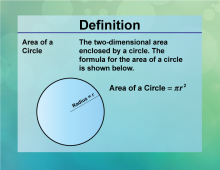
Definition--Circle Concepts--Area of a Circle	Area of a Circle Topic Circles Definition The area of a circle is the space contained within its circumference, calculated as A = πr2 Description The area of a circle is a fundamental concept in geometry, representing the space enclosed by the circle's boundary. This concept is widely applicable in fields such as physics, engineering, and design, where understanding the area is crucial for tasks like calculating material quantities or designing circular components. The formula A = πr2	Definition of a Circle
Definition--Circle Concepts--Area of a Circle	Area of a Circle Topic Circles Definition The area of a circle is the space contained within its circumference, calculated as A = πr2 Description The area of a circle is a fundamental concept in geometry, representing the space enclosed by the circle's boundary. This concept is widely applicable in fields such as physics, engineering, and design, where understanding the area is crucial for tasks like calculating material quantities or designing circular components. The formula A = πr2	Definition of a Circle