
Illustrative Math Alignment: Grade 7 Unit 8
Probability and Sampling
Lesson 2: Chance Experiments
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|

|
Definition--Statistics and Probability Concepts--Addition Rule of Probability | Addition Rule of ProbabilityTopicStatistics and Probability DefinitionThe Addition Rule of Probability is a key concept in statistics that helps in understanding the interaction between different events. DescriptionThe Addition Rule of Probability is crucial in the field of statistics because it allows us to make informed decisions based on the relationships between variables. For instance, in real-world applications, this concept is essential in various fields such as economics, social sciences, and health studies. Understanding how events affect each other can lead to better predictions and strategies. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Addition Rule of Probability | Addition Rule of ProbabilityTopicStatistics and Probability DefinitionThe Addition Rule of Probability is a key concept in statistics that helps in understanding the interaction between different events. DescriptionThe Addition Rule of Probability is crucial in the field of statistics because it allows us to make informed decisions based on the relationships between variables. For instance, in real-world applications, this concept is essential in various fields such as economics, social sciences, and health studies. Understanding how events affect each other can lead to better predictions and strategies. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Addition Rule of Probability | Addition Rule of ProbabilityTopicStatistics and Probability DefinitionThe Addition Rule of Probability is a key concept in statistics that helps in understanding the interaction between different events. DescriptionThe Addition Rule of Probability is crucial in the field of statistics because it allows us to make informed decisions based on the relationships between variables. For instance, in real-world applications, this concept is essential in various fields such as economics, social sciences, and health studies. Understanding how events affect each other can lead to better predictions and strategies. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Addition Rule of Probability | Addition Rule of ProbabilityTopicStatistics and Probability DefinitionThe Addition Rule of Probability is a key concept in statistics that helps in understanding the interaction between different events. DescriptionThe Addition Rule of Probability is crucial in the field of statistics because it allows us to make informed decisions based on the relationships between variables. For instance, in real-world applications, this concept is essential in various fields such as economics, social sciences, and health studies. Understanding how events affect each other can lead to better predictions and strategies. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Bivariate Data | Bivariate DataTopicStatistics and Probability DefinitionBivariate data involves the analysis of two variables to determine relationships between them. DescriptionBivariate data is essential in statistics as it allows for the exploration of relationships between two variables, such as height and weight. This analysis is used in various fields, including economics, biology, and social sciences, to understand correlations and causations. For example, a scatter plot can be used to visually represent bivariate data, helping to identify trends or patterns. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Bivariate Data | Bivariate DataTopicStatistics and Probability DefinitionBivariate data involves the analysis of two variables to determine relationships between them. DescriptionBivariate data is essential in statistics as it allows for the exploration of relationships between two variables, such as height and weight. This analysis is used in various fields, including economics, biology, and social sciences, to understand correlations and causations. For example, a scatter plot can be used to visually represent bivariate data, helping to identify trends or patterns. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Bivariate Data | Bivariate DataTopicStatistics and Probability DefinitionBivariate data involves the analysis of two variables to determine relationships between them. DescriptionBivariate data is essential in statistics as it allows for the exploration of relationships between two variables, such as height and weight. This analysis is used in various fields, including economics, biology, and social sciences, to understand correlations and causations. For example, a scatter plot can be used to visually represent bivariate data, helping to identify trends or patterns. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Bivariate Data | Bivariate DataTopicStatistics and Probability DefinitionBivariate data involves the analysis of two variables to determine relationships between them. DescriptionBivariate data is essential in statistics as it allows for the exploration of relationships between two variables, such as height and weight. This analysis is used in various fields, including economics, biology, and social sciences, to understand correlations and causations. For example, a scatter plot can be used to visually represent bivariate data, helping to identify trends or patterns. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Bivariate Data | Bivariate DataTopicStatistics and Probability DefinitionBivariate data involves the analysis of two variables to determine relationships between them. DescriptionBivariate data is essential in statistics as it allows for the exploration of relationships between two variables, such as height and weight. This analysis is used in various fields, including economics, biology, and social sciences, to understand correlations and causations. For example, a scatter plot can be used to visually represent bivariate data, helping to identify trends or patterns. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Bivariate Data | Bivariate DataTopicStatistics and Probability DefinitionBivariate data involves the analysis of two variables to determine relationships between them. DescriptionBivariate data is essential in statistics as it allows for the exploration of relationships between two variables, such as height and weight. This analysis is used in various fields, including economics, biology, and social sciences, to understand correlations and causations. For example, a scatter plot can be used to visually represent bivariate data, helping to identify trends or patterns. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Causation | CausationTopicStatistics and Probability DefinitionCausation refers to the relationship between two events where one event is affected by the other. DescriptionCausation is a fundamental concept in statistics that distinguishes between correlation and causation. Understanding causation is vital in fields like medicine and social sciences to establish cause-effect relationships. For example, clinical trials are designed to establish causation between treatments and outcomes. Grasping causation is important for students to critically evaluate research findings and understand the implications of statistical analyses. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Causation | CausationTopicStatistics and Probability DefinitionCausation refers to the relationship between two events where one event is affected by the other. DescriptionCausation is a fundamental concept in statistics that distinguishes between correlation and causation. Understanding causation is vital in fields like medicine and social sciences to establish cause-effect relationships. For example, clinical trials are designed to establish causation between treatments and outcomes. Grasping causation is important for students to critically evaluate research findings and understand the implications of statistical analyses. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Causation | CausationTopicStatistics and Probability DefinitionCausation refers to the relationship between two events where one event is affected by the other. DescriptionCausation is a fundamental concept in statistics that distinguishes between correlation and causation. Understanding causation is vital in fields like medicine and social sciences to establish cause-effect relationships. For example, clinical trials are designed to establish causation between treatments and outcomes. Grasping causation is important for students to critically evaluate research findings and understand the implications of statistical analyses. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Causation | CausationTopicStatistics and Probability DefinitionCausation refers to the relationship between two events where one event is affected by the other. DescriptionCausation is a fundamental concept in statistics that distinguishes between correlation and causation. Understanding causation is vital in fields like medicine and social sciences to establish cause-effect relationships. For example, clinical trials are designed to establish causation between treatments and outcomes. Grasping causation is important for students to critically evaluate research findings and understand the implications of statistical analyses. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Causation | CausationTopicStatistics and Probability DefinitionCausation refers to the relationship between two events where one event is affected by the other. DescriptionCausation is a fundamental concept in statistics that distinguishes between correlation and causation. Understanding causation is vital in fields like medicine and social sciences to establish cause-effect relationships. For example, clinical trials are designed to establish causation between treatments and outcomes. Grasping causation is important for students to critically evaluate research findings and understand the implications of statistical analyses. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Causation | CausationTopicStatistics and Probability DefinitionCausation refers to the relationship between two events where one event is affected by the other. DescriptionCausation is a fundamental concept in statistics that distinguishes between correlation and causation. Understanding causation is vital in fields like medicine and social sciences to establish cause-effect relationships. For example, clinical trials are designed to establish causation between treatments and outcomes. Grasping causation is important for students to critically evaluate research findings and understand the implications of statistical analyses. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Combination 1 | Combination 1TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Combination 1 | Combination 1TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Combination 1 | Combination 1TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Combination 1 | Combination 1TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Combination 1 | Combination 1TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Combination 1 | Combination 1TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Combination 2 | Combination 2TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Probability |

|
Definition--Statistics and Probability Concepts--Combination 2 | Combination 2TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Probability |

|
Definition--Statistics and Probability Concepts--Combination 2 | Combination 2TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Probability |

|
Definition--Statistics and Probability Concepts--Combination 2 | Combination 2TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Probability |

|
Definition--Statistics and Probability Concepts--Combination 2 | Combination 2TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Probability |

|
Definition--Statistics and Probability Concepts--Combination 2 | Combination 2TopicStatistics and Probability DefinitionA combination is a selection of items from a larger pool where order does not matter. DescriptionCombinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is
where n is the total number of items, and r is the number of items to choose. |
Probability |

|
Definition--Statistics and Probability Concepts--Compound Events | Compound EventsTopicStatistics and Probability DefinitionA compound event in probability is an event that consists of two or more simple events. DescriptionCompound events are important in probability as they allow for the calculation of the likelihood of multiple events occurring together, which is applicable in areas like risk assessment and decision-making. Understanding compound events helps students develop skills to analyze complex scenarios and calculate probabilities effectively. |
Probability |

|
Definition--Statistics and Probability Concepts--Compound Events | Compound EventsTopicStatistics and Probability DefinitionA compound event in probability is an event that consists of two or more simple events. DescriptionCompound events are important in probability as they allow for the calculation of the likelihood of multiple events occurring together, which is applicable in areas like risk assessment and decision-making. Understanding compound events helps students develop skills to analyze complex scenarios and calculate probabilities effectively. |
Probability |

|
Definition--Statistics and Probability Concepts--Compound Events | Compound EventsTopicStatistics and Probability DefinitionA compound event in probability is an event that consists of two or more simple events. DescriptionCompound events are important in probability as they allow for the calculation of the likelihood of multiple events occurring together, which is applicable in areas like risk assessment and decision-making. Understanding compound events helps students develop skills to analyze complex scenarios and calculate probabilities effectively. |
Probability |

|
Definition--Statistics and Probability Concepts--Compound Events | Compound EventsTopicStatistics and Probability DefinitionA compound event in probability is an event that consists of two or more simple events. DescriptionCompound events are important in probability as they allow for the calculation of the likelihood of multiple events occurring together, which is applicable in areas like risk assessment and decision-making. Understanding compound events helps students develop skills to analyze complex scenarios and calculate probabilities effectively. |
Probability |

|
Definition--Statistics and Probability Concepts--Compound Events | Compound EventsTopicStatistics and Probability DefinitionA compound event in probability is an event that consists of two or more simple events. DescriptionCompound events are important in probability as they allow for the calculation of the likelihood of multiple events occurring together, which is applicable in areas like risk assessment and decision-making. Understanding compound events helps students develop skills to analyze complex scenarios and calculate probabilities effectively. |
Probability |

|
Definition--Statistics and Probability Concepts--Compound Events | Compound EventsTopicStatistics and Probability DefinitionA compound event in probability is an event that consists of two or more simple events. DescriptionCompound events are important in probability as they allow for the calculation of the likelihood of multiple events occurring together, which is applicable in areas like risk assessment and decision-making. Understanding compound events helps students develop skills to analyze complex scenarios and calculate probabilities effectively. |
Probability |

|
Definition--Statistics and Probability Concepts--Conditional Relative Frequency | Conditional Relative FrequencyTopicStatistics and Probability DefinitionConditional relative frequency is the ratio of the frequency of a subset of data to the frequency of a condition. DescriptionConditional relative frequency is crucial in analyzing categorical data, helping to understand the distribution of data under specific conditions. For example, the frequency of students who pass a test given they have attended all classes is a conditional relative frequency. Understanding this concept helps students interpret data in surveys and experiments more accurately. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Conditional Relative Frequency | Conditional Relative FrequencyTopicStatistics and Probability DefinitionConditional relative frequency is the ratio of the frequency of a subset of data to the frequency of a condition. DescriptionConditional relative frequency is crucial in analyzing categorical data, helping to understand the distribution of data under specific conditions. For example, the frequency of students who pass a test given they have attended all classes is a conditional relative frequency. Understanding this concept helps students interpret data in surveys and experiments more accurately. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Conditional Relative Frequency | Conditional Relative FrequencyTopicStatistics and Probability DefinitionConditional relative frequency is the ratio of the frequency of a subset of data to the frequency of a condition. DescriptionConditional relative frequency is crucial in analyzing categorical data, helping to understand the distribution of data under specific conditions. For example, the frequency of students who pass a test given they have attended all classes is a conditional relative frequency. Understanding this concept helps students interpret data in surveys and experiments more accurately. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Conditional Relative Frequency | Conditional Relative FrequencyTopicStatistics and Probability DefinitionConditional relative frequency is the ratio of the frequency of a subset of data to the frequency of a condition. DescriptionConditional relative frequency is crucial in analyzing categorical data, helping to understand the distribution of data under specific conditions. For example, the frequency of students who pass a test given they have attended all classes is a conditional relative frequency. Understanding this concept helps students interpret data in surveys and experiments more accurately. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Conditional Relative Frequency | Conditional Relative FrequencyTopicStatistics and Probability DefinitionConditional relative frequency is the ratio of the frequency of a subset of data to the frequency of a condition. DescriptionConditional relative frequency is crucial in analyzing categorical data, helping to understand the distribution of data under specific conditions. For example, the frequency of students who pass a test given they have attended all classes is a conditional relative frequency. Understanding this concept helps students interpret data in surveys and experiments more accurately. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Conditional Relative Frequency | Conditional Relative FrequencyTopicStatistics and Probability DefinitionConditional relative frequency is the ratio of the frequency of a subset of data to the frequency of a condition. DescriptionConditional relative frequency is crucial in analyzing categorical data, helping to understand the distribution of data under specific conditions. For example, the frequency of students who pass a test given they have attended all classes is a conditional relative frequency. Understanding this concept helps students interpret data in surveys and experiments more accurately. |
Data Analysis |
|
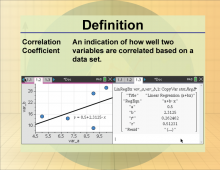
Definition--Statistics and Probability Concepts--Correlation Coefficient | Correlation CoefficientTopicStatistics and Probability DefinitionThe correlation coefficient is a measure that quantifies the degree to which two variables are related. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Correlation Coefficient | Correlation CoefficientTopicStatistics and Probability DefinitionThe correlation coefficient is a measure that quantifies the degree to which two variables are related. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Correlation Coefficient | Correlation CoefficientTopicStatistics and Probability DefinitionThe correlation coefficient is a measure that quantifies the degree to which two variables are related. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Correlation Coefficient | Correlation CoefficientTopicStatistics and Probability DefinitionThe correlation coefficient is a measure that quantifies the degree to which two variables are related. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Correlation Coefficient | Correlation CoefficientTopicStatistics and Probability DefinitionThe correlation coefficient is a measure that quantifies the degree to which two variables are related. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Correlation Coefficient | Correlation CoefficientTopicStatistics and Probability DefinitionThe correlation coefficient is a measure that quantifies the degree to which two variables are related. |
Data Analysis |

|
Definition--Statistics and Probability Concepts--Dependent Event | Dependent EventTopicStatistics and Probability DefinitionA dependent event is an event whose outcome is influenced by the outcome of a preceding event. |
Probability |

|
Definition--Statistics and Probability Concepts--Dependent Event | Dependent EventTopicStatistics and Probability DefinitionA dependent event is an event whose outcome is influenced by the outcome of a preceding event. |
Probability |

|
Definition--Statistics and Probability Concepts--Dependent Event | Dependent EventTopicStatistics and Probability DefinitionA dependent event is an event whose outcome is influenced by the outcome of a preceding event. |
Probability |

|
Definition--Statistics and Probability Concepts--Dependent Event | Dependent EventTopicStatistics and Probability DefinitionA dependent event is an event whose outcome is influenced by the outcome of a preceding event. |
Probability |