
Illustrative Math Alignment: Grade 8 Unit 7
Exponents and Scientific Notation
Lesson 7: Practice with Rational Bases
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|

|
Math Example--Exponential Concepts--Laws of Exponents: Example 22 | Math Example--Exponential Concepts--Laws of Exponents: Example 22TopicExponents DescriptionThis example demonstrates the simplification of an expression involving multiplication and division with both positive and negative exponents. The problem involves simplifying (x6 * x(-7)) / x(-9), which results in x8. This showcases how positive and negative exponents interact when multiplying and dividing terms with the same base, and how dividing by a negative exponent can lead to a positive result. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Laws of Exponents: Example 23 | Math Example--Exponential Concepts--Laws of Exponents: Example 23TopicExponents DescriptionThis example focuses on simplifying an expression involving multiplication and division with both positive and negative exponents. The problem involves simplifying (x(-5) * x9) / x(-6), which results in x10. This demonstrates how positive and negative exponents interact when multiplying and dividing terms with the same base, and how dividing by a negative exponent can lead to a larger positive exponent. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Laws of Exponents: Example 24 | Math Example--Exponential Concepts--Laws of Exponents: Example 24TopicExponents DescriptionThis example demonstrates the simplification of an expression involving multiplication and division with negative exponents. The problem involves simplifying (x(-2) * x(-6)) / x(-8), which results in 1. This showcases how negative exponents interact when multiplying and dividing terms with the same base, and how they can cancel out to yield a result of 1. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Laws of Exponents: Example 3 | Math Example--Exponential Concepts--Laws of Exponents: Example 3TopicExponents DescriptionThis example demonstrates the simplification of an expression resulting in a negative exponent. The problem involves simplifying x5 * x(-6), which results in x(-1) or 1/x. This showcases how positive and negative exponents interact and how the result can be expressed as a fraction. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Laws of Exponents: Example 4 | Math Example--Exponential Concepts--Laws of Exponents: Example 4TopicExponents DescriptionThis example focuses on simplifying an expression with two negative exponents, resulting in a fraction. The problem involves simplifying x(-10) * x(-7), which results in x(-17) or 1/x17. This demonstrates how negative exponents combine and how the result can be expressed as a fraction with a positive exponent. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Laws of Exponents: Example 5 | Math Example--Exponential Concepts--Laws of Exponents: Example 5TopicExponents DescriptionThis example demonstrates the simplification of an expression using exponents with multiple terms. The problem involves simplifying x4 * x6 * x8, which results in x18. This showcases how the exponents of terms with the same base can be added when multiplying. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Laws of Exponents: Example 6 | Math Example--Exponential Concepts--Laws of Exponents: Example 6TopicExponents DescriptionThis example focuses on simplifying an expression with both negative and positive exponents. The problem involves simplifying x(-2) * x7 * x11, which results in x16. This demonstrates how positive and negative exponents interact when multiplying terms with the same base, and how they can be combined to yield a single positive exponent. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Laws of Exponents: Example 7 | Math Example--Exponential Concepts--Laws of Exponents: Example 7TopicExponents DescriptionThis example demonstrates the simplification of an expression using both positive and negative exponents. The problem involves simplifying x8 * x(-5) * x6, which results in x9. This showcases how positive and negative exponents interact when multiplying terms with the same base, and how they can be combined to yield a single positive exponent. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Laws of Exponents: Example 8 | Math Example--Exponential Concepts--Laws of Exponents: Example 8TopicExponents DescriptionThis example focuses on simplifying an expression with negative exponents and converting it to a fraction. The problem involves simplifying x2 * x * x(-5), which results in 1/x2. This demonstrates how positive and negative exponents interact when multiplying terms with the same base, and how the result can be expressed as a fraction. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Laws of Exponents: Example 9 | Math Example--Exponential Concepts--Laws of Exponents: Example 9TopicExponents DescriptionThis example demonstrates the simplification of an expression with multiple negative exponents and one positive exponent. The problem involves simplifying x(-6) * x(-5) * x7, which results in x(-4) or 1/x4. This showcases how positive and negative exponents interact when multiplying terms with the same base, and how the result can be expressed as a fraction. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 1 | Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 1
This is part of a collection of math examples that focus on exponents, exponential expressions, and exponential functions. |
Numerical Expressions |

|
Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 2 | Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 2
This is part of a collection of math examples that focus on exponents, exponential expressions, and exponential functions. |
Numerical Expressions |

|
Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 3 | Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 3
This is part of a collection of math examples that focus on exponents, exponential expressions, and exponential functions. |
Numerical Expressions |

|
Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 4 | Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 4
This is part of a collection of math examples that focus on exponents, exponential expressions, and exponential functions. |
Numerical Expressions |

|
Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 5 | Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 5
This is part of a collection of math examples that focus on exponents, exponential expressions, and exponential functions. |
Numerical Expressions |

|
Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 6 | Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 6
This is part of a collection of math examples that focus on exponents, exponential expressions, and exponential functions. |
Numerical Expressions |

|
Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 7 | Math Example--Exponential Concepts--Modeling Sums with Integer Exponents--Example 7
This is part of a collection of math examples that focus on exponents, exponential expressions, and exponential functions. |
Numerical Expressions |

|
MATH EXAMPLES--Teacher's Guide: Laws of Exponents | MATH EXAMPLES--Teacher's Guide: Laws of Exponents
This Teacher's Guide provides an overview of the 24 worked-out examples on the laws of exponents. This is part of a collection of teacher's guides. To see the complete collection of teacher's guides, click on this link. Note: The download is a PDF file.Related ResourcesTo see resources related to this topic click on the Related Resources tab above. |
Laws of Exponents |

|
Paper-and-Pencil Quiz: Exponential Functions (Easy) | Paper-and-Pencil Quiz: Exponential Functions (Easy)
This is part of a collection of math quizzes on the topic of Exponential Functions. To see the complete quiz collection on this topic, click on this link. Note: The download is the PDF version of the quiz (with answer key).Related ResourcesTo see additional resources on this topic, click on the Related Resources tab.Quiz LibraryTo see the complete collection of Quizzes, click on this link.ary">click on this link. |
Exponential and Logarithmic Functions and Equations and Graphs of Exponential and Logarithmic Functions |

|
Paper-and-Pencil Quiz: Exponential Functions (Hard) | Paper-and-Pencil Quiz: Exponential Functions (Hard)
This is part of a collection of math quizzes on the topic of Exponential Functions. To see the complete quiz collection on this topic, click on this link. Note: The download is the PDF version of the quiz (with answer key).Related ResourcesTo see additional resources on this topic, click on the Related Resources tab.Quiz LibraryTo see the complete collection of Quizzes, click on this link.ary">click on this link. |
Exponential and Logarithmic Functions and Equations and Graphs of Exponential and Logarithmic Functions |

|
Paper-and-Pencil Quiz: Exponential Functions (Medium) | Paper-and-Pencil Quiz: Exponential Functions (Medium)
This is part of a collection of math quizzes on the topic of Exponential Functions. To see the complete quiz collection on this topic, click on this link. Note: The download is the PDF version of the quiz (with answer key).Related ResourcesTo see additional resources on this topic, click on the Related Resources tab.Quiz LibraryTo see the complete collection of Quizzes, click on this link.ary">click on this link. |
Exponential and Logarithmic Functions and Equations and Graphs of Exponential and Logarithmic Functions |

|
Paper-and-Pencil Quiz: Laws of Exponents (Easy) | Paper-and-Pencil Quiz: Laws of Exponents (Easy)
This is part of a collection of math quizzes on the topic of the Laws of Exponents. To see the complete quiz collection on this topic, click on this link. Note: The download is the PDF version of the quiz (with answer key).Related ResourcesTo see additional resources on this topic, click on the Related Resources tab.Quiz LibraryTo see the complete collection of Quizzes, click on this link.ary">click on this link. |
Laws of Exponents |

|
Paper-and-Pencil Quiz: Laws of Exponents (Hard) | Paper-and-Pencil Quiz: Laws of Exponents (Hard)
This is part of a collection of math quizzes on the topic of the Laws of Exponents. To see the complete quiz collection on this topic, click on this link. Note: The download is the PDF version of the quiz (with answer key).Related ResourcesTo see additional resources on this topic, click on the Related Resources tab.Quiz LibraryTo see the complete collection of Quizzes, click on this link.ary">click on this link. |
Laws of Exponents |

|
Paper-and-Pencil Quiz: Laws of Exponents (Medium) | Paper-and-Pencil Quiz: Laws of Exponents (Medium)
This is part of a collection of math quizzes on the topic of the Laws of Exponents. To see the complete quiz collection on this topic, click on this link. Note: The download is the PDF version of the quiz (with answer key).Related ResourcesTo see additional resources on this topic, click on the Related Resources tab.Quiz LibraryTo see the complete collection of Quizzes, click on this link.ary">click on this link. |
Laws of Exponents |

|
Video Transcript: Algebra Applications: Exponential Functions | Video Transcript: Algebra Applications: Exponential Functions
This is the transcript for the video of same title. Video contents: In this episode of Algebra Applications, students explore earthquakes using exponential models. In particular, students analyze the earthquake that struck the Sichuan Province in China in 2008, months before the Beijing Olympics. This dramatic, real-world example allows students to apply their understanding of exponential functions and their inverses, along with data analysis and periodic function analysis. |
Applications of Exponential and Logarithmic Functions and Graphs of Exponential and Logarithmic Functions |

|
Video Transcript: Algebra Applications: Exponential Functions, Segment 1: Introduction | Video Transcript: Algebra Applications: Exponential Functions, Segment 1: Introduction
This is the transcript for the video of same title. Video contents: In this introductory segment students learn about the great earthquake of 2008 that hit the Sichuan province of China. In the process they learn about how exponential functions provide a good model for describing earthquake intensity. |
Applications of Exponential and Logarithmic Functions and Graphs of Exponential and Logarithmic Functions |

|
Video Transcript: Algebra Applications: Exponential Functions, Segment 2: What Is an Earthquake? | Video Transcript: Algebra Applications: Exponential Functions, Segment 2: What Is an Earthquake?
This is the transcript for the video of same title. Video contents: The basic definition of an exponential function is shown in the intensity function for an earthquake. Students analyze data and perform an exponential regression based on data from the Sichuan earthquake. |
Applications of Exponential and Logarithmic Functions and Graphs of Exponential and Logarithmic Functions |

|
Video Transcript: Algebra Applications: Exponential Functions, Segment 3: What Is the Difference between Earthquake Intensity and Magnitude? | Video Transcript: Algebra Applications: Exponential Functions, Segment 3: What Is the Difference between Earthquake Intensity and Magnitude?
This is the transcript for the video of same title. Video contents: An exponential model describes the intensity of an earthquake, while a logarithmic model describes the magnitude of an earthquake. In the process students learn about the inverse of an exponential function. |
Applications of Exponential and Logarithmic Functions and Graphs of Exponential and Logarithmic Functions |

|
Video Transcript: Algebra Applications: Exponential Functions, Segment 4: How Is Earthquake Magnitude Measured? | Video Transcript: Algebra Applications: Exponential Functions, Segment 4: How Is Earthquake Magnitude Measured?
This is the transcript for the video of same title. Video contents: An earthquake is an example of a seismic wave. A wave can be modeled with a trigonometric function. Using the TI-Nspire, students link the amplitude to an exponential function to analyze the dramatic increase in intensity resulting from minor changes to magnitude. |
Applications of Exponential and Logarithmic Functions and Graphs of Exponential and Logarithmic Functions |

|
Video Transcript: Algebra Nspirations: Exponents | Video Transcript: Algebra Nspirations: Exponents and Exponential Functions
This is the transcript for the video of same title. Video contents: Almost everyone has an intuitive understanding that exponential growth means rapid growth. Written and hosted by internationally acclaimed math educator Dr. Monica Neagoy, this video builds on students' intuitive notions, explores exponential notation, and analyzes properties of exponential function graphs, with the help of TI-Nspire features such as sliders and graph transformations. Using exponential functions to model finance applications and a Newton??s law of cooling problem further help students build a solid foundation for these fundamental algebraic concepts. Concepts explored: functions, exponents, exponential functions |
Applications of Exponential and Logarithmic Functions and Graphs of Exponential and Logarithmic Functions |

|
Video Transcript: Algebra Nspirations: Exponents, 1 | Video Transcript: Algebra Nspirations: Exponents and Exponential Functions, Part 1
This is the transcript for the video of same title. Video contents: In this Investigation we explore the properties of exponents and exponential graphs. |
Applications of Exponential and Logarithmic Functions and Graphs of Exponential and Logarithmic Functions |

|
Video Transcript: Algebra Nspirations: Exponents, 2 | Video Transcript: Algebra Nspirations: Exponents and Exponential Functions, Part 2
This is the transcript for the video of same title. Video contents: In this Investigation we look at exponential growth and decay models. |
Applications of Exponential and Logarithmic Functions and Graphs of Exponential and Logarithmic Functions |

|
VIDEO: Algebra Nspirations: Exponents, 1 | VIDEO: Algebra Nspirations: Exponents, 1
TopicExponential Functions |
Applications of Exponential and Logarithmic Functions, Exponential and Logarithmic Functions and Equations and Graphs of Exponential and Logarithmic Functions |

|
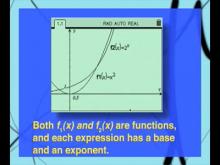
VIDEO: Algebra Nspirations: Exponents | VIDEO: Algebra Nspirations: Exponents
TopicExponential Functions |
Applications of Exponential and Logarithmic Functions, Exponential and Logarithmic Functions and Equations and Graphs of Exponential and Logarithmic Functions |
|
VIDEO: Algebra Nspirations: Exponents, 2 | VIDEO: Algebra Nspirations: Exponents, 2
TopicExponential Functions DescriptionIn this Math Lab explore a quadratic graph and an exponential graph and analyze their respective equations. This video is relevant to the topic of Exponential Functions as it provides a detailed exploration of key concepts and their applications. By presenting clear and practical examples, it helps students understand and apply mathematical principles effectively. |
Applications of Exponential and Logarithmic Functions, Exponential and Logarithmic Functions and Equations and Graphs of Exponential and Logarithmic Functions |

|
VIDEO: Algebra Nspirations: Exponents, 3 | VIDEO: Algebra Nspirations: Exponents, 3
TopicExponential Functions |
Applications of Exponential and Logarithmic Functions, Exponential and Logarithmic Functions and Equations and Graphs of Exponential and Logarithmic Functions |

|
VIDEO: Algebra Nspirations: Exponents, 4 | VIDEO: Algebra Nspirations: Exponents, 4
TopicExponential Functions DescriptionIn this Math Lab explore cooling curves expressed as exponential functions. This video is relevant to the topic of Exponential Functions as it provides a detailed exploration of key concepts and their applications. By presenting clear and practical examples, it helps students understand and apply mathematical principles effectively. |
Applications of Exponential and Logarithmic Functions, Exponential and Logarithmic Functions and Equations and Graphs of Exponential and Logarithmic Functions |

|
Worksheet: TI-Nspire Mini-Tutorial: Exploring Exponential Graphs with Sliders | Worksheet: TI-Nspire Mini-Tutorial: Exploring Exponential Graphs with Sliders
This is part of a collection of math worksheets on the use of the TI-Nspire graphing calculator. Each worksheet supports a companion TI-Nspire Mini-Tutorial video. It provides all the keystrokes for the activity. To see the complete worksheet collection on this topic, click on this link. Note: The download is a PDF file.Related ResourcesTo see additional resources on this topic, click on the Related Resources tab.Worksheet LibraryTo see the complete collection of Worksheets, click on this link. |
Graphs of Exponential and Logarithmic Functions |

|
Worksheet: TI-Nspire Mini-Tutorial: Exploring Logarithmic Graphs with Sliders | Worksheet: TI-Nspire Mini-Tutorial: Exploring Logarithmic Graphs with Sliders
This is part of a collection of math worksheets on the use of the TI-Nspire graphing calculator. Each worksheet supports a companion TI-Nspire Mini-Tutorial video. It provides all the keystrokes for the activity. To see the complete worksheet collection on this topic, click on this link. Note: The download is a PDF file.Related ResourcesTo see additional resources on this topic, click on the Related Resources tab.Worksheet LibraryTo see the complete collection of Worksheets, click on this link. |
Graphs of Exponential and Logarithmic Functions |