Illustrative Math Alignment: Grade 7 Unit 7

Topic

Quadratics

Description

Factoring is explained as a method to solve quadratics expressed as products of binomials. Terms like factor pairs and standard form are covered. Applications include determining dimensions of rectangles, triangles, and squares.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of video tutorials on the topic of Rational Numbers. This includes defining rational numbers, rational number operations, comparing and ordering rational numbers, and applications of rational numbers.

The following section includes background information on rational numbers. Refer to this section as you view the videos, or as review material afterward.

This is part of a collection of math definitions from Media4Math's Visual Glossary.

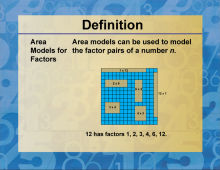
Topic

Factors and Multiples

Definition

Factors are numbers that divide another number without leaving a remainder, while multiples are the result of multiplying a number by an integer.

Description

Understanding factors and multiples is crucial in mathematics, particularly in number theory and algebra. Factors are the building blocks of numbers, representing the integers that can be multiplied together to produce another number. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12. Multiples, on the other hand, are the product of a number and any integer. For instance, the multiples of 5 include 5, 10, 15, 20, and so on.

Topic

Factors and Multiples

Definition

A common factor is a number that divides two or more numbers exactly without leaving a remainder.

Description

Common factors are integral to understanding the mathematical concepts of factors and multiples. They are the numbers that can divide two or more given numbers without leaving a remainder. For example, if you consider the numbers 12 and 18, their common factors are 1, 2, 3, and 6. This is because these numbers can divide both 12 and 18 exactly, with no remainder.

Topic

Factors and Multiples

Definition

A common multiple is a number that is a multiple of two or more numbers.

Description

In the context of factors and multiples, understanding common multiples is crucial for solving various mathematical problems, particularly those involving fractions, least common multiples (LCM), and algebraic expressions. A common multiple of a set of numbers is a number that each of the numbers in the set divides without leaving a remainder. For example, the common multiples of 4 and 6 include 12, 24, 36, and so on. The smallest of these, 12, is known as the least common multiple (LCM).

Topic

Factors and Multiples

Definition

A divisor is a number that divides another number evenly, leaving no remainder.

Description

In the context of factors and multiples, a divisor plays a crucial role in understanding the relationships between numbers. A divisor is essentially a factor of a given number, as it divides that number without leaving a remainder. This concept is fundamental to identifying factors and understanding divisibility rules.

Topic

Factors and Multiples

Definition

Factor pairs are sets of two numbers that, when multiplied together, produce a given number.

Description

Factor pairs play a crucial role in understanding the relationships between numbers and their factors. They are essential in various mathematical operations, particularly in algebra and number theory. Factor pairs help students visualize the multiplicative structure of numbers and provide a foundation for more advanced concepts such as prime factorization and divisibility rules.

Topic

Factors and Multiples

Definition

A factor tree is a graphical method used to break down a number into its prime factors.

Description

A factor tree is a visual representation that helps in identifying the prime factors of a given number. It starts with the original number at the top and branches downwards, breaking the number into its factors until all the branches end in prime numbers. This method is particularly useful in understanding the concept of prime factorization, which is the process of expressing a number as the product of its prime factors.

Topic

Factors and Multiples

Definition

Factoring algebraic expressions involves breaking down a polynomial into simpler components called factors that, when multiplied together, give the original polynomial.

Description

Factoring algebraic expressions is a fundamental concept in algebra that plays a crucial role in simplifying expressions, solving equations, and understanding polynomial functions. This process involves expressing a polynomial as a product of its factors, which are simpler polynomials or numbers. For instance, the polynomial x^2 - 5x + 6 can be factored into (x - 2)(x - 3).

Topic

Factors and Multiples

Definition

Factors are numbers that divide evenly into another number without leaving a remainder.

Description

Factors play a crucial role in understanding the fundamental properties of numbers and their relationships. In the context of factors and multiples, factors are the building blocks that, when multiplied together, create a given number. This concept is essential for various mathematical operations and problem-solving techniques.

Topic

Factors and Multiples

Definition

The Greatest Common Factor (GCF) is the largest number that divides two or more numbers without leaving a remainder.

Description

The concept of the Greatest Common Factor (GCF) is crucial in the study of factors and multiples. The GCF is used to simplify fractions, find common denominators, and solve problems involving ratios. Understanding the GCF helps in breaking down complex problems into simpler parts, making it easier to handle large numbers and perform arithmetic operations efficiently.

Topic

Factors and Multiples

Definition

The least common multiple (LCM) is the smallest number that is a multiple of two or more numbers.

Description

The concept of the least common multiple (LCM) is essential in the study of factors and multiples. The LCM of two or more numbers is the smallest number that is evenly divisible by all of the given numbers. This concept is particularly useful in various mathematical operations and problem-solving scenarios.

Topic

Factors and Multiples

Definition

A multiple is a number that can be divided by another number without leaving a remainder.

Description

Multiples are fundamental in understanding the relationships between numbers in mathematics. They are particularly relevant when learning about factors and multiples, which are key concepts in elementary and middle school math curricula. A multiple of a number is obtained by multiplying that number by an integer. For example, the multiples of 5 include 5, 10, 15, 20, and so on.

Topic

Factors and Multiples

Definition

Multiples of 10 are the numbers that result from multiplying 10 by any whole number.

Description

Multiples of 10 play a significant role in understanding factors and multiples. These numbers are easily recognizable as they always end in zero and form a predictable pattern on the number line. In the context of factors and multiples, multiples of 10 serve as an excellent example to illustrate the concept of multiples in general.

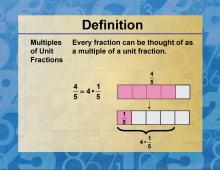
Topic

Factors and Multiples

Definition

Multiples are the results obtained when a number is multiplied by an integer.

Description

In mathematics, understanding the concept of multiples is crucial, especially when dealing with factors and multiples. A multiple of a number is the product obtained when that number is multiplied by an integer. For example, the multiples of 3 include 3, 6, 9, 12, and so on. This concept is fundamental in various mathematical operations and problem-solving scenarios.

Topic

Factors and Multiples

Definition

Prime factorization is the process of breaking down a composite number into its prime factors.

Description

Prime factorization is a fundamental concept in the study of factors and multiples. It involves expressing a composite number as a product of prime numbers. For example, the number 60 can be factorized into 2 × 2 × 3 × 5. This process is crucial because it provides a unique representation of numbers, which is essential in various mathematical applications, including simplifying fractions, finding least common multiples (LCM), and greatest common divisors (GCD).

Topic

Factors and Multiples

Definition

Prime factors are the prime numbers that multiply together to give the original number.

Description

Prime factors are a fundamental concept in the study of factors and multiples. They are the building blocks of all numbers, as any integer greater than 1 can be expressed as a product of prime numbers. This process is known as prime factorization. For example, the prime factorization of 28 is 2 × 2 × 7, where 2 and 7 are prime numbers.

Topic

Factors and Multiples

Definition

Proper factors are the factors of a number excluding the number itself and 1.

Description

Proper factors play a significant role in the study of factors and multiples. Understanding proper factors is essential for grasping more complex mathematical concepts such as prime factorization, greatest common divisors, and least common multiples.

Topic

Factors and Multiples

Definition

Simplifying fractions involves reducing the fraction to its simplest form by dividing both the numerator and the denominator by their greatest common factor (GCF).

Topic

Factors and Multiples

Definition

An unknown factor is a value in a multiplication equation that needs to be determined to solve the equation.

Description

Factors and multiples are fundamental concepts in mathematics, particularly in number theory and arithmetic. Understanding these concepts is crucial for solving a wide range of mathematical problems, from basic arithmetic to more advanced topics such as algebra and number theory.

Factors of a number are the integers that can be multiplied together to produce that number. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12. These numbers can be paired in various ways to produce the product 12 (e.g., 2 x 6, 3 x 4).

Topic

Factors and Multiples

Definition

The Least Common Multiple (LCM) of two or more numbers is used to find the Least Common Denominator (LCD) when working with fractions.

Topic

Factors and Multiples

Definition

Visual models of multiples are graphical representations that help illustrate the concept of multiples and common multiples of numbers, making it easier to understand their relationships and properties.

Topic

Fractions

Definition

The Greatest Common Factor (GCF) is the highest number that divides exactly into two or more numbers without leaving a remainder.

Description

The concept of the Greatest Common Factor (GCF) is crucial in the study of fractions. It is used to simplify fractions to their lowest terms, making them easier to work with and understand. When two or more fractions have the same GCF, it means they share a common factor that can be used to reduce each fraction to its simplest form. This process is essential for performing operations such as addition, subtraction, multiplication, and division of fractions.

Topic

Polynomials

Definition

A binomial factor is a two-term expression that, when multiplied by another factor, produces a given polynomial.

Description

Binomial factors are essential components in the study of polynomials, particularly in the context of factoring and solving polynomial equations. These two-term expressions play a crucial role in breaking down more complex polynomials into simpler, more manageable parts. Understanding binomial factors is key to mastering various algebraic techniques and problem-solving strategies.

Topic

Polynomials

Definition

The Factor Theorem states that a polynomial p(x) has a factor (x - r) if and only if p(r) = 0.

Description

The Factor Theorem is a powerful tool in polynomial algebra that bridges the concepts of roots and factors. This theorem provides a method for determining whether a given linear expression is a factor of a polynomial without performing long division. It's particularly useful in finding roots of polynomials and in polynomial factorization.

Topic

Polynomials

Definition

A factored cubic polynomial is a third-degree polynomial expressed as the product of its linear factors, typically in the form a(x - r₁)(x - r₂)(x - r₃), where r₁, r₂, and r₃ are the roots of the polynomial.

Topic

Polynomials

Definition

A factored polynomial is a polynomial that is expressed as the product of its factors.

Description

Factoring polynomials is a fundamental skill in algebra that simplifies complex expressions and solves polynomial equations. By expressing a polynomial as the product of its factors, students can more easily identify the roots of the polynomial and understand its behavior. Factored forms are particularly useful in solving quadratic and higher-degree equations.

Topic

Polynomials

Definition

A factored quadratic is a second-degree polynomial expressed as the product of its linear factors.

Description

Factoring quadratic polynomials is a key concept in algebra that simplifies solving quadratic equations and analyzing their properties. A factored quadratic reveals the roots of the equation, which are the x-intercepts of its graph. This form is particularly useful in solving equations, graphing quadratic functions, and understanding the behavior of parabolas.

Topic

Polynomials

Definition

A factorial, denoted by n!, is the product of all positive integers less than or equal to n.

Description

The factorial function is a fundamental concept in mathematics, particularly in combinatorics, algebra, and calculus. It is denoted by n! and represents the product of all positive integers up to a given number n. Factorials are used to calculate permutations, combinations, and in various mathematical series and functions.

Topic

Prime and Composite Numbers

Definition

Common factors are numbers that are factors of two or more numbers. In the context of prime and composite numbers, common factors can help identify relationships between different numbers.

Description

Common factors play a significant role in understanding the properties and relationships between prime and composite numbers. A prime number is defined as a number greater than 1 that has no positive divisors other than 1 and itself. In contrast, a composite number has more than two factors, meaning it can be divided evenly by numbers other than 1 and itself.

Topic

Prime and Composite Numbers

Definition

A factor is a number that divides another number without leaving a remainder.

Description

Factors play a crucial role in understanding prime and composite numbers. A prime number is defined as a number greater than 1 that has no positive divisors other than 1 and itself. This means a prime number has exactly two distinct factors: 1 and the number itself. For example, the number 7 is prime because its only factors are 1 and 7.

Topic

Prime and Composite Numbers

Definition

A factor tree is a graphical representation used to determine the prime factors of a composite number by breaking it down into its prime components.

Topic

Prime and Composite Numbers

Definition

The Greatest Common Factor (GCF) of two or more numbers is the largest number that divides all of them without leaving a remainder.

Description

The concept of the Greatest Common Factor (GCF) is crucial in the study of prime and composite numbers. The GCF helps in simplifying fractions, finding common denominators, and solving problems involving divisibility. Understanding the GCF is essential for grasping more complex mathematical concepts such as least common multiples, prime factorization, and algebraic expressions.

Topic

Prime and Composite Numbers

Definition

Prime factors are the prime numbers that when multiplied together give a particular result.

Description

Prime factors play a crucial role in the study of prime and composite numbers. A prime factor is a factor that is a prime number, one of the building blocks of all numbers. For example, the prime factors of 12 are 2 and 3, because 2 • 2 • 3 = 12. Understanding prime factors helps in various mathematical processes such as simplifying fractions, finding the greatest common divisor (GCD), and the least common multiple (LCM).

Topic

Prime and Composite Numbers

Definition

Prime factorization is the process of breaking down a composite number into a product of its prime factors.

Description

Prime factorization is a fundamental concept in number theory and is essential for understanding the properties of numbers. It involves expressing a composite number as a product of prime numbers, which are numbers greater than 1 that have no divisors other than 1 and themselves. For example, the prime factorization of 28 is 22 • 7, meaning 28 can be broken down into the prime numbers 2 and 7.

Topic

Prime and Composite Numbers

Definition

Proper factors of a number are all the factors of that number except the number itself and 1.

Description

Proper factors play a significant role in the study of prime and composite numbers. A prime number is defined as a number greater than 1 that has no proper factors other than itself and 1. This means that prime numbers cannot be divided evenly by any other numbers. For example, the number 7 is a prime number because its only factors are 1 and 7, and it has no proper factors.

Topic

Quadratics Concepts

Definition

A quadratic function in factored form is expressed as 

f(x) = a(x − r1)(x − r2)

where r1 ​ and r2 ​ are the roots.