Illustrative Math Alignment: Grade 8 Unit 3

Linear Relationships

Lesson 13: More Solutions to Linear Equations

Use the following Media4Math resources with this Illustrative Math lesson.

Title	Body	Curriculum Topic
INSTRUCTIONAL RESOURCE: Math Examples 26	INSTRUCTIONAL RESOURCE: Math Examples--Linear Equations in Standard Form This PowerPoint includes the 22 Tutorials on the topic of converting Linear Equations in Standard Form to Slope-Intercept Form. This is part of a collection of math examples for a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Applications of Linear Functions and Graphs of Linear Functions
INSTRUCTIONAL RESOURCE: Math Examples 30	INSTRUCTIONAL RESOURCE: Math Examples--Midpoint Formula The complete set of 20 examples that make up this set of tutorials. This is part of a collection of math examples for a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Midpoint Formula
INSTRUCTIONAL RESOURCE: Math Examples 36	INSTRUCTIONAL RESOURCE: Math Examples--Point-Slope Form The complete set of 8 examples that make up this set of tutorials. This is part of a collection of math examples for a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Applications of Linear Functions and Graphs of Linear Functions
INSTRUCTIONAL RESOURCE: Nspire App Tutorial: Point-Slope Form	In this Slide Show, create a template for determining the equation of a line given a point and the slope of the line. This presentation requires the use of the TI-Nspire iPad App. Note: the download is a PPT.	Applications of Linear Functions, Graphs of Linear Functions and Point-Slope Form
INSTRUCTIONAL RESOURCE: Tutorial: Solving Non-linear Systems	INSTRUCTIONAL RESOURCE: Tutorial: Solving Non-linear Systems This slide show defines non-linear systems, showing examples of linear and quadratic systems. This is part of a collection of tutorials on a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. < Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Solving Systems of Equations
INSTRUCTIONAL RESOURCE: Tutorial: Solving Systems: The Elimination Method	INSTRUCTIONAL RESOURCE: Tutorial: Solving Systems: The Elimination Method This slide show shows how to solve a system using the elimination method. This is part of a collection of tutorials on a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. < Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Solving Systems of Equations
INSTRUCTIONAL RESOURCE: Tutorial: Solving Systems: The Substitution Method	INSTRUCTIONAL RESOURCE: Tutorial: Solving Systems: The Substitution Method This slide show shows how to solve a system using the substitution method. This is part of a collection of tutorials on a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. < Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Solving Systems of Equations
INSTRUCTIONAL RESOURCE: Tutorial: The Point-Slope Form	<h1>INSTRUCTIONAL RESOURCE: Tutorial: The Point-Slope Form</h1><p>In this Slide Show, learn about thepoint-slope form. </p><h3> This is part of a collection of tutorials on a variety of math topics. To see the complete collection of these resources, <a href="https://www.media4math.com/instructional-resources?field_la_display_tit… on this link.</u></strong></a> </h3> <h3> Note: The download is a PPT file. </h3>< <h2> Library of Instructional Resources </h3>	Applications of Linear Functions, Graphs of Linear Functions and Point-Slope Form
INSTRUCTIONAL RESOURCE: Tutorial: Equations of Parallel and Perpendiclar Lines (SAT Prep)	INSTRUCTIONAL RESOURCE: Tutorial: Equations of Parallel and Perpendiclar Lines (SAT Prep) This slide show shows how to write equations in slope-intercept form for parallel and perpendicular lines. This slide show also includes some sample SAT-style questions. This is part of a collection of tutorials on a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Slope-Intercept Form
INSTRUCTIONAL RESOURCE: Tutorial: Point-Slope Form (SAT Prep)	INSTRUCTIONAL RESOURCE: Tutorial: Point-Slope Form (SAT Prep) This slide show shows how to use the point-slope form to write a linear equation in slope-intercept form. This slide show also includes some sample SAT-style questions. This is part of a collection of tutorials on a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Point-Slope Form
INSTRUCTIONAL RESOURCE: Tutorial: Solving a Linear System	INSTRUCTIONAL RESOURCE: Tutorial: Solving a Linear System In this Slide Show, learn how to solve a linear system by the elimination method. This is part of a collection of tutorials on a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Applications of Linear Systems and Solving Systems of Equations
INSTRUCTIONAL RESOURCE: Tutorial: What Is a System of Equations?	INSTRUCTIONAL RESOURCE: Tutorial: What Is a System of Equations? This slide show shows what a system of equations is. This is part of a collection of tutorials on a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. < Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Solving Systems of Equations
Interactive Crossword Puzzle--Slope Intercept Form	Interactive Crossword Puzzle--Slope Intercept Form This interactive crossword puzzle tests knowledge of key terms on the topic of the slope intercept form. This is part of a collection of math games and interactives. To see the complete collection of the games, click on this link. Note: The download is the teacher's guide. Related Resources To see additional resources on this topic, click on the Related Resources tab.	Slope-Intercept Form
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 1	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 1 Topic Linear Functions Description This example demonstrates how to graph a linear function with a slope of 2 and a y-intercept of 3. The process involves three key steps: first, plotting the y-intercept at (0, 3); second, using the slope to find another point on the line; and finally, connecting these points to form the line.	Slope-Intercept Form
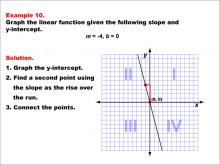
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 10	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 10 Topic Linear Functions Description This example illustrates the process of graphing a linear function with a slope of -4 and a y-intercept of 0. The method involves three main steps: plotting the y-intercept at the origin (0, 0), using the slope to determine a second point on the line, and connecting these points to create the linear graph.	Slope-Intercept Form
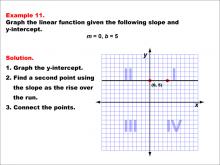
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 11	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 11 Topic Linear Functions Description This example demonstrates the process of graphing a linear function with a slope of 0 and a y-intercept of 5. The procedure involves three key steps: plotting the y-intercept at (0, 5), recognizing that a slope of 0 results in a horizontal line, and drawing the line parallel to the x-axis through the y-intercept.	Slope-Intercept Form
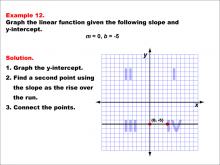
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 12	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 12 Topic Linear Functions Description This example illustrates the process of graphing a linear function with a slope of 0 and a y-intercept of -5. The method involves three main steps: plotting the y-intercept at (0, -5), recognizing that a slope of 0 results in a horizontal line, and drawing the line parallel to the x-axis through the y-intercept.	Slope-Intercept Form
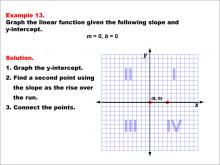
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 13	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 13 Topic Linear Functions Description This example demonstrates how to graph a linear function with a slope of 0 and a y-intercept of 0. The process involves recognizing that this special case results in a horizontal line coinciding with the x-axis. The line passes through the origin (0, 0) and extends infinitely in both directions along the x-axis.	Slope-Intercept Form
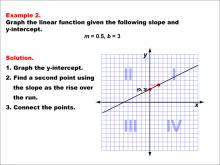
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 2	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 2 Topic Linear Functions Description This example illustrates the process of graphing a linear function with a slope of 0.5 and a y-intercept of 3. The method involves three main steps: plotting the y-intercept at (0, 3), using the slope to determine a second point on the line, and connecting these points to create the linear graph.	Slope-Intercept Form
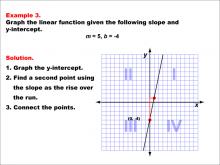
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 3	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 3 Topic Linear Functions Description This example demonstrates the process of graphing a linear function with a slope of 5 and a y-intercept of -4. The procedure involves three key steps: plotting the y-intercept at (0, -4), using the slope to determine a second point on the line, and connecting these points to form the linear graph.	Slope-Intercept Form
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 4	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 4 Topic Linear Functions Description This example illustrates the process of graphing a linear function with a slope of 0.1 and a y-intercept of -4. The method involves three main steps: plotting the y-intercept at (0, -4), using the slope to determine a second point on the line, and connecting these points to create the linear graph.	Slope-Intercept Form
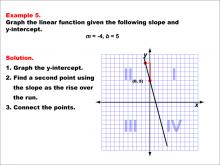
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 5	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 5 Topic Linear Functions Description This example demonstrates how to graph a linear function with a slope of -4 and a y-intercept of 5. The process involves three key steps: first, plotting the y-intercept at (0, 5); second, using the slope to find another point on the line; and finally, connecting these points to form the line.	Slope-Intercept Form
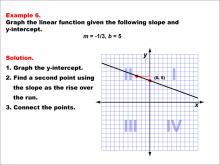
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 6	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 6 Topic Linear Functions Description This example illustrates the process of graphing a linear function with a slope of -1/3 and a y-intercept of 5. The method involves three main steps: plotting the y-intercept at (0, 5), using the slope to determine a second point on the line, and connecting these points to create the linear graph.	Slope-Intercept Form
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 7	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 7 Topic Linear Functions Description This example demonstrates the process of graphing a linear function with a slope of -3 and a y-intercept of -2. The procedure involves three key steps: plotting the y-intercept at (0, -2), using the slope to determine a second point on the line, and connecting these points to form the linear graph.	Slope-Intercept Form
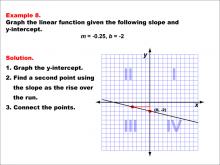
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 8	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 8 Topic Linear Functions Description This example illustrates the process of graphing a linear function with a slope of -0.25 and a y-intercept of -2. The method involves three main steps: plotting the y-intercept at (0, -2), using the slope to determine a second point on the line, and connecting these points to create the linear graph.	Slope-Intercept Form
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 9	Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 9 Topic Linear Functions Description This example demonstrates how to graph a linear function with a slope of 0.25 and a y-intercept of 0. The process involves three key steps: first, plotting the y-intercept at the origin (0, 0); second, using the slope to find another point on the line; and finally, connecting these points to form the line.	Slope-Intercept Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 1	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 1 Topic Linear Functions Description This example demonstrates the process of converting a linear equation from standard form to slope-intercept form. The equation 2x + 4y = 8 is solved step-by-step, isolating y and dividing by its coefficient. The result is y = -1/2 x + 2, clearly showing the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 10	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 10 Topic Linear Functions Description This example illustrates the conversion of the linear equation x + y = 1 from standard form to slope-intercept form. The process involves isolating y, resulting in y = -x + 1. This simple transformation clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 11	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 11 Topic Linear Functions Description This example showcases the transformation of the linear equation x + y = -1 from standard form to slope-intercept form. The process involves isolating y, resulting in y = -x - 1. This step-by-step solution clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 12	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 12 Topic Linear Functions Description This example demonstrates the conversion of the linear equation x - y = 1 from standard form to slope-intercept form. The solution process involves isolating y and changing the sign of both sides, resulting in y = x - 1. This transformation clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 13	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 13 Topic Linear Functions Description This example illustrates the process of converting the linear equation -x + y = 1 from standard form to slope-intercept form. The solution involves rearranging the equation to isolate y, resulting in y = x + 1. This transformation clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 14	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 14 Topic Linear Functions Description This example demonstrates the conversion of the linear equation -x - y = -1 from standard form to slope-intercept form. The process involves manipulating the equation to solve for y, yielding y = -x + 1. This transformation clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 15	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 15 Topic Linear Functions Description This example showcases the transformation of the linear equation -x - y = 1 from standard form to slope-intercept form. The solution process involves isolating y, resulting in y = -x - 1. This step-by-step conversion clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 16	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 16 Topic Linear Functions Description This example demonstrates the conversion of the linear equation -x + y = -1 from standard form to slope-intercept form. The process involves isolating y, resulting in y = x - 1. This transformation clearly reveals the slope and y-intercept of the line. Linear functions are fundamental mathematical concepts that describe relationships between two variables. The examples in this collection, such as showing step-by-step transformations from standard form to slope-intercept form, help in understanding how each part of the equation affects the graph and the relationship itself.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 17	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 17 Topic Linear Functions Description This example illustrates the conversion of the linear equation x - y = -1 from standard form to slope-intercept form. The solution involves isolating y, resulting in y = x + 1. This process clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 18	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 18 Topic Linear Functions Description This example demonstrates the conversion of the linear equation -x - y = 1 from standard form to slope-intercept form. The process involves rearranging the equation to isolate y, resulting in y = -x - 1. This transformation clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 19	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 19 Topic Linear Functions Description This example illustrates the conversion of the linear equation 12x + 28y = 0 from standard form to slope-intercept form. The solution involves isolating y and dividing by its coefficient, resulting in y = -3/7 x. This process clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 2	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 2 Topic Linear Functions Description This example illustrates the conversion of the linear equation 3x + 6y = -18 from standard form to slope-intercept form. The solution involves isolating y and dividing by its coefficient, resulting in y = -1/2 x - 3. This process clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 20	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 20 Topic Linear Functions Description This example demonstrates the conversion of the linear equation -14x - 35y = 0 from standard form to slope-intercept form. The process involves isolating y and dividing by its coefficient, resulting in y = -2/5 x. This transformation clearly reveals the slope and y-intercept of the line.	Standard Form
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 21	Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 21 Topic Linear Functions Description This example showcases the transformation of the linear equation 28x - 21y = 0 from standard form to slope-intercept form. The process involves isolating y and dividing by its coefficient, resulting in y = 4/3 x. This step-by-step solution clearly reveals the slope and y-intercept of the line.	Standard Form

Illustrative Math Alignment: Grade 8 Unit 3

Linear Relationships

Lesson 13: More Solutions to Linear Equations

Library of Instructional Resources

Library of Instructional Resources

Library of Instructional Resources

Library of Instructional Resources

Library of Instructional Resources

Library of Instructional Resources

Library of Instructional Resources

Library of Instructional Resources

Library of Instructional Resources

Library of Instructional Resources

Related Resources

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description

Topic

Description