
Illustrative Math Alignment: Grade 8 Unit 3
Linear Relationships
Lesson 6: More Linear Relationships
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|
|
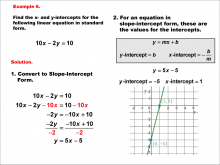
Math Example--Linear Function Concepts--Linear Intercepts: Example 6 | Math Example--Linear Function Concepts--Linear Intercepts: Example 6TopicLinear Functions DescriptionFind the x- and y-intercepts for the linear equation 10x - 2y = 10 given in standard form. Convert it to slope-intercept form. The equation is rewritten as y = 5x - 5. The y-intercept is -5 (where x = 0), and the x-intercept is 1 (where y = 0). These intercepts are plotted on a graph. Linear functions are a fundamental concept in algebra, where we explore the relationships between variables. The examples in this collection highlight how to find intercepts and analyze the relationships they represent in a linear context. |
Slope-Intercept Form and Standard Form |
|
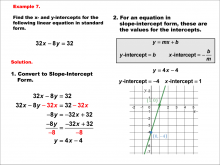
Math Example--Linear Function Concepts--Linear Intercepts: Example 7 | Math Example--Linear Function Concepts--Linear Intercepts: Example 7TopicLinear Functions DescriptionFind the x- and y-intercepts for the linear equation 32x - 8y = 32 given in standard form. Convert it to slope-intercept form. The equation is rewritten as y = 4x - 4. The y-intercept is -4 (where x = 0), and the x-intercept is 1 (where y = 0). These intercepts are plotted on a graph. Linear functions are a fundamental concept in algebra, where we explore the relationships between variables. The examples in this collection highlight how to find intercepts and analyze the relationships they represent in a linear context. |
Slope-Intercept Form and Standard Form |

|
Math Example--Linear Function Concepts--Linear Intercepts: Example 7 | Math Example--Linear Function Concepts--Linear Intercepts: Example 7TopicLinear Functions DescriptionFind the x- and y-intercepts for the linear equation 32x - 8y = 32 given in standard form. Convert it to slope-intercept form. The equation is rewritten as y = 4x - 4. The y-intercept is -4 (where x = 0), and the x-intercept is 1 (where y = 0). These intercepts are plotted on a graph. Linear functions are a fundamental concept in algebra, where we explore the relationships between variables. The examples in this collection highlight how to find intercepts and analyze the relationships they represent in a linear context. |
Slope-Intercept Form and Standard Form |
|
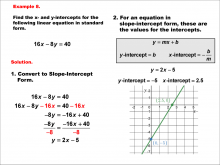
Math Example--Linear Function Concepts--Linear Intercepts: Example 8 | Math Example--Linear Function Concepts--Linear Intercepts: Example 8TopicLinear Functions DescriptionFind the x- and y-intercepts for the linear equation 16x - 8y = 40 given in standard form. Convert it to slope-intercept form. The equation is rewritten as y = 2x - 5. The y-intercept is -5 (where x = 0), and the x-intercept is 2.5 (where y = 0). These intercepts are plotted on a graph. Linear functions are a fundamental concept in algebra, where we explore the relationships between variables. The examples in this collection highlight how to find intercepts and analyze the relationships they represent in a linear context. |
Slope-Intercept Form and Standard Form |

|
Math Example--Linear Function Concepts--Linear Intercepts: Example 8 | Math Example--Linear Function Concepts--Linear Intercepts: Example 8TopicLinear Functions DescriptionFind the x- and y-intercepts for the linear equation 16x - 8y = 40 given in standard form. Convert it to slope-intercept form. The equation is rewritten as y = 2x - 5. The y-intercept is -5 (where x = 0), and the x-intercept is 2.5 (where y = 0). These intercepts are plotted on a graph. Linear functions are a fundamental concept in algebra, where we explore the relationships between variables. The examples in this collection highlight how to find intercepts and analyze the relationships they represent in a linear context. |
Slope-Intercept Form and Standard Form |
|
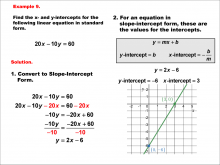
Math Example--Linear Function Concepts--Linear Intercepts: Example 9 | Math Example--Linear Function Concepts--Linear Intercepts: Example 9TopicLinear Functions DescriptionFind the x- and y-intercepts for the linear equation 20x - 10y = 60 given in standard form. Convert it to slope-intercept form. The equation is rewritten as y = 2x - 6. The y-intercept is -6 (where x = 0), and the x-intercept is 3 (where y = 0). These intercepts are plotted on a graph. Linear functions are a fundamental concept in algebra, where we explore the relationships between variables. The examples in this collection highlight how to find intercepts and analyze the relationships they represent in a linear context. |
Slope-Intercept Form and Standard Form |

|
Math Example--Linear Function Concepts--Linear Intercepts: Example 9 | Math Example--Linear Function Concepts--Linear Intercepts: Example 9TopicLinear Functions DescriptionFind the x- and y-intercepts for the linear equation 20x - 10y = 60 given in standard form. Convert it to slope-intercept form. The equation is rewritten as y = 2x - 6. The y-intercept is -6 (where x = 0), and the x-intercept is 3 (where y = 0). These intercepts are plotted on a graph. Linear functions are a fundamental concept in algebra, where we explore the relationships between variables. The examples in this collection highlight how to find intercepts and analyze the relationships they represent in a linear context. |
Slope-Intercept Form and Standard Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1TopicLinear Functions DescriptionThis example demonstrates how to find the equation of a line passing through two given points: (6, 4) and (8, 8). The slope is calculated using the formula (y2 - y1) / (x2 - x1), resulting in a slope of 2. Using the point-slope form of a line, y - y1 = m(x - x1), the equation is derived as y - 8 = 2(x - 8), which simplifies to y = 2x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1TopicLinear Functions DescriptionThis example demonstrates how to find the equation of a line passing through two given points: (6, 4) and (8, 8). The slope is calculated using the formula (y2 - y1) / (x2 - x1), resulting in a slope of 2. Using the point-slope form of a line, y - y1 = m(x - x1), the equation is derived as y - 8 = 2(x - 8), which simplifies to y = 2x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1TopicLinear Functions DescriptionThis example demonstrates how to find the equation of a line passing through two given points: (6, 4) and (8, 8). The slope is calculated using the formula (y2 - y1) / (x2 - x1), resulting in a slope of 2. Using the point-slope form of a line, y - y1 = m(x - x1), the equation is derived as y - 8 = 2(x - 8), which simplifies to y = 2x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1TopicLinear Functions DescriptionThis example demonstrates how to find the equation of a line passing through two given points: (6, 4) and (8, 8). The slope is calculated using the formula (y2 - y1) / (x2 - x1), resulting in a slope of 2. Using the point-slope form of a line, y - y1 = m(x - x1), the equation is derived as y - 8 = 2(x - 8), which simplifies to y = 2x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1TopicLinear Functions DescriptionThis example demonstrates how to find the equation of a line passing through two given points: (6, 4) and (8, 8). The slope is calculated using the formula (y2 - y1) / (x2 - x1), resulting in a slope of 2. Using the point-slope form of a line, y - y1 = m(x - x1), the equation is derived as y - 8 = 2(x - 8), which simplifies to y = 2x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 1TopicLinear Functions DescriptionThis example demonstrates how to find the equation of a line passing through two given points: (6, 4) and (8, 8). The slope is calculated using the formula (y2 - y1) / (x2 - x1), resulting in a slope of 2. Using the point-slope form of a line, y - y1 = m(x - x1), the equation is derived as y - 8 = 2(x - 8), which simplifies to y = 2x - 8. |
Point-Slope Form and Slope-Intercept Form |
|
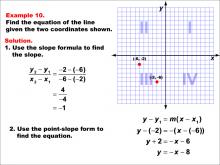
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-6, -2) and (-2, -6) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated, and the equation is derived using point-slope form. The slope is calculated as (y2 - y1) / (x2 - x1) = (-6 - (-2)) / (-2 - (-6)) = -4 / 4 = -1. Using point-slope form, y - y1 = m(x - x1), the equation is derived as y + 2 = -(x + 6), which simplifies to y = -x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-6, -2) and (-2, -6) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated, and the equation is derived using point-slope form. The slope is calculated as (y2 - y1) / (x2 - x1) = (-6 - (-2)) / (-2 - (-6)) = -4 / 4 = -1. Using point-slope form, y - y1 = m(x - x1), the equation is derived as y + 2 = -(x + 6), which simplifies to y = -x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-6, -2) and (-2, -6) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated, and the equation is derived using point-slope form. The slope is calculated as (y2 - y1) / (x2 - x1) = (-6 - (-2)) / (-2 - (-6)) = -4 / 4 = -1. Using point-slope form, y - y1 = m(x - x1), the equation is derived as y + 2 = -(x + 6), which simplifies to y = -x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-6, -2) and (-2, -6) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated, and the equation is derived using point-slope form. The slope is calculated as (y2 - y1) / (x2 - x1) = (-6 - (-2)) / (-2 - (-6)) = -4 / 4 = -1. Using point-slope form, y - y1 = m(x - x1), the equation is derived as y + 2 = -(x + 6), which simplifies to y = -x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-6, -2) and (-2, -6) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated, and the equation is derived using point-slope form. The slope is calculated as (y2 - y1) / (x2 - x1) = (-6 - (-2)) / (-2 - (-6)) = -4 / 4 = -1. Using point-slope form, y - y1 = m(x - x1), the equation is derived as y + 2 = -(x + 6), which simplifies to y = -x - 8. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 10TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-6, -2) and (-2, -6) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated, and the equation is derived using point-slope form. The slope is calculated as (y2 - y1) / (x2 - x1) = (-6 - (-2)) / (-2 - (-6)) = -4 / 4 = -1. Using point-slope form, y - y1 = m(x - x1), the equation is derived as y + 2 = -(x + 6), which simplifies to y = -x - 8. |
Point-Slope Form and Slope-Intercept Form |
|
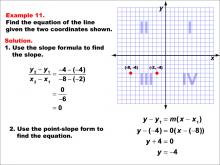
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-8, -4) and (-2, -4) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated as zero, indicating a horizontal line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (-8 - (-2)) = 0 / -6 = 0. Since the slope is zero, it indicates a horizontal line at y = -4. Using point-slope form, the equation becomes y + 4 = 0, which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-8, -4) and (-2, -4) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated as zero, indicating a horizontal line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (-8 - (-2)) = 0 / -6 = 0. Since the slope is zero, it indicates a horizontal line at y = -4. Using point-slope form, the equation becomes y + 4 = 0, which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-8, -4) and (-2, -4) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated as zero, indicating a horizontal line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (-8 - (-2)) = 0 / -6 = 0. Since the slope is zero, it indicates a horizontal line at y = -4. Using point-slope form, the equation becomes y + 4 = 0, which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-8, -4) and (-2, -4) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated as zero, indicating a horizontal line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (-8 - (-2)) = 0 / -6 = 0. Since the slope is zero, it indicates a horizontal line at y = -4. Using point-slope form, the equation becomes y + 4 = 0, which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-8, -4) and (-2, -4) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated as zero, indicating a horizontal line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (-8 - (-2)) = 0 / -6 = 0. Since the slope is zero, it indicates a horizontal line at y = -4. Using point-slope form, the equation becomes y + 4 = 0, which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 11TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-8, -4) and (-2, -4) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is calculated as zero, indicating a horizontal line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (-8 - (-2)) = 0 / -6 = 0. Since the slope is zero, it indicates a horizontal line at y = -4. Using point-slope form, the equation becomes y + 4 = 0, which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |
|
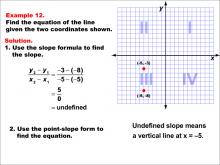
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-5, -8) and (-5, -3) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is undefined, indicating a vertical line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-3 - (-8)) / (-5 - (-5)) = 5 / 0, which is undefined. Since the slope is undefined, it indicates a vertical line at x = -5. Therefore, the equation of the line is simply x = -5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-5, -8) and (-5, -3) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is undefined, indicating a vertical line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-3 - (-8)) / (-5 - (-5)) = 5 / 0, which is undefined. Since the slope is undefined, it indicates a vertical line at x = -5. Therefore, the equation of the line is simply x = -5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-5, -8) and (-5, -3) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is undefined, indicating a vertical line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-3 - (-8)) / (-5 - (-5)) = 5 / 0, which is undefined. Since the slope is undefined, it indicates a vertical line at x = -5. Therefore, the equation of the line is simply x = -5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-5, -8) and (-5, -3) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is undefined, indicating a vertical line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-3 - (-8)) / (-5 - (-5)) = 5 / 0, which is undefined. Since the slope is undefined, it indicates a vertical line at x = -5. Therefore, the equation of the line is simply x = -5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-5, -8) and (-5, -3) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is undefined, indicating a vertical line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-3 - (-8)) / (-5 - (-5)) = 5 / 0, which is undefined. Since the slope is undefined, it indicates a vertical line at x = -5. Therefore, the equation of the line is simply x = -5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 12TopicLinear Functions DescriptionThe image shows a coordinate plane with two points (-5, -8) and (-5, -3) marked. It provides a step-by-step solution to find the equation of the line passing through these points. The slope is undefined, indicating a vertical line. The slope is calculated as (y2 - y1) / (x2 - x1) = (-3 - (-8)) / (-5 - (-5)) = 5 / 0, which is undefined. Since the slope is undefined, it indicates a vertical line at x = -5. Therefore, the equation of the line is simply x = -5. |
Point-Slope Form and Slope-Intercept Form |
|
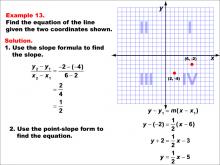
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13TopicLinear Functions DescriptionThis image shows a graph with two points (2, -4) and (6, -2) marked. The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as 1/2, and the point-slope form is used to derive the equation of the line. The slope formula is used: (y2 - y1) / (x2 - x1) = (-2 - (-4)) / (6 - 2) = 2 / 4 = 1/2. Then, using the point-slope form: y - (-2) = (1/2)(x - 6), which simplifies to y = (1/2)x - 5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13TopicLinear Functions DescriptionThis image shows a graph with two points (2, -4) and (6, -2) marked. The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as 1/2, and the point-slope form is used to derive the equation of the line. The slope formula is used: (y2 - y1) / (x2 - x1) = (-2 - (-4)) / (6 - 2) = 2 / 4 = 1/2. Then, using the point-slope form: y - (-2) = (1/2)(x - 6), which simplifies to y = (1/2)x - 5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13TopicLinear Functions DescriptionThis image shows a graph with two points (2, -4) and (6, -2) marked. The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as 1/2, and the point-slope form is used to derive the equation of the line. The slope formula is used: (y2 - y1) / (x2 - x1) = (-2 - (-4)) / (6 - 2) = 2 / 4 = 1/2. Then, using the point-slope form: y - (-2) = (1/2)(x - 6), which simplifies to y = (1/2)x - 5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13TopicLinear Functions DescriptionThis image shows a graph with two points (2, -4) and (6, -2) marked. The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as 1/2, and the point-slope form is used to derive the equation of the line. The slope formula is used: (y2 - y1) / (x2 - x1) = (-2 - (-4)) / (6 - 2) = 2 / 4 = 1/2. Then, using the point-slope form: y - (-2) = (1/2)(x - 6), which simplifies to y = (1/2)x - 5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13TopicLinear Functions DescriptionThis image shows a graph with two points (2, -4) and (6, -2) marked. The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as 1/2, and the point-slope form is used to derive the equation of the line. The slope formula is used: (y2 - y1) / (x2 - x1) = (-2 - (-4)) / (6 - 2) = 2 / 4 = 1/2. Then, using the point-slope form: y - (-2) = (1/2)(x - 6), which simplifies to y = (1/2)x - 5. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 13TopicLinear Functions DescriptionThis image shows a graph with two points (2, -4) and (6, -2) marked. The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as 1/2, and the point-slope form is used to derive the equation of the line. The slope formula is used: (y2 - y1) / (x2 - x1) = (-2 - (-4)) / (6 - 2) = 2 / 4 = 1/2. Then, using the point-slope form: y - (-2) = (1/2)(x - 6), which simplifies to y = (1/2)x - 5. |
Point-Slope Form and Slope-Intercept Form |
|
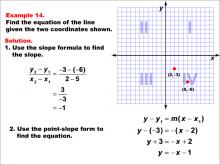
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14TopicLinear Functions DescriptionThis image shows a graph with two points (2, -3) and (5, -6). The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as -1, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-3 - (-6)) / (2 - 5) = 3 / -3 = -1. Then, using the point-slope form: y - (-3) = -(x - 2), which simplifies to y = -x - 1. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14TopicLinear Functions DescriptionThis image shows a graph with two points (2, -3) and (5, -6). The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as -1, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-3 - (-6)) / (2 - 5) = 3 / -3 = -1. Then, using the point-slope form: y - (-3) = -(x - 2), which simplifies to y = -x - 1. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14TopicLinear Functions DescriptionThis image shows a graph with two points (2, -3) and (5, -6). The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as -1, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-3 - (-6)) / (2 - 5) = 3 / -3 = -1. Then, using the point-slope form: y - (-3) = -(x - 2), which simplifies to y = -x - 1. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14TopicLinear Functions DescriptionThis image shows a graph with two points (2, -3) and (5, -6). The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as -1, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-3 - (-6)) / (2 - 5) = 3 / -3 = -1. Then, using the point-slope form: y - (-3) = -(x - 2), which simplifies to y = -x - 1. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14TopicLinear Functions DescriptionThis image shows a graph with two points (2, -3) and (5, -6). The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as -1, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-3 - (-6)) / (2 - 5) = 3 / -3 = -1. Then, using the point-slope form: y - (-3) = -(x - 2), which simplifies to y = -x - 1. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 14TopicLinear Functions DescriptionThis image shows a graph with two points (2, -3) and (5, -6). The example demonstrates how to find the equation of a line passing through these points. The slope is calculated as -1, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-3 - (-6)) / (2 - 5) = 3 / -3 = -1. Then, using the point-slope form: y - (-3) = -(x - 2), which simplifies to y = -x - 1. |
Point-Slope Form and Slope-Intercept Form |
|
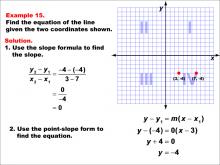
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15TopicLinear Functions DescriptionThis image shows a graph with two points (3, -4) and (7, -4). The example demonstrates how to find the equation of a horizontal line passing through these points. The slope is calculated as 0, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (7 - 3) = 0 / 4 = 0. Then, using the point-slope form: y - (-4) = 0(x - 3), which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15TopicLinear Functions DescriptionThis image shows a graph with two points (3, -4) and (7, -4). The example demonstrates how to find the equation of a horizontal line passing through these points. The slope is calculated as 0, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (7 - 3) = 0 / 4 = 0. Then, using the point-slope form: y - (-4) = 0(x - 3), which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15TopicLinear Functions DescriptionThis image shows a graph with two points (3, -4) and (7, -4). The example demonstrates how to find the equation of a horizontal line passing through these points. The slope is calculated as 0, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (7 - 3) = 0 / 4 = 0. Then, using the point-slope form: y - (-4) = 0(x - 3), which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15TopicLinear Functions DescriptionThis image shows a graph with two points (3, -4) and (7, -4). The example demonstrates how to find the equation of a horizontal line passing through these points. The slope is calculated as 0, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (7 - 3) = 0 / 4 = 0. Then, using the point-slope form: y - (-4) = 0(x - 3), which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15TopicLinear Functions DescriptionThis image shows a graph with two points (3, -4) and (7, -4). The example demonstrates how to find the equation of a horizontal line passing through these points. The slope is calculated as 0, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (7 - 3) = 0 / 4 = 0. Then, using the point-slope form: y - (-4) = 0(x - 3), which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |

|
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 15TopicLinear Functions DescriptionThis image shows a graph with two points (3, -4) and (7, -4). The example demonstrates how to find the equation of a horizontal line passing through these points. The slope is calculated as 0, and the point-slope form is used to derive the equation. The slope formula is used: (y2 - y1) / (x2 - x1) = (-4 - (-4)) / (7 - 3) = 0 / 4 = 0. Then, using the point-slope form: y - (-4) = 0(x - 3), which simplifies to y = -4. |
Point-Slope Form and Slope-Intercept Form |
|
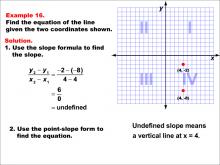
Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 16 | Math Example--Linear Function Concepts--The Equation of a Line Given Two Points: Example 16TopicLinear Functions DescriptionThis image shows a graph with two points (4, -8) and (4, -2). The example demonstrates how to find the equation of a vertical line passing through these points. The slope is undefined because division by zero occurs in calculating it. The slope formula is used: (y2 - y1) / (x2 - x1) = (-2 - (-8)) / (4 - 4) = 6 / 0 = undefined. Since this represents a vertical line, its equation is simply x = 4. |
Point-Slope Form and Slope-Intercept Form |