
Illustrative Math Alignment: Grade 7 Unit 7
Angles, Triangles, and Prisms
Lesson 1: Relationships of Angles
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Nodes |
|---|---|---|---|

|
Closed Captioned Video: Geometry Applications: Angles and Planes | Closed Captioned Video: Geometry Applications: Angles and PlanesTopicGeometry Basics |
Applications of Angles and Planes |

|
Closed Captioned Video: Geometry Applications: Angles and Planes, 1 | Closed Captioned Video: Geometry Applications: Angles and Planes, 1TopicGeometry Basics DescriptionExplore Geometry Concepts: This segment introduces angles and planes through the Earth's orbital ecliptic and its 23-degree tilt. It connects these geometric principles to real-world phenomena, such as seasons and Kepler's laws, laying a foundation for subsequent discussions on angles and planes. |
Applications of Angles and Planes |

|
Closed Captioned Video: Geometry Applications: Angles and Planes, 2 | Closed Captioned Video: Geometry Applications: Angles and Planes, 2TopicGeometry Basics DescriptionExplore Geometry Concepts: This segment focuses on the strategic use of angles in Himeji Castle for defense. It explains tangent lines, line-of-sight angles, and acute angle measurements, connecting these concepts to historical architecture and modern geometry tools for analysis. |
Applications of Angles and Planes |

|
Closed Captioned Video: Geometry Applications: Angles and Planes, 3 | Closed Captioned Video: Geometry Applications: Angles and Planes, 3TopicGeometry Basics DescriptionExplore Geometry Concepts: This segment delves into parallel planes, illustrated by sedimentary rock layers in fossil analysis. It shows how these concepts are used to determine the relative age of fossils and explores how tectonic activity can alter parallel planes to create geological intersections. |
Applications of Angles and Planes |

|
Closed Captioned Video: Geometry Applications: Triangles | Closed Captioned Video: Geometry Applications: TrianglesTopicTriangles |
Definition of a Triangle and Applications of Triangles |

|
Closed Captioned Video: Geometry Applications: Triangles, 1 | Closed Captioned Video: Geometry Applications: Triangles, 1TopicTriangles |
Definition of a Triangle and Applications of Triangles |

|
Closed Captioned Video: Geometry Applications: Triangles, 2 | Closed Captioned Video: Geometry Applications: Triangles, 2TopicTriangles |
Definition of a Triangle and Applications of Triangles |

|
Closed Captioned Video: Geometry Applications: Triangles, 3 | Closed Captioned Video: Geometry Applications: Triangles, 3TopicTriangles |
Definition of a Triangle and Applications of Triangles |

|
Closed Captioned Video: Ratios and Proportions: Scale Drawings | Closed Captioned Video: Ratios and Proportions: Scale DrawingsTopicRatios DescriptionThe video explains how proportions are used to create scale drawings, ensuring geometric figures remain proportional. Examples include finding dimensions in similar triangles, scaling architectural models, and solving geometric problems. The concept of proportional relationships is key to accurate scaling. |
Ratios and Rates |
|
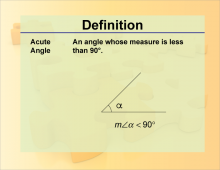
Definition--Angle Concepts--Acute Angle | Acute AngleTopicAngles DefinitionAn acute angle is an angle that measures less than 90 degrees. |
Definition of an Angle |

|
Definition--Angle Concepts--Adjacent Angles | Adjacent AnglesTopicAngles DefinitionAdjacent angles are two angles that share a common vertex and side, but do not overlap. |
Definition of an Angle |
|
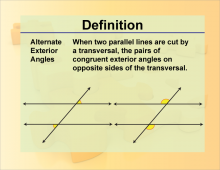
Definition--Angle Concepts--Alternate Exterior Angles | Alternate Exterior AnglesTopicAngles DefinitionAlternate exterior angles are pairs of angles formed when a transversal crosses two parallel lines, and they lie on opposite sides of the transversal and outside the parallel lines. |
Definition of an Angle |
|
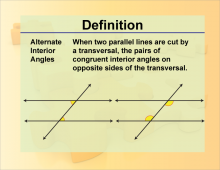
Definition--Angle Concepts--Alternate Interior Angles | Alternate Interior AnglesTopicAngles DefinitionAlternate interior angles are pairs of angles formed when a transversal crosses two parallel lines, and they lie on opposite sides of the transversal and inside the parallel lines. |
Definition of an Angle |
|
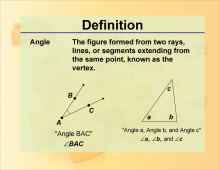
Definition--Angle Concepts--Angle | AngleTopicAngles DefinitionAn angle is formed by two rays with a common endpoint, known as the vertex. DescriptionAngles are a fundamental concept in geometry, forming the basis for understanding shapes and their properties. They are used in a wide range of applications, from measuring land and constructing buildings to designing machinery and creating art. Angles are measured in degrees or radians, and their understanding is essential for solving problems involving triangles, circles, and other geometric figures. In education, angles are introduced early in the curriculum to develop spatial awareness and geometric reasoning, providing a foundation for more advanced mathematical concepts. |
Definition of an Angle |
|
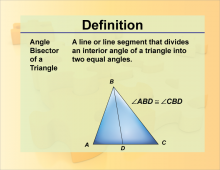
Definition--Angle Concepts--Angle Bisector | Angle BisectorTopicAngles DefinitionAn angle bisector is a line or ray that divides an angle into two equal parts. DescriptionAngle bisectors are used in various geometric constructions and proofs, such as constructing the incenter of a triangle, which is the point where the angle bisectors of a triangle intersect. In practical applications, angle bisectors are used in design and architecture to create symmetrical and balanced structures. For students, learning about angle bisectors enhances their understanding of geometric constructions and the properties of angles, contributing to their overall mathematical proficiency. |
Definition of an Angle |
|
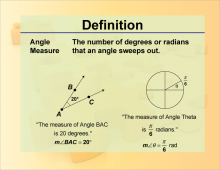
Definition--Angle Concepts--Angle Measure | Angle MeasureTopicAngles DefinitionAngle measure refers to the size of an angle, typically expressed in degrees or radians. DescriptionUnderstanding angle measurement is crucial for solving geometric problems and for applications in trigonometry, physics, and engineering. Accurate angle measurement is essential in fields such as surveying, navigation, and construction, where precise calculations are necessary for successful outcomes. In education, mastering angle measurement is a key component of the geometry curriculum, helping students develop skills in spatial reasoning and problem-solving. |
Definition of an Angle |
|
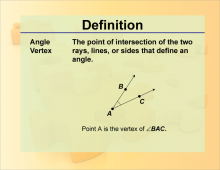
Definition--Angle Concepts--Angle Vertex | Angle VertexTopicAngles DefinitionThe vertex of an angle is the common endpoint of the two rays that form the angle. |
Definition of an Angle |

|
Definition--Angle Concepts--Central Angle | Central AngleTopicAngles DefinitionA central angle is an angle whose vertex is at the center of a circle and whose sides are radii of the circle. DescriptionCentral angles are important in the study of circles and are used to define arcs and sectors. They are commonly used in problems involving circular motion and in the design of circular objects, such as wheels and gears. In mathematics education, understanding central angles helps students grasp the properties of circles and their applications, forming a basis for more advanced studies in trigonometry and calculus. |
Definition of an Angle |
|
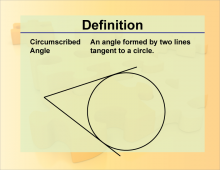
Definition--Angle Concepts--Circumscribed Angle | Circumscribed AngleTopicAngles DefinitionA circumscribed angle is an angle formed outside a circle by two intersecting lines, each tangent to the circle. DescriptionCircumscribed angles are used in geometry to study the properties of tangents and their relationships with circles. They are important in problems involving circle theorems and are applied in fields such as architecture and design, where circular shapes are common. For students, learning about circumscribed angles enhances their understanding of circle geometry and the relationships between angles and arcs, contributing to their overall mathematical knowledge. |
Definition of an Angle |

|
Definition--Angle Concepts--Complementary Angle | Complementary AngleTopicAngles DefinitionComplementary angles are two angles whose measures add up to 90 degrees. |
Definition of an Angle |
|
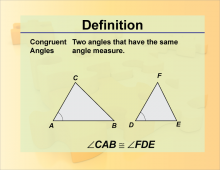
Definition--Angle Concepts--Congruent Angles | Congruent AnglesTopicAngles DefinitionCongruent angles are angles that have the same measure. DescriptionCongruent angles are a key concept in geometry, often used in proving the similarity and congruence of geometric figures. They are essential in understanding the properties of polygons, where congruent angles indicate symmetry and balance. In real-world applications, congruent angles are used in design and engineering to ensure that components are identical and fit together seamlessly. For students, learning about congruent angles enhances their understanding of geometric relationships and proofs, contributing to their overall mathematical proficiency. |
Definition of an Angle |

|
Definition--Angle Concepts--Corresponding Angles | Corresponding AnglesTopicAngles DefinitionCorresponding angles are pairs of angles that are in similar positions at each intersection where a transversal crosses two lines. |
Definition of an Angle |
|
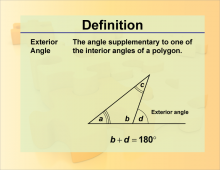
Definition--Angle Concepts--Exterior Angle | Exterior AngleTopicAngles DefinitionAn exterior angle is an angle formed outside a polygon by extending one of its sides. |
Definition of an Angle |
|
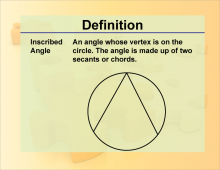
Definition--Angle Concepts--Inscribed Angle | Inscribed AngleTopicAngles DefinitionAn inscribed angle is an angle formed by two chords in a circle that have a common endpoint. DescriptionInscribed angles are important in the study of circles, where they are used to define arcs and sectors. They are commonly used in problems involving circle theorems and in the design of circular objects, such as wheels and gears. In mathematics education, understanding inscribed angles helps students grasp the properties of circles and their applications, forming a basis for more advanced studies in trigonometry and calculus. |
Definition of an Angle |
|
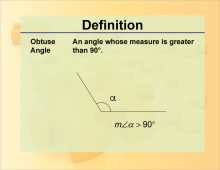
Definition--Angle Concepts--Obtuse Angle | Obtuse AngleTopicAngles DefinitionAn obtuse angle is an angle that measures more than 90 degrees but less than 180 degrees. |
Definition of an Angle |
|
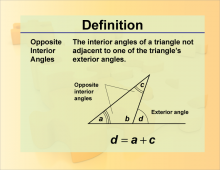
Definition--Angle Concepts--Opposite Interior Angles | Opposite Interior AnglesTopicAngles DefinitionOpposite interior angles are pairs of angles opposite the exterior angle of a triangle. In the case of parallel lines cut by a transversal, they are congruent. |
Definition of an Angle |

|
Definition--Angle Concepts--Reflex Angle | Reflex AngleTopicAngles DefinitionA reflex angle is an angle that measures more than 180 degrees but less than 360 degrees. DescriptionReflex angles are often encountered in the study of rotations and circular motion, where they are used to describe angles that extend beyond a straight line. They are important in fields such as physics and engineering, where understanding rotations and angular measurements is crucial. In mathematics education, reflex angles help students develop a comprehensive understanding of angle measurement and classification, contributing to their overall geometric reasoning and problem-solving abilities. |
Definition of an Angle |

|
Definition--Angle Concepts--Right Angle | Right AngleTopicAngles DefinitionA right angle is an angle that measures exactly 90 degrees. DescriptionRight angles are fundamental in geometry and are often used as a reference for measuring other angles. They are commonly found in various geometric shapes and real-world structures, such as squares, rectangles, and buildings. In real-world applications, right angles are essential in construction and design, where they ensure that elements are perpendicular and aligned correctly. In education, understanding right angles is crucial for learning about perpendicularity and the properties of geometric figures, forming a basis for more advanced mathematical concepts. |
Definition of an Angle |

|
Definition--Angle Concepts--Straight Angle | Straight AngleTopicAngles DefinitionA straight angle is an angle that measures exactly 180 degrees. DescriptionStraight angles are used to describe angles that form a straight line, often encountered in the study of linear relationships and transformations. They are important in fields such as physics and engineering, where understanding linear motion and alignment is crucial. In mathematics education, straight angles help students develop a comprehensive understanding of angle measurement and classification, contributing to their overall geometric reasoning and problem-solving abilities. |
Definition of an Angle |

|
Definition--Angle Concepts--Supplementary Angles | Supplementary AnglesTopicAngles DefinitionSupplementary angles are two angles whose measures add up to 180 degrees. |
Definition of an Angle |
|
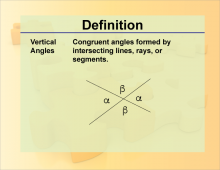
Definition--Angle Concepts--Vertical Angles | Vertical AnglesTopicAngles DefinitionVertical angles are pairs of opposite angles formed by two intersecting lines, and they are equal in measure. |
Definition of an Angle |
|
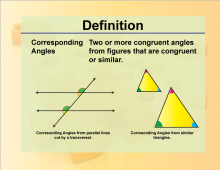
Definition--Geometry Basics--Corresponding Angles | Corresponding AnglesTopicGeometry Basics DefinitionCorresponding angles are angles that are in the same relative position at each intersection where a straight line crosses two others. DescriptionCorresponding angles are formed when a transversal intersects two parallel lines. These angles are equal in measure, which is a key concept in proving the properties of parallel lines and solving geometric problems. For example, if two parallel lines are cut by a transversal, then each pair of corresponding angles is equal. This concept is used extensively in geometry to establish relationships between angles and to solve complex problems involving parallel lines. |
Definition of an Angle |
|
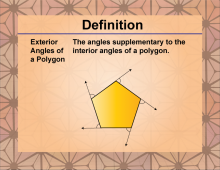
Definition--Polygon Concepts--Exterior Angles of a Polygon | Exterior Angles of a PolygonTopicPolygons DefinitionAn exterior angle of a polygon is the angle formed between any side of the polygon and the extension of an adjacent side. |
Definition of a Polygon |

|
Definition--Polygon Concepts--Interior Angles of a Polygon | Sum of Interior Angles of a PolygonTopicPolygons DefinitionThe sum of interior angles of a polygon with n sides is given by the formula: (n - 2) × 180°, where n is the number of sides in the polygon. DescriptionThe sum of interior angles is a fundamental property of polygons that plays a crucial role in geometry. This concept is essential for understanding the structure and properties of various polygons, from simple triangles to complex multi-sided figures. The formula (n - 2) × 180° provides a quick and efficient way to calculate the total measure of all interior angles in any polygon, regardless of its regularity. |
Definition of a Polygon |

|
Definition--Polygon Concepts--Sum of the Exterior Angles of a Polygon | Sum of Exterior Angles of a PolygonTopicPolygons DefinitionThe sum of the exterior angles of any polygon, regardless of the number of sides, is always 360°. DescriptionThe sum of exterior angles of a polygon is a fundamental concept in geometry that holds true for all polygons, regardless of their shape or number of sides. This property is crucial for understanding the nature of polygons and their relationship to circular motion. Exterior angles are formed by extending each side of the polygon and measuring the angle between this extension and the adjacent side. |
Definition of a Polygon |
|
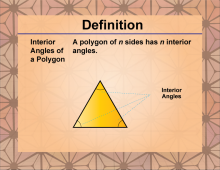
Definition--Polygon Concepts--Sum of the Interior Angles of a Polygon | Interior Angles of a PolygonTopicPolygons DefinitionAn interior angle of a polygon is an angle formed inside the polygon by two adjacent sides. |
Definition of a Polygon |
|
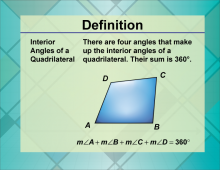
Definition--Quadrilateral Concepts--Interior Angles of a Quadrilateral | Interior Angles of a QuadrilateralTopicQuadrilaterals DefinitionThe sum of the interior angles of a quadrilateral is always 360 degrees. |
Definition of a Quadrilateral |
|
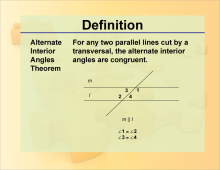
Definition--Theorems and Postulates--Alternate Interior Angles Theorem | Definition--Theorems and Postulates--Alternate Interior Angles Theorem
This is part of a collection of definitions of geometric theorems and postulates. |
Definition of an Angle |

|
Definition--Theorems and Postulates--Converse of Same-Side Interior Angles Postulate | Definition--Theorems and Postulates--Converse of Same-Side Interior Angles Postulate
This is part of a collection of definitions of geometric theorems and postulates. |
Definition of an Angle |

|
Definition--Theorems and Postulates--Converse of the Alternate Interior Angles Theorem | Definition--Theorems and Postulates--Converse of the Alternate Interior Angles Theorem
This is part of a collection of definitions of geometric theorems and postulates. |
Definition of an Angle |

|
Definition--Theorems and Postulates--Converse of the Corresponding Angles Theorem | Definition--Theorems and Postulates--Converse of the Corresponding Angles Theorem
This is part of a collection of definitions of geometric theorems and postulates. |
Definition of an Angle |

|
Definition--Theorems and Postulates--Corresponding Angles Theorem | Definition | Theorems and Postulates | Corresponding Angles Theorem
This is part of a collection of definitions of geometric theorems and postulates. —CLICK PREVIEW TO SEE THE DEFINITION— To see the complete collection of theorems and postulates, click on this link.The following section includes background information on angles. What Are Angles?In this illustration two rays intersect at a point. Points A and B are on one ray and points B and C are on another ray. These two rays form an angle. |
Definition of an Angle |

|
Definition--Theorems and Postulates--Same-Side Interior Angles Postulate | Definition--Theorems and Postulates--Same-Side Interior Angles Postulate
This is part of a collection of definitions of geometric theorems and postulates. |
Definition of a Triangle |

|
Definition--Triangle Concepts--30-60-90 Right Triangles | 30-60-90 Right TrianglesTopicTriangles DefinitionA 30-60-90 right triangle is a right triangle with angles of 30 degrees, 60 degrees, and 90 degrees. DescriptionThe 30-60-90 triangle is a special right triangle characterized by its angle measures. The sides of this triangle are in the ratio 1:√3:2, meaning the length of the hypotenuse is twice the length of the shorter leg, and the length of the longer leg is √3 times the length of the shorter leg. This relationship can be derived using trigonometric principles and is fundamental in geometry. |
Definition of a Triangle |

|
Definition--Triangle Concepts--45-45-90 Right Triangles | 45-45-90 Right TrianglesTopicTriangles DefinitionA 45-45-90 right triangle is a right triangle with two 45-degree angles and one 90-degree angle. DescriptionThe 45-45-90 triangle is another special right triangle where the two legs are of equal length, and the hypotenuse is √2 times the length of each leg. This type of triangle is also known as an isosceles right triangle due to its equal legs. |
Definition of a Triangle |

|
Definition--Triangle Concepts--Congruent Triangles | Congruent TrianglesTopicTriangles DefinitionCongruent triangles are triangles that have the same size and shape, with corresponding sides and angles that are equal. DescriptionCongruent triangles are fundamental in geometry, representing triangles that are identical in size and shape. This means that all corresponding sides and angles of congruent triangles are equal. The concept of congruence is crucial for proving various geometric theorems and properties. |
Definition of a Triangle |
|
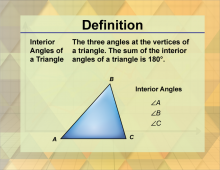
Definition--Triangle Concepts--Interior Angles of a Triangle | Interior Angles of a TriangleTopicTriangles DefinitionThe interior angles of a triangle are the angles inside the triangle, and their sum is always 180 degrees. DescriptionThe interior angles of a triangle are a fundamental concept in geometry, representing the angles inside the triangle. The sum of the interior angles of any triangle is always 180 degrees, which is a crucial property used in various geometric proofs and constructions. |
Definition of a Triangle |
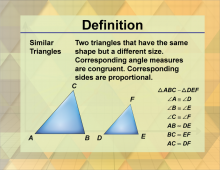
|
Definition--Triangle Concepts--Similar Triangles | Similar TrianglesTopicTriangles DefinitionSimilar triangles are triangles that have the same shape but not necessarily the same size, with corresponding angles equal and corresponding sides proportional. DescriptionSimilar triangles are fundamental in geometry, representing triangles that have the same shape but not necessarily the same size. This means that all corresponding angles are equal, and all corresponding sides are proportional. |
Definition of a Triangle |

|
Geometry Applications Teachers Guide: Angles and Planes | Geometry Applications Teachers Guide: Angles and Planes
This is the Teacher's Guide that accompanies Geometry Applications: Angles and Planes. This is part of a collection of teacher's guides. To see the complete collection of teacher's guides, click on this link. Note: The download is a PDF file.Related ResourcesTo see resources related to this topic click on the Related Resources tab above. |
Applications of Angles and Planes |

|
Geometry Applications, Triangles, Segment 1, Introduction | VIDEO: Geometry Applications: Triangles, 1
TopicTriangles DescriptionThis video introduces the importance of triangles in architecture and structural design. It highlights the use of triangles in structures like the Bank of China Tower in Hong Kong, noting their ability to provide strength and stability. The video focuses on the role of triangles in reinforcing skyscrapers to withstand strong winds, especially during typhoon seasons. Key vocabulary includes architectural support, triangular base, and stability. The video sets up a foundation for exploring how triangle properties are applied in real-world contexts. |
Applications of Triangles and Definition of a Triangle |