
Illustrative Math Alignment: Grade 8 Unit 1
Rigid Transformations and Congruence
Lesson 15: Adding the Angles in a Triangle
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|
|
Math Clip Art--Geometry Concepts--Kite Shape 16 | Math Clip Art--Kite Shape 16TopicGeometry Concepts DescriptionThis image illustrates a triangular-shaped kite. Geometrically, a kite is a quadrilateral with two pairs of adjacent equal-length sides and diagonals that intersect at right angles. The symmetry and unique properties of kite shapes make them interesting geometric figures. Kite shapes are used to explore properties such as symmetry, area, and perimeter. They are applicable in various mathematical contexts, including geometry and design. Teacher's Script: "Observe this kite. How is this a kite in the everyday sense? Is this a kite in the geometric sense?" |
Definition of a Polygon and Definition of a Triangle |
|
Math Clip Art--Geometry Concepts--Kite Shape 2 | Math Clip Art--Kite Shape 2TopicGeometry Concepts DescriptionThis image depicts another variation of a kite shape, in this case a circular shape. Geometrically, a kite is characterized by two pairs of adjacent equal-length sides and diagonals that intersect at right angles. This geometric configuration is symmetrical and unique. Kite shapes are used to explore geometric properties such as symmetry, area, and perimeter. They are applicable in various fields, including mathematics, design, and architecture. Teacher's Script: "Look at this kite. How is it a kite in the everyday sense? Is it a kite in a geometric sense?" |
Definition of a Circle, Definition of a Polygon and Definition of a Triangle |
|
Math Clip Art--Geometry Concepts--Kite Shape 3 | Math Clip Art--Kite Shape 3TopicGeometry Concepts DescriptionThis image illustrates a circular kite shape. Geometrically, a kite is a a quadrilateral with two pairs of adjacent equal-length sides and diagonals that intersect at right angles. The symmetry and unique properties of kite shapes make them interesting geometric figures. Kite shapes are used to explore properties such as symmetry, area, and perimeter. They are applicable in various mathematical contexts, including geometry and design. Teacher's Script: "Observe this kite. How is a kite in the everyday sense of the word? Is it a kite in the geometric sense?" |
Definition of a Circle, Definition of a Polygon and Definition of a Triangle |
|
Math Clip Art--Geometry Concepts--Kite Shape 4 | Math Clip Art--Kite Shape 4TopicGeometry Concepts DescriptionThis image features a hexagonal kite shape. Geometrically, a kite is a quadrilateral with two pairs of adjacent equal-length sides and diagonals that intersect at right angles. The symmetry and unique properties of kite shapes make them interesting geometric figures. Kite shapes are used to explore properties such as symmetry, area, and perimeter. They are applicable in various mathematical contexts, including geometry and design. Teacher's Script: "Examine this kite. How is it a kite in the everyday sense? Is it a kite in a geometric sense?" |
Definition of a Polygon and Definition of a Triangle |

|
Math Clip Art--Geometry Concepts--Kite Shape 5 | Math Clip Art--Kite Shape 5TopicGeometry Concepts DescriptionThis image depicts a hexagonal kite shape. Geometrically, a kite is characterized by two pairs of adjacent equal-length sides and diagonals that intersect at right angles. This geometric configuration is symmetrical and unique. Kite shapes are used to explore geometric properties such as symmetry, area, and perimeter. They are applicable in various fields, including mathematics, design, and architecture. Teacher's Script: "Look at this kite. How is this a kite in the everydays sense of the word? Is it a kite in the geometric sense?" |
Definition of a Polygon and Definition of a Triangle |

|
Math Clip Art--Geometry Concepts--Kite Shape 6 | Math Clip Art--Kite Shape 6TopicGeometry Concepts DescriptionThis image illustrates a hexagonal kite shape. Geometrically, a kite is a quadrilateral with two pairs of adjacent equal-length sides and diagonals that intersect at right angles. The symmetry and unique properties of kite shapes make them interesting geometric figures. Kite shapes are used to explore properties such as symmetry, area, and perimeter. They are applicable in various mathematical contexts, including geometry and design. Teacher's Script: "Observe this kite. How is it a kite in the everyday sense? Is it a kite in the geometric sense?" |
Definition of a Polygon and Definition of a Triangle |

|
Math Clip Art--Geometry Concepts--Kite Shape 7 | Math Clip Art--Kite Shape 7TopicGeometry Concepts DescriptionThis image features a kite shape, a quadrilateral with two pairs of adjacent equal-length sides and diagonals that intersect at right angles. The symmetry and unique properties of kite shapes make them interesting geometric figures. Kite shapes are used to explore properties such as symmetry, area, and perimeter. They are applicable in various mathematical contexts, including geometry and design. |
Definition of a Polygon and Definition of a Triangle |
|
Math Clip Art--Geometry Concepts--Kite Shape 8 | Math Clip Art--Kite Shape 8TopicGeometry Concepts DescriptionThis image depicts a kite shape, characterized by two pairs of adjacent equal-length sides and diagonals that intersect at right angles. This geometric configuration is symmetrical and unique. Kite shapes are used to explore geometric properties such as symmetry, area, and perimeter. They are applicable in various fields, including mathematics, design, and architecture. Teacher's Script: "Look at this kite shape. How do its symmetrical properties and intersecting diagonals help us understand its geometry? What are some mathematical concepts you can explore using kite shapes?" |
Definition of a Polygon and Definition of a Triangle |

|
Math Clip Art--Geometry Concepts--Kite Shape 9 | Math Clip Art--Kite Shape 9TopicGeometry Concepts DescriptionThis image illustrates a kite shape, a quadrilateral with two pairs of adjacent equal-length sides and diagonals that intersect at right angles. The symmetry and unique properties of kite shapes make them interesting geometric figures. Kite shapes are used to explore properties such as symmetry, area, and perimeter. They are applicable in various mathematical contexts, including geometry and design. |
Definition of a Polygon and Definition of a Triangle |

|
Math Clip Art--Geometry Concepts--Tessellation Pattern 1 | Math Clip Art--Tessellation Pattern 1TopicGeometry Concepts DescriptionThis image shows a tessellation pattern formed using basic shapes from pattern blocks. Pattern blocks typically include shapes such as equilateral triangles (green), squares (orange), rhombuses (blue), trapezoids (red), hexagons (yellow), and parallelograms (tan). These shapes fit together without gaps or overlaps to create a continuous pattern. Pattern block tessellations are excellent for exploring geometric concepts such as symmetry, transformations, and spatial reasoning. They provide a hands-on way to understand how shapes can be combined to cover a plane completely. |
Geometric Constructions with Polygons |

|
Math Clip Art--Geometry Concepts--Tessellation Pattern 2 | Math Clip Art--Tessellation Pattern 2TopicGeometry Concepts DescriptionThis image presents another tessellation pattern using basic shapes from pattern blocks. The basic pattern block shapes include equilateral triangles, squares, rhombuses, trapezoids, hexagons, and parallelograms. They are arranged to cover a plane without gaps or overlaps, demonstrating the principles of tessellation. Tessellations with pattern blocks help students explore geometric concepts such as symmetry, transformations, and tiling. They provide a visual and interactive way to understand how shapes can be combined to create complex patterns. |
Geometric Constructions with Polygons |
|
Math Clip Art--Geometry Concepts--Tessellation Pattern 3 | Math Clip Art--Tessellation Pattern 3TopicGeometry Concepts DescriptionThis image displays a tessellation pattern created with basic shapes from pattern blocks. The basic pattern block set includes equilateral triangles, squares, rhombuses, trapezoids, hexagons, and parallelograms. These shapes are arranged to tile a plane without gaps or overlaps. Using pattern blocks for tessellations allows students to explore geometric concepts such as symmetry, transformations, and spatial reasoning. They provide a hands-on approach to understanding how different shapes can be combined to form intricate patterns. |
Geometric Constructions with Polygons |

|
Math Clip Art--Geometry Concepts--Tessellation Pattern 4 | Math Clip Art--Tessellation Pattern 4TopicGeometry Concepts DescriptionThis image presents a tessellation pattern using basic shapes from pattern blocks. A basic pattern block set includes equilateral triangles, squares, rhombuses, trapezoids, hexagons, and parallelograms. These shapes are arranged to cover a plane without gaps or overlaps, illustrating the concept of tessellation. Tessellations with pattern blocks help students explore geometric concepts like symmetry, transformations, and tiling. They provide a visual and interactive way to understand how shapes can be combined to create complex patterns. |
Geometric Constructions with Polygons |
|
Math Clip Art--Triangles--Two Triangles Share Vertical Angles Congruent--Labeled | Math Clip Art--Triangles--Two Triangles Share Vertical Angles Congruent--Labeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |

|
Math Clip Art--Triangles--Two Triangles Share Vertical Angles Congruent--Unlabeled | Math Clip Art--Triangles--Two Triangles Share Vertical Angles Congruent--Unlabeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |

|
Math Clip Art--Triangles--Two Triangles Share Vertical Angles Non Congruent--Labeled | Math Clip Art--Triangles--Two Triangles Share Vertical Angles Non Congruent--Labeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |

|
Math Clip Art--Triangles--Two Triangles Share Vertical Angles Non Congruent--Unlabeled | Math Clip Art--Triangles--Two Triangles Share Vertical Angles Non Congruent--Unlabeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |
|
Math Clip Art--Triangles--Two Triangles Share Vertical Angles Similar--Labeled | Math Clip Art--Triangles--Two Triangles Share Vertical Angles Similar--Labeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |

|
Math Clip Art--Triangles--Two Triangles Share Vertical Angles Similar--Unlabeled | Math Clip Art--Triangles--Two Triangles Share Vertical Angles Similar--Unlabeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |
|
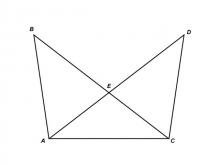
Math Clip Art--Triangles--Two Triangles with Common Base--Congruent--Labeled | Math Clip Art--Triangles--Two Triangles with Common Base--Congruent--Labeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |

|
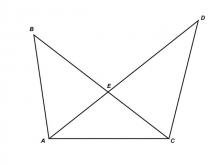
Math Clip Art--Triangles--Two Triangles with Common Base--Congruent--Unlabeled | Math Clip Art--Triangles--Two Triangles with Common Base--Congruent--Unlabeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |
|
Math Clip Art--Triangles--Two Triangles with Common Base--Non Congruent--Labeled | Math Clip Art--Triangles--Two Triangles with Common Base--Non Congruent--Labeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |

|
Math Clip Art--Triangles--Two Triangles with Common Base--Non Congruent--Unlabeled | Math Clip Art--Triangles--Two Triangles with Common Base--Non Congruent--Unlabeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |

|
Math Clip Art: Parallel Lines Cut by a Transversal 1 | Math Clip Art: Parallel Lines Cut by a Transversal 1TopicGeometry DescriptionThis math clip art image is part of a series that illustrates the properties of parallel lines cut by a transversal. In this particular image, we see two parallel lines without a transversal, serving as an introduction to the concept. The image is designed to help students visualize and understand the fundamental idea of parallel lines before exploring more complex relationships. |
Parallel Lines |
|
Math Clip Art: Parallel Lines Cut by a Transversal 10 | Math Clip Art: Parallel Lines Cut by a Transversal 10TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It showcases a fourth pair of corresponding angles, solidifying the concept that these angles are congruent when parallel lines are cut by a transversal. The image uses a distinct color coding to identify this final pair of corresponding angles, providing students with a comprehensive view of all possible corresponding angle pairs in this configuration. |
Parallel Lines |
|
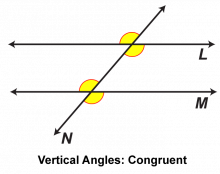
Math Clip Art: Parallel Lines Cut by a Transversal 11 | Math Clip Art: Parallel Lines Cut by a Transversal 11TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights vertical angles, demonstrating that these angles are congruent when any two lines intersect. The image uses color coding to clearly identify a pair of vertical angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
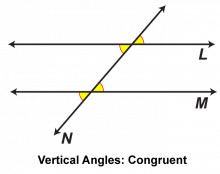
|
Math Clip Art: Parallel Lines Cut by a Transversal 12 | Math Clip Art: Parallel Lines Cut by a Transversal 12TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of vertical angles, reinforcing the concept that these angles are congruent when any two lines intersect. The image uses distinct color coding to identify this second pair of vertical angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
|
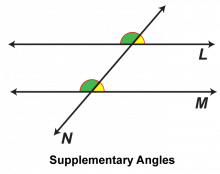
Math Clip Art: Parallel Lines Cut by a Transversal 13 | Math Clip Art: Parallel Lines Cut by a Transversal 13TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights supplementary angles, demonstrating that certain pairs of angles formed by parallel lines and a transversal are supplementary (their measures add up to 180°). The image uses color coding to clearly identify a pair of supplementary angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
|
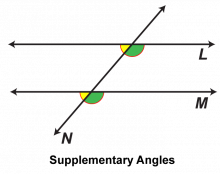
Math Clip Art: Parallel Lines Cut by a Transversal 14 | Math Clip Art: Parallel Lines Cut by a Transversal 14TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of supplementary angles, reinforcing the concept that certain pairs of angles formed by parallel lines and a transversal are supplementary. The image uses distinct color coding to identify this second pair of supplementary angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
|
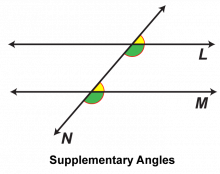
Math Clip Art: Parallel Lines Cut by a Transversal 15 | Math Clip Art: Parallel Lines Cut by a Transversal 15TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It showcases a third pair of supplementary angles, solidifying the concept that certain pairs of angles formed by parallel lines and a transversal are supplementary. The image uses a unique color coding to identify this pair of supplementary angles, providing students with multiple examples of this geometric relationship. |
Parallel Lines |

|
Math Clip Art: Parallel Lines Cut by a Transversal 16 | Math Clip Art: Parallel Lines Cut by a Transversal 16TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It showcases a fourth pair of supplementary angles, reinforcing the concept that certain pairs of angles formed by parallel lines and a transversal are supplementary (their measures add up to 180°). The image uses distinct color coding to identify this pair of supplementary angles, providing students with a comprehensive view of all possible supplementary angle pairs in this configuration. |
Parallel Lines |
|
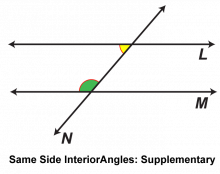
Math Clip Art: Parallel Lines Cut by a Transversal 17 | Math Clip Art: Parallel Lines Cut by a Transversal 17TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights same side interior angles, demonstrating that these angles are supplementary (their measures add up to 180°) when parallel lines are cut by a transversal. The image uses color coding to clearly identify a pair of same side interior angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
|
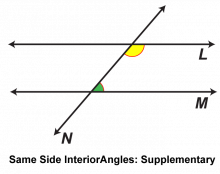
Math Clip Art: Parallel Lines Cut by a Transversal 18 | Math Clip Art: Parallel Lines Cut by a Transversal 18TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of same side interior angles, reinforcing the concept that these angles are supplementary when parallel lines are cut by a transversal. The image uses distinct color coding to identify this second pair of same side interior angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
|
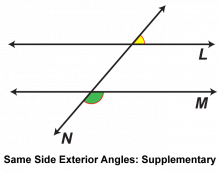
Math Clip Art: Parallel Lines Cut by a Transversal 19 | Math Clip Art: Parallel Lines Cut by a Transversal 19TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights same side exterior angles, demonstrating that these angles are supplementary (their measures add up to 180°) when parallel lines are cut by a transversal. The image uses color coding to clearly identify a pair of same side exterior angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
|
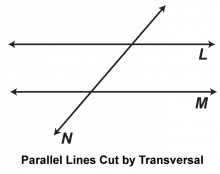
Math Clip Art: Parallel Lines Cut by a Transversal 2 | Math Clip Art: Parallel Lines Cut by a Transversal 2TopicGeometry DescriptionThis math clip art image is the second in a series illustrating the properties of parallel lines cut by a transversal. It shows two parallel lines intersected by a third line, known as a transversal. This visual representation is crucial for introducing students to the concept of parallel lines cut by a transversal and the various angle relationships that result from this configuration. |
Parallel Lines |

|
Math Clip Art: Parallel Lines Cut by a Transversal 20 | Math Clip Art: Parallel Lines Cut by a Transversal 20TopicGeometry DescriptionThis math clip art image is the final in a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of same side exterior angles, reinforcing the concept that these angles are supplementary when parallel lines are cut by a transversal. The image uses distinct color coding to identify this second pair of same side exterior angles, providing students with a comprehensive view of all angle relationships in this configuration. |
Parallel Lines |
|
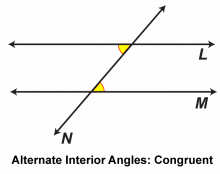
Math Clip Art: Parallel Lines Cut by a Transversal 3 | Math Clip Art: Parallel Lines Cut by a Transversal 3TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It specifically highlights alternate interior angles, showing that they are congruent when parallel lines are cut by a transversal. The image uses color coding to clearly identify the alternate interior angles, making it easier for students to visualize this important geometric relationship. |
Parallel Lines |
|
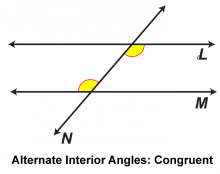
Math Clip Art: Parallel Lines Cut by a Transversal 4 | Math Clip Art: Parallel Lines Cut by a Transversal 4TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of alternate interior angles, reinforcing the concept that these angles are congruent when parallel lines are cut by a transversal. The image uses distinct color coding to identify this second pair of alternate interior angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
|
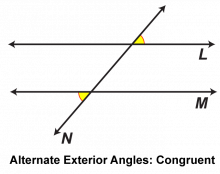
Math Clip Art: Parallel Lines Cut by a Transversal 5 | Math Clip Art: Parallel Lines Cut by a Transversal 5TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights alternate exterior angles, demonstrating that these angles are congruent when parallel lines are cut by a transversal. The image uses color coding to clearly identify the alternate exterior angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
|
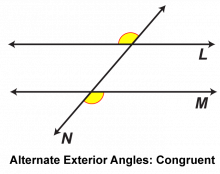
Math Clip Art: Parallel Lines Cut by a Transversal 6 | Math Clip Art: Parallel Lines Cut by a Transversal 6TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on alternate exterior angles, demonstrating that these angles are congruent when parallel lines are cut by a transversal. The image uses color coding to clearly identify another pair of alternate exterior angles, reinforcing the concept introduced in the previous image. |
Parallel Lines |
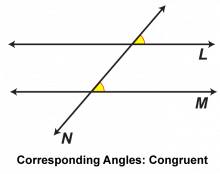
|
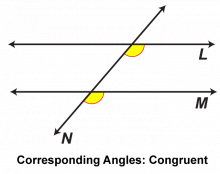
Math Clip Art: Parallel Lines Cut by a Transversal 7 | Math Clip Art: Parallel Lines Cut by a Transversal 7TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights corresponding angles, demonstrating that these angles are congruent when parallel lines are cut by a transversal. The image uses color coding to clearly identify a pair of corresponding angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |

|
Math Clip Art: Parallel Lines Cut by a Transversal 8 | Math Clip Art: Parallel Lines Cut by a Transversal 8TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of corresponding angles, reinforcing the concept that these angles are congruent when parallel lines are cut by a transversal. The image uses distinct color coding to identify this second pair of corresponding angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
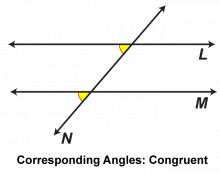
|
Math Clip Art: Parallel Lines Cut by a Transversal 9 | Math Clip Art: Parallel Lines Cut by a Transversal 9TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights yet another pair of corresponding angles, further reinforcing the concept that these angles are congruent when parallel lines are cut by a transversal. The image uses a unique color coding to identify this third pair of corresponding angles, providing students with multiple examples of this geometric relationship. |
Parallel Lines |
|
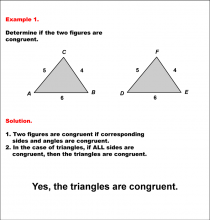
Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 1 | Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 1TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are congruent. Triangle ABC has sides 5, 4, and 6, while triangle DEF also has sides 5, 4, and 6. The SSS Postulate ensures that these triangles are congruent. Congruence of shapes is fundamental in geometry as it allows us to establish relationships between figures and understand properties of transformations. This example collection illustrates congruence by analyzing side lengths and angles to determine equivalence. |
Definition of a Triangle |
|
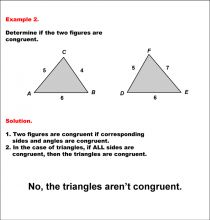
Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 2 | Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 2TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are congruent. Triangle ABC has sides 5, 4, and 6, while triangle DEF has sides 5, 7, and 6. Because corresponding sides are not all congruent, then the triangles are not congruent. Congruence of shapes is fundamental in geometry as it allows us to establish relationships between figures and understand properties of transformations. This example collection illustrates congruence by analyzing side lengths and angles to determine equivalence. |
Definition of a Triangle |
|
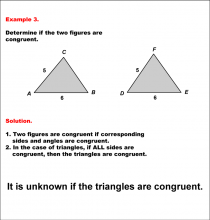
Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 3 | Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 3TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are congruent. Triangle ABC has sides 5 and 6, and triangle DEF has sides 5 and 6, but no information on the third side. As a result, there isn't enough information to know if they are congruent. Congruence of shapes is fundamental in geometry as it allows us to establish relationships between figures and understand properties of transformations. This example collection illustrates congruence by analyzing side lengths and angles to determine equivalence. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 4 | Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 4TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are congruent. Both triangles have two sides (5 and 6) and an included angle of 60°. As a results of the SAS Postulate, the triangles are congruent. Congruence of shapes is fundamental in geometry as it allows us to establish relationships between figures and understand properties of transformations. This example collection illustrates congruence by analyzing side lengths and angles to determine equivalence. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 5 | Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 5TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are congruent. Triangle ABC has sides 5 and 6 with an included angle of 60°, and triangle DEF has sides 5 and 6 with an included angle of 62°. Because corresponding angles are not congruent, then the triangles are not congruent. Congruence of shapes is fundamental in geometry as it allows us to establish relationships between figures and understand properties of transformations. This example collection illustrates congruence by analyzing side lengths and angles to determine equivalence. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 6 | Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 6TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are congruent. Both triangles have angles of 63°, 60°, and 57°, but no information on side lengths. As a result, we can't conclude if the triangles are congruent. Congruence of shapes is fundamental in geometry as it allows us to establish relationships between figures and understand properties of transformations. This example collection illustrates congruence by analyzing side lengths and angles to determine equivalence. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 7 | Math Example--Geometric Shapes--Analyzing Congruent Shapes--Example 7TopicGeometric Shapes DescriptionDetermine if two triangles on a grid are congruent. Both triangles appear identical in shape and orientation, positioned differently on the grid. Using the grid, you can see that corresponding sides are congruent. Therefore the triangles are congruent. Congruence of shapes is fundamental in geometry as it allows us to establish relationships between figures and understand properties of transformations. This example collection illustrates congruence by analyzing side lengths and angles to determine equivalence. |
Definition of a Triangle |