Illustrative Math Alignment: Grade 8 Unit 8

Topic

Rationals and Radicals

Definition

Rational numbers are numbers that can be expressed as a ratio of two integers, where the denominator is not zero.

Topic

Rationals and Radicals

Definition

Rationalizing a radical is the process of eliminating radicals from the denominator of a fraction by multiplying both the numerator and denominator by an appropriate factor.

Description

Rationalizing a Radical is an important technique in the study of Radical Numbers, Expressions, Equations, and Functions. This process involves eliminating radicals from the denominator of a fraction, which simplifies the expression and often makes it easier to work with or compare to other expressions. For example, to rationalize the denominator of 

$$\frac{1}{\sqrt{3}}$$

Topic

Rationals and Radicals

Definition

Rationalizing the denominator is the process of eliminating radicals or complex numbers from the denominator of a fraction by multiplying both the numerator and denominator by an appropriate factor.

Description

Rationalizing the Denominator is a crucial technique in the study of Radical Numbers, Expressions, Equations, and Functions. This process involves removing radicals or complex numbers from the denominator of a fraction, which simplifies the expression and often makes it easier to evaluate or compare with other expressions. For example, to rationalize the denominator of 

Topic

Rationals and Radicals

Definition

Simplifying a radical expression involves reducing the expression to its simplest form by factoring the radicand and removing any perfect square factors (for square roots) or perfect cube factors (for cube roots).

Description

Simplifying a Radical Expression is a fundamental skill in the study of Radical Numbers, Expressions, Equations, and Functions. This process involves reducing a radical expression to its simplest form, which often makes it easier to work with and understand. For example, simplifying 

$$\sqrt{18}$$

results in 

Topic

Rationals and Radicals

Definition

Simplifying a rational expression involves reducing the fraction to its lowest terms by factoring both the numerator and denominator and canceling common factors.

Description

Simplifying a Rational Expression is a crucial skill in the study of Rational Numbers, Expressions, Equations, and Functions. This process involves reducing a rational expression to its simplest form by factoring both the numerator and denominator and canceling common factors. For example, simplifying 

$$\frac{x^2 - 1}{x - 1}$$

results in x + 1 for 

Topic

Rationals and Radicals

Definition

The square root of a number is a value that, when multiplied by itself, gives the number. It is denoted by the radical symbol √.

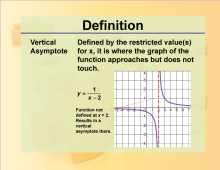
Topic

Rationals and Radicals

Definition

A vertical asymptote is a vertical line that the graph of a function approaches but never reaches as the input values get closer to a certain point.

Description

Vertical Asymptotes are a crucial concept in the study of Rational Numbers, Expressions, Equations, and Functions. They occur in rational functions when the denominator equals zero for certain input values, causing the function to approach infinity or negative infinity. For example, the function 

$$f(x) = \frac{1}{x-2}$$

The complete set of 28 examples that make up this set of tutorials.

Library of Instructional Resources

To see the complete library of Instructional Resources , click on this link.

In this Slide Show, do a hands-on geometry activity to measure π. Note: The download is a PPT file.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

This is part of a collection of math clip art images about different number systems. Included are integers, rational numbers, and real numbers.

Topic

Exponents

Description

Shows Example 1 with the expression 32. The solution explains how to simplify by multiplying 3 by itself according to the exponent. Example 1: Simplify 32. Multiply 3 by itself two times: 3•3 = 9.

Topic

Exponents

Description

Shows Example 10 with the expression (-3)3•54. The solution uses order of operations to evaluate each term before multiplying. Example 10: Simplify (-3)3•54. Evaluate each exponential term separately, then multiply: -27•625= -16,875.

Topic

Exponents

Description

Example 11 shows how to simplify (1/2)2. The image illustrates multiplying 1/2 by itself due to the exponent of 2. Example 11: Simplify (1/2)2. Multiply one-half two times. Solution: (1/2) * (1/2) = 1/4.

Topic

Exponents

Description

Example 12 shows how to simplify (1/3)4. The image illustrates multiplying 1/3 four times due to the exponent of 4. Example 12: Simplify (1/3)4. Multiply one-third four times. Solution: (1/3) * (1/3) * (1/3) * (1/3) = 1/81.

Topic

Exponents

Description

Example 13 shows how to simplify (-1/3)3. The image illustrates multiplying -1/3 three times due to the exponent of 3. Example 13: Simplify (-1/3)3. Multiply negative one-third three times. Solution: (-1/3) * (-1/3) * (-1/3) = -1/27.

Topic

Exponents

Description

Example 14 shows how to simplify 2-1. The image illustrates rewriting 2-1 as the reciprocal of 2 raised to the first power. Example 14: Simplify 2-1. Negative exponents are written as reciprocals. Solution: 2-1 = 1/2.

Topic

Exponents

Description

Example 15 shows how to simplify 2-2. The image illustrates rewriting 2-2 as the reciprocal of 2 raised to the second power. Example 15: Simplify 2-2. Negative exponents are written as reciprocals. Solution: 2-2 = 1/(22) = 1/4.

Topic

Exponents

Description

Shows Example 2 with the expression 43. The solution explains the simplification by multiplying 4 three times. Example 2: Simplify 43. Multiply 4 by itself three times: 4•4•4 = 64.

In general, the topic of exponents involves understanding how repeated multiplication can be expressed more compactly. The examples provided in this collection allow students to see the step-by-step breakdown of how to simplify various exponential expressions, which can include positive and negative bases, fractional bases, and negative exponents.

Topic

Exponents

Description

Shows Example 3 with the expression 54. The solution details multiplying 5 four times. Example 3: Simplify 54. Multiply 5 by itself four times: 5•5•5•5 = 625.

In general, the topic of exponents involves understanding how repeated multiplication can be expressed more compactly. The examples provided in this collection allow students to see the step-by-step breakdown of how to simplify various exponential expressions, which can include positive and negative bases, fractional bases, and negative exponents.

Topic

Exponents

Description

Shows Example 4 with the expression 106. The solution simplifies by multiplying 10 six times. Example 4: Simplify 106. Multiply 10 by itself six times: 10•10•10•10•10•10 = 1,000,000.

Topic

Exponents

Description

Shows Example 5 with the expression (-1)2. The solution explains the result by multiplying -1 by itself. Example 5: Simplify (-1)2. Multiply -1 by itself two times: (-1)•(-1) = 1.

Topic

Exponents

Description

Shows Example 6 with the expression (-5)3. The solution demonstrates multiplying -5 three times. Example 6: Simplify (_5)3. Multiply -5 by itself three times: (-5)•(-5)•(-5) = -125.

Topic

Exponents

Description

Shows Example 7 with the expression (-6)4. The solution explains multiplying -6 by itself four times. Example 7: Simplify (-6)4. Multiply -6 by itself four times: (-6)•(-6)•(-6)•(-6) = 1296.

Topic

Exponents

Description

Shows Example 8 with the expression 23•32. The solution demonstrates using order of operations to simplify each term separately, then multiply. Example 8: Simplify 23•32. Evaluate each exponential term separately, then multiply: 8•9 = 72.

Topic

Exponents

Description

Shows Example 9 with the expression (-1)2•43. The solution explains simplifying each term individually before multiplying. Example 9: Simplify (-1)2•43. Evaluate each exponential term separately, then multiply: 1•64 = 64.

Topic

Numerical Expressions

Description

This example demonstrates the division of two positive integers: 12 divided by 3. The solution shows that when dividing one integer by another, the result is a rational number. In this case, with two positive integers, the quotient is positive. The calculation is presented as 12 ÷ 3 = 12 / 3 = 4.

Topic

Numerical Expressions

Description

This example demonstrates the division of three integers: (-144) ÷ 12 ÷ (-2). The solution shows that dividing three integers, two negative and one positive, results in a positive quotient. The calculation is presented step-by-step: (-144) ÷ 12 ÷ (-2) = -12 / -2 = 6.

Topic

Numerical Expressions

Description

This example demonstrates the division of three integers: (-150) ÷ (-5) ÷ 3. The solution shows that dividing three integers, two negative and one positive, results in a positive quotient. The calculation is presented step-by-step: (-150) ÷ (-5) ÷ 3 = 30 / 3 = 10.

Topic

Numerical Expressions

Description

This example illustrates the division of three negative integers: -108 ÷ (-2) ÷ (-6). The solution demonstrates that dividing three negative integers results in a negative quotient. The calculation is presented step-by-step: (-108 / -2) / -6 = 54 / -6 = -9.

Topic

Numerical Expressions

Description

This example demonstrates the division of two positive integers: 12 ÷ 5. The solution shows that dividing two positive integers results in a positive quotient, which in this case is a mixed number. The calculation is presented as: 12 / 5 = 2 2/5.

Topic

Numerical Expressions

Description

This example illustrates the division of a positive integer by a negative integer: 14 ÷ (-6). The solution demonstrates that dividing a positive by a negative results in a negative quotient, which in this case is a mixed number. The calculation is presented as: 14 / -6 = -2 1/3.

Topic

Numerical Expressions

Description

This example demonstrates the division of a negative integer by a positive integer: -18 ÷ 4. The solution shows that dividing a negative by a positive results in a negative quotient, which in this case is a fraction. The calculation is presented as: -18 / 4 = -9/2 = -4 1/2.

Topic

Numerical Expressions

Description

This example illustrates the division of two negative integers: (-9) ÷ (-6). The solution demonstrates that dividing two negative integers results in a positive fraction. The calculation is presented step-by-step: 

(-9) ÷ (-6) = -9 / -6 = -3 / -2 = 1 1/2.

Topic

Numerical Expressions

Description

This example demonstrates the division of three positive integers: 20 ÷ 2 ÷ 4. The solution shows that dividing three positive integers results in a positive mixed number quotient. The calculation is presented step-by-step: 

20 ÷ 2 ÷ 4 = 20 / 2 / 4 = 10 / 4 = 2 1/2.

Topic

Numerical Expressions

Description

This example illustrates the division of three integers: 48 ÷ 6 ÷ (-3). The solution demonstrates that dividing two positive integers and one negative integer results in a negative mixed number quotient. The calculation is presented step-by-step: 

48 ÷ 6 ÷ (-3) = 48 / 6 / (-3) = 8 / (-3) = -2 2/3.

Topic

Numerical Expressions

Description

This example demonstrates the division of three integers: 24 ÷ (-6) ÷ 2. The solution shows that dividing two positive integers and one negative integer results in a negative quotient. The calculation is presented step-by-step: 

24 ÷ (-6) ÷ 2 = 24 / (-6) / (2) = -4 / 2 = -2.

Topic

Numerical Expressions

Description

This example illustrates the division of a positive integer by a negative integer: 14 divided by -7. The solution demonstrates that when dividing one integer by another, the result is a rational number. In this case, when dividing a positive integer by a negative integer, the quotient is negative. The calculation is presented as 14 ÷ (-7) = 14 / -7 = -2.

Topic

Numerical Expressions

Description

This example demonstrates the division of three integers: (-72) / 2 / 5. The solution shows that dividing a negative integer by two positive integers results in a negative mixed number quotient. The calculation is presented step-by-step: 

(-72) / 2 / 5 = -72 / (2 * 5) = -36 / 5 = -7 1/5.

Topic

Numerical Expressions

Description

This example demonstrates the division of three integers: 125 / (-25) / (-2). The solution shows that dividing a positive integer by two negative integers results in a positive mixed number quotient. The calculation is presented step-by-step: 

125 / (-25) / (-2) = 125 / (25 * -2) = -5 / -2 = 2 1/2.

Topic

Numerical Expressions

Description

This example illustrates the division of three integers: (-144) / 12 / (-5). The solution demonstrates that dividing a negative integer by a positive integer and then by a negative integer results in a positive fraction. The calculation is presented step-by-step: 

(-144) / 12 / (-5) = -144 / (12 * -5) = -12 / -5 = 2 2/5.