IXL Ad

Illustrative Math-Media4Math Alignment

 

 

Illustrative Math Alignment: Grade 6 Unit 8

Data Sets and Distributions

Lesson 4: Dot Plots

Use the following Media4Math resources with this Illustrative Math lesson.

Thumbnail Image Title Body Curriculum Nodes
Definition--Measures of Central Tendency--Median of an Even Data Set Definition--Measures of Central Tendency--Median of an Even Data Set Median of an Even Data Set

Topic

Statistics

Definition

The median of an even data set is the mean of two of the terms.

Description

The Median is the middle term of a data set. If the data set consists of an even number of terms, then the Median won't be one of ther terms in the set. In such a case the Median is the Mean of the two middle terms. 

 Data Analysis
Definition--Measures of Central Tendency--Discrete Data Definition--Measures of Central Tendency--Discrete Data Discrete Data

Topic

Statistics

Definition

Discrete data consists of countable values, often represented by whole numbers.

Description

Discrete data is commonly used in situations where data points are distinct and separate, such as the number of students in a class or the number of cars in a parking lot. It is crucial for fields like computer science, where discrete structures and algorithms are fundamental. In mathematics, discrete data is used in probability theory and combinatorics, helping to solve problems involving permutations and combinations.

 Data Analysis
Definition--Measures of Central Tendency--Population Mean Definition--Measures of Central Tendency--Population Mean Population Mean

Topic

Statistics

Definition

The population mean is a measure of central tendency that provides an average representation of a set of data.

Description

The Population Mean is an important concept in statistics, used to summarize data effectively. It is meant to represent the mean for a given statistic for an entire population. For example, the mean length of a salmon.

 Data Analysis
Definition--Measures of Central Tendency--Variance Definition--Measures of Central Tendency--Variance Variance

Topic

Statistics

Definition

Variance is a measure of the dispersion of a set of values, calculated as the average of the squared deviations from the mean.

Description

Variance quantifies the degree of spread in a data set, providing insight into the variability of data points around the mean. It is a fundamental concept in statistics, used in fields such as finance, research, and engineering to assess risk and variability. A high variance indicates greater dispersion, while a low variance suggests that data points are closer to the mean.

 Data Analysis
Definition--Measures of Central Tendency--Continuous Data Definition--Measures of Central Tendency--Continuous Data Continuous Data

Topic

Statistics

Definition

Continuous data is numerical data that can take any value within a range.

Description

Continuous data is vital for representing measurements such as height, weight, and temperature, which can assume an infinite number of values within a given range. In real-world applications, continuous data is used in fields like engineering, physics, and economics to model and predict outcomes. Understanding continuous data is essential for performing calculations involving integrals and derivatives in calculus.

 Data Analysis
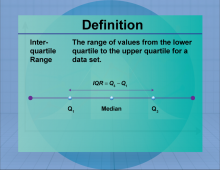
Definition--Measures of Central Tendency--Interquartile Range Definition--Measures of Central Tendency--Interquartile Range Interquartile Range

Topic

Statistics

Definition

The interquartile range (IQR) is the range between the first and third quartiles, representing the middle 50% of a data set.

Description

The IQR is a measure of statistical dispersion, indicating the spread of the central portion of a data set. It is particularly useful for identifying outliers and understanding the variability of data. In real-world applications, the IQR is used in finance to assess investment risks and in quality control to monitor process stability.

 Data Analysis
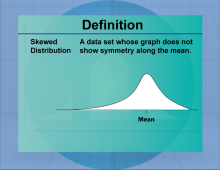
Definition--Measures of Central Tendency--Skewed Distribution Definition--Measures of Central Tendency--Skewed Distribution Skewed Distribution

Topic

Statistics

Definition

A skewed distribution is a probability distribution that is not symmetric, with data tending to cluster more on one side.

Description

Skewed distributions occur when data is not evenly distributed around the mean, resulting in a longer tail on one side. Skewness can be positive (right-skewed) or negative (left-skewed), affecting the interpretation of data and statistical measures such as the mean and median. Skewed distributions are common in real-world data, such as income levels and test scores, where extreme values can influence the overall distribution.

 Data Analysis
Definition--Measures of Central Tendency--Upper Quartile Definition--Measures of Central Tendency--Upper Quartile Upper Quartile

Topic

Statistics

Definition

The upper quartile (Q3) is the median of the upper half of a data set, representing the 75th percentile.

Description

The upper quartile is a measure of position that indicates the value below which 75% of the data falls. It is used in conjunction with other quartiles to understand the distribution and spread of data. In real-world applications, the upper quartile is used in finance to assess investment performance and in education to evaluate student achievement levels.

 Data Analysis
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 5 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 5 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 5

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 4 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 4 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 4

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 1 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 1 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 1

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
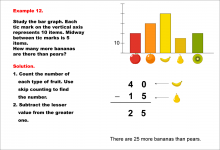
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 12 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 12 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 12

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 8 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 8 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 8

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 7 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 7 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 7

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 3 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 3 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 3

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
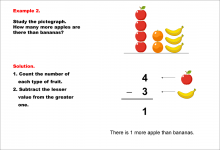
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 2 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 2 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 2

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 11 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 11 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 11

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
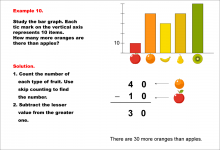
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 10 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 10 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 10

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 6 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 6 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 6

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
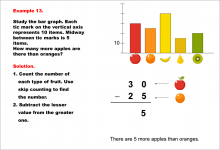
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 13 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 13 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 13

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 9 Math Example--Charts, Graphs, and Plots--Reading and Interpreting Scaled Graphs--Example 9 Math Example--Charts, Graphs, and Plots-- Reading and Interpreting Scaled Graphs--Example 9

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Subtraction Facts to 100 and Data Analysis
Closed Captioned Video: Algebra Nspirations: Data Analysis and Probability Closed Captioned Video: Algebra Nspirations: Data Analysis and Probability Closed Captioned Video: Algebra Nspirations: Data Analysis and Probability

What are the two meanings of statistics? What does it really mean that an event has a 50% probability of occurring? Why are data analysis and probability always taught together? Written and hosted by internationally acclaimed math educator Dr. Monica Neagoy, this video answers these questions and addresses fundamental concepts such as the law of large numbers and the notion of regression analysis. Both engaging investigations are based on true stories and real data, utilize different Nspire applications, and model the seamless connection among various problem representations. Concepts explored: statistics, data analysis, regression analysis.

 Data Analysis and Data Gathering
Closed Captioned Video: Algebra Nspirations: Data Analysis and Probability, Segment 1 Closed Captioned Video: Algebra Nspirations: Data Analysis and Probability, 1 Closed Captioned Video: Algebra Nspirations: Data Analysis and Probability, Segment 1

In this Investigation we explore uncertainty and randomness. This video is Segment 1 of a 4 segment series related to Data Analysis and Probability. Segments 1 and 2 are grouped together.

 Data Analysis and Data Gathering
Closed Captioned Video: Algebra Nspirations: Data Analysis and Probability, Segment 3 Closed Captioned Video: Algebra Nspirations: Data Analysis and Probability, 3 Closed Captioned Video: Algebra Nspirations: Data Analysis and Probability, Segment 3

In this Investigation we look at real-world data involving endangered wolf populations. This video is Segment 3 of a 4 segment series related to Data Analysis and Probability. Segments 3 and 4 are grouped together.

 Data Analysis and Data Gathering
Closed Captioned Video: Algebra Applications: Data Analysis, Segment 4: What is an Adjustable Rate Mortgage? Closed Captioned Video: Algebra Applications: Data Analysis, 4 Closed Captioned Video: Algebra Applications: Data Analysis, Segment 4: What is an Adjustable Rate Mortgage?

Another factor in the mortgage crisis was the use of adjustable rate mortgages. Students run a number of scenarios to test adjustable rate mortgages, while also taking into account the state of the housing market during the time of the mortgage crisis.

 Data Analysis and Data Gathering
Closed Captioned Video: Algebra Applications: Data Analysis, Segment 1: Introduction Closed Captioned Video: Algebra Applications: Data Analysis, 1 Closed Captioned Video: Algebra Applications: Data Analysis, Segment 1: Introduction

In this introductory segment students learn about the mortage crisis of 2008. In the process they get a brief introduction to what a mortgage is.

 Data Analysis and Data Gathering
Closed Captioned Video: Algebra Applications: Data Analysis, Segment 3: What Is a What is a Subprime Mortgage? Closed Captioned Video: Algebra Applications: Data Analysis, 3 Closed Captioned Video: Algebra Applications: Data Analysis, Segment 3: What Is a What is a Subprime Mortgage?

Having learned the general features of a mortgage, students learn the specifics of a subprime mortgage. With this comes the notion of a credit score, and with credit scores come the probabilities for a loan default. Students use the amortization table to run probability simulations to determine possible loan defaults on subprime mortgages.

 Data Analysis and Data Gathering
Closed Captioned Video: Algebra Applications: Data Analysis, Segment 2: What Is a Mortgage? Closed Captioned Video: Algebra Applications: Data Analysis, 2 Closed Captioned Video: Algebra Applications: Data Analysis, Segment 2: What Is a Mortgage?

The time value of money is at the basis of all loans. Students learn about the key factors that determine monthly mortgage payments and use the TI-Nspire to create an amortization table. This table is used throughout the rest of the program to explore different scenarios.

 Data Analysis and Data Gathering
Closed Captioned Video: Algebra Applications: Linear Functions, Segment 3: Oil Exploration Closed Captioned Video: Algebra Applications: Linear Functions, 3 Closed Captioned Video: Algebra Applications: Linear Functions, Segment 3: Oil Exploration

Linear Expressions, Equations, and Functions

Linear Exp 		Special Functions and Applications of Linear Functions
Closed Captioned Video: Algebra Applications: Data Analysis Closed Captioned Video: Algebra Applications: Data Analysis Closed Captioned Video: Algebra Applications: Data Analysis

In this episode of Algebra Applications, students explore the dramatic events of 2008 related to the mortgage crisis. Brought about principally through mortgage defaults, the effect on the overall economy was severe. Yet, this situation offers an ideal case study for the exploration of key concepts in data analysis and probability. By exploring these questions students get a front row seat to the historical events of the world's largest economy.

 Data Analysis and Data Gathering
Closed Captioned Video: Ratios and Rates: Rates from Data Closed Captioned Video: Ratios and Rates: Rates from Data Closed Captioned Video: Ratios and Rates: Rates from Data

Video Tutorial: Ratios and Rates: Rates from Data. In this video, we look at linear data sets that can be used to find the rate of change.

 Ratios and Rates
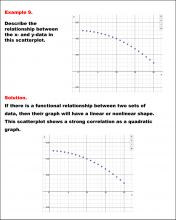
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 9 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 9 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 9

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
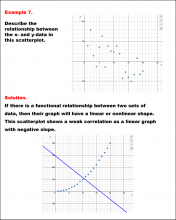
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 2 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 2 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 2

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 7 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 7 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 7

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
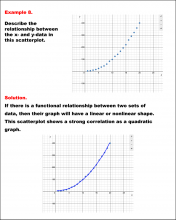
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 8 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 8 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 8

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 6 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 6 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 6

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
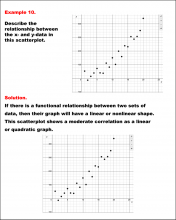
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 10 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 10 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 10

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
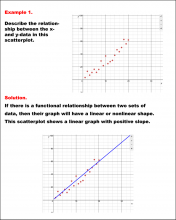
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 1 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 1 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 1

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
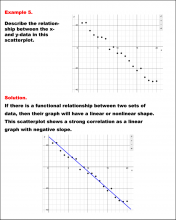
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 5 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 5 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 5

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
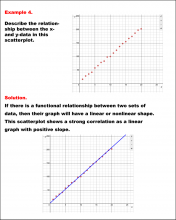
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 4 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 4 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 4

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
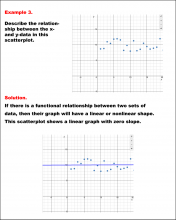
Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 3 Math Example--Charts, Graphs, and Plots--Analyzing Scatterplots: Example 3 Math Example--Charts, Graphs, and Plots-- Analyzing Scatterplots: Example 3

In this set of math examples, analyze the behavior of different scatterplots. This includes linear and quadratic models.

 Data Analysis
Definition--Measures of Central Tendency--Probability Distribution Definition--Measures of Central Tendency--Probability Distribution Probability Distribution

Topic

Statistics

Definition

A probability distribution describes how the values of a random variable are distributed.

Description

Probability distributions are fundamental in statistics, providing a mathematical function that gives the probabilities of occurrence of different possible outcomes for an experiment. They are used in various fields such as finance, science, and engineering to model uncertainty and variability. For instance, the normal distribution is a common probability distribution that describes many natural phenomena.

 Data Analysis
Definition--Measures of Central Tendency--Outlier Definition--Measures of Central Tendency--Outlier Outlier

Topic

Statistics

Definition

The outlier is is an extreme value for a data set.

Description

The Outlier is an important concept in statistics. While it doesn't represent the average data set, it does set the range of extreme values in the data set. An outlier can be extremely large or small. 

In mathematics education, understanding outlier is crucial as it lays the foundation for more advanced statistical concepts. It allows students to grasp the significance of data analysis and interpretation. In classes, students often perform exercises calculating the mean of sets, which enhances their understanding of averaging techniques.

 Data Analysis
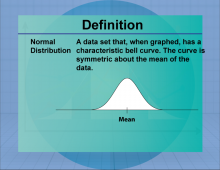
Definition--Measures of Central Tendency--Normal Distribution Definition--Measures of Central Tendency--Normal Distribution Normal Distribution

Topic

Statistics

Definition

The normal distribution is a measure of central tendency that provides an average representation of a set of data.

Description

The Normal Distribution is an important concept in statistics, used to summarize data effectively. In real-world applications, the Normal Distribution helps to interpret data distributions and is widely used in areas such as economics, social sciences, and research.

 Data Analysis
Definition--Measures of Central Tendency--Categorical Data Definition--Measures of Central Tendency--Categorical Data Categorical Data

Topic

Statistics

Definition

Categorical data refers to data that can be divided into specific categories or groups.

Description

Categorical data is essential for organizing and analyzing information that falls into distinct categories, such as gender, race, or product type. This type of data is often used in market research, social sciences, and public health studies to identify patterns and relationships between groups. In mathematics, understanding categorical data is crucial for interpreting bar charts, pie charts, and frequency tables.

 Data Analysis
Definition--Measures of Central Tendency Definition--Measures of Central Tendency Measures of Central Tendency

Topic

Statistics

Definition

The measures of central tendency is a measure of central tendency that provides an average representation of a set of data.

Description

The Measures of Central Tendency is an important concept in statistics, used to summarize data effectively.

In real-world applications, the Measures of Central Tendency helps to interpret data distributions and is widely used in areas such as economics, social sciences, and research.

For example, if a data set consists of the values 2, 3, and 10, the mean is calculated as (2 + 3 + 10)/3 = 5.

 Data Analysis
Worksheet: The Language of Math: Multiplication Equations, Worksheet 2 Worksheet: The Language of Math: Multiplication Equations, Worksheet 2 Worksheet: The Language of Math: Multiplication Equations, Worksheet 2

This is part of a collection of math worksheets on the topic of the language of math in which students translate verbal expressions into numerical expressions and then perform the calculations.

To see the complete worksheet collection on this topic, click on this link. Note: The download is a PDF file.

Related Resources

To see additional resources on this topic, click on the Related Resources tab.

Worksheet Library

To see the complete collection of Worksheets, click on this link. 		Numerical and Algebraic Expressions
Worksheet: The Language of Math: Multiplication Equations, Worksheet 4 Worksheet: The Language of Math: Multiplication Equations, Worksheet 4 Worksheet: The Language of Math: Multiplication Equations, Worksheet 4

This is part of a collection of math worksheets on the topic of the language of math in which students translate verbal expressions into numerical expressions and then perform the calculations.

To see the complete worksheet collection on this topic, click on this link. Note: The download is a PDF file.

Related Resources

To see additional resources on this topic, click on the Related Resources tab.

Worksheet Library

To see the complete collection of Worksheets, click on this link. 		Numerical and Algebraic Expressions
Worksheet: The Language of Math: Multiplication Equations, Worksheet 5 Worksheet: The Language of Math: Multiplication Equations, Worksheet 5 Worksheet: The Language of Math: Multiplication Equations, Worksheet 5

This is part of a collection of math worksheets on the topic of the language of math in which students translate verbal expressions into numerical expressions and then perform the calculations.

To see the complete worksheet collection on this topic, click on this link. Note: The download is a PDF file.

Related Resources

To see additional resources on this topic, click on the Related Resources tab.

Worksheet Library

To see the complete collection of Worksheets, click on this link. 		Numerical and Algebraic Expressions
Worksheet: The Language of Math: Multiplication Equations, Worksheet 1 Worksheet: The Language of Math: Multiplication Equations, Worksheet 1 Worksheet: The Language of Math: Multiplication Equations, Worksheet 1

This is part of a collection of math worksheets on the topic of the language of math in which students translate verbal expressions into numerical expressions and then perform the calculations.

To see the complete worksheet collection on this topic, click on this link. Note: The download is a PDF file.

Related Resources

To see additional resources on this topic, click on the Related Resources tab.

Worksheet Library

To see the complete collection of Worksheets, click on this link. 		Numerical and Algebraic Expressions