
Illustrative Math Alignment: Grade 6 Unit 9
Putting it All Together
Lesson 4: How Do We Choose?
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topics |
|---|---|---|---|
|
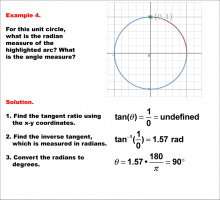
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 4 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 4TopicAngles DescriptionThe image shows a unit circle with a highlighted arc and a point labeled (0, 1) at the top of the circle, representing an undefined tangent ratio as it lies on the y-axis directly above the origin. Solution steps are listed on the right side. Example 4: The tangent ratio is undefined because tan(Θ) = 1 / 0, but tan-1(undefined) gives an angle of Θ = 1.57 radians, or 90°. |
Trigonometric Functions |
|
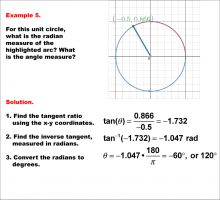
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 5 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 5TopicAngles DescriptionThis image shows a unit circle with a highlighted arc in the second quadrant. The point (-0.5, 0.866) is marked on the circle. The caption explains how to find the radian measure of the highlighted arc using trigonometric ratios. The arc is in red, and the radius is drawn. Example 5: The tangent ratio is calculated using tan(Θ) = 0.866 / -0.5 = -1.732. The inverse tangent is found as tan-1(-1.732) = -1.047 radians, and converting this to degrees gives Θ = -60° or 120°. |
Trigonometric Functions |
|
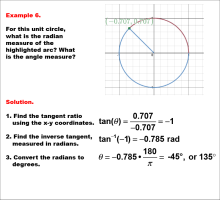
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 6 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 6TopicAngles DescriptionThis image shows a unit circle with a highlighted arc in the second quadrant. The point (-0.707, 0.707) is marked on the circle. The caption explains how to find the radian measure of the highlighted arc using trigonometric ratios. The arc is in red, and the radius is drawn. Example 6: The tangent ratio is calculated using tan(Θ) = 0.707 / -0.707 = -1. The inverse tangent is found as tan-1(-1) = -0.785 radians, and converting this to degrees gives Θ = -45 degrees or 135 degrees. |
Trigonometric Functions |
|
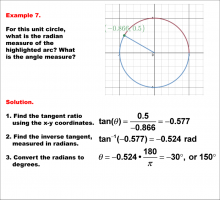
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 7 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 7TopicAngles DescriptionThis image shows a unit circle with a highlighted arc in the second quadrant. The point (-0.866, 0.5) is marked on the circle. The caption explains how to find the radian measure of the highlighted arc using trigonometric ratios. The arc is in red, and the radius is drawn. Example 7: The tangent ratio is calculated using tan(Θ) = 0.5 / -0.866 = -0.577. The inverse tangent is found as tan-1(-0.577) = -0.524 radians, and converting this to degrees gives Θ = -30° or 150°. |
Trigonometric Functions |

|
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 8 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 8TopicAngles DescriptionThis image shows a unit circle with a highlighted arc along the negative x-axis (180 degrees). The point (-1, 0) is marked on the circle at this position on the unit circle's circumference, representing an angle of either 0 or 180 degrees depending on direction. Example 8: The tangent ratio is calculated using tan(Θ) = 0 / 1 = 0. The inverse tangent is found as tan-1(0) = 0 radians, and converting this to degrees gives Θ = 0° or 180° depending on direction along the unit circle's circumference from origin to point (-1, 0). |
Trigonometric Functions |
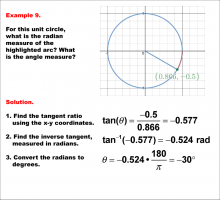
|
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 9 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 9TopicAngles DescriptionThe image shows a unit circle with a highlighted arc in the fourth quadrant. The coordinates of the point on the circle are (0.866, -0.5). The tangent ratio is calculated, followed by the inverse tangent to find the angle in radians and degrees. Example 9: The x-y coordinates are (0.866, -0.5). The tangent ratio is calculated as tan(Θ) = -0.5 / 0.866 = -0.577. The inverse tangent gives Θ = -0.524 radians, which is converted to degrees as Θ = -30°. |
Trigonometric Functions |
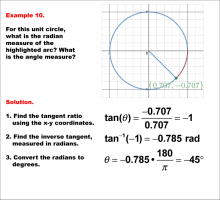
|
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 10 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 10TopicAngles DescriptionThis image displays a unit circle with a highlighted arc in the fourth quadrant. The coordinates of the point on the circle are (0.707, -0.707). The tangent ratio is calculated, followed by the inverse tangent to find the angle in radians and degrees. Example 10: The x-y coordinates are (0.707, -0.707). The tangent ratio is calculated as tan(Θ) = -0.707 / 0.707 = -1. The inverse tangent gives Θ = -0.785 radians, which is converted to degrees as Θ = -45°. |
Trigonometric Functions |
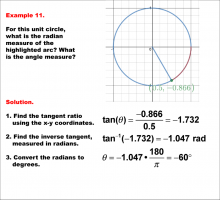
|
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 11 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 11TopicAngles DescriptionThis image shows a unit circle with a highlighted arc in the fourth quadrant. The coordinates of the point on the circle are (0.5, -0.866). The tangent ratio is calculated, followed by the inverse tangent to find the angle in radians and degrees. Example 11: The x-y coordinates are (0.5, -0.866). The tangent ratio is calculated as tan(Θ) = -0.866 / 0.5 = -1.732. The inverse tangent gives Θ = -1.047 radians, which is converted to degrees as Θ = -60°. |
Trigonometric Functions |
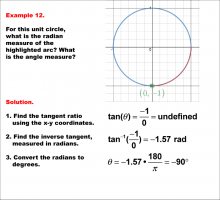
|
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 12 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 12TopicAngles DescriptionThis image displays a unit circle with a highlighted arc along the negative y-axis (third quadrant). The coordinates of the point on the circle are (0, -1). The tangent ratio is undefined due to division by zero, but the angle is found using radians/degrees. Example 12: The x-y coordinates are (0, -1). Since tan(Θ) = -1 / 0 is undefined, we use the known value for this position on the unit circle: Θ = -1.57 radians, which is converted to degrees as Θ = -90°. |
Trigonometric Functions |
|
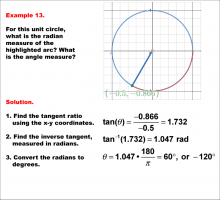
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 13 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 13TopicAngles DescriptionThe image shows a unit circle with a point at (-0.5, -0.866), and the highlighted arc is in the third quadrant. The tangent ratio is calculated using these coordinates. The solution involves finding the inverse tangent and converting radians to degrees. Example 13: The tangent ratio is calculated as tan(Θ) = -0.866 / -0.5 = 1.732. The inverse tangent is tan-1(1.732) = 1.047 radians. Converting this to degrees gives Θ = 60° or -120°. |
Trigonometric Functions |
|
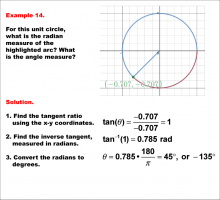
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 14 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 14TopicAngles DescriptionThis image shows a unit circle with a point at (-0.707, -0.707) in the third quadrant, and the highlighted arc is shown in red. The tangent ratio is calculated using these coordinates to find the angle in both radians and degrees. Example 14: The tangent ratio is tan(Θ) = -0.707 / -0.707 = 1, leading to an inverse tangent of tan-1(1) = 0.785 radians. Converting this to degrees gives Θ = 45° or -135°. |
Trigonometric Functions |

|
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 15 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 15TopicAngles DescriptionThis image shows a unit circle with a point at (-0.866, -0.5), and the highlighted arc in red appears in the third quadrant of the circle. The tangent ratio is calculated using these coordinates, followed by finding the angle in both radians and degrees. Example 15: The tangent ratio is tan(Θ) = -0.5 / -0.866 = 0.577, leading to an inverse tangent of tan-1(0.577) = 0.524 radians. Converting this to degrees gives Θ = 30° or -150°. |
Trigonometric Functions |
|
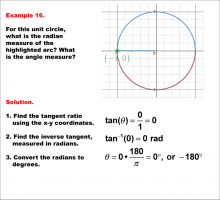
Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 16 | Math Example--Angle Concepts--Using Trig Ratios to Measure Radians--Example 16TopicAngles DescriptionThis image shows a unit circle with a point at (-1, 0), located on the negative x-axis, and the highlighted arc covers half of the circle (180¡). The tangent ratio is calculated using these coordinates, followed by finding the angle in both radians and degrees. Example 16: The tangent ratio is tan(Θ) = 0 / 1 = 0, leading to an inverse tangent of tan-1(0) = 0 radians. Converting this to degrees gives Θ = 0° or -180°. |
Trigonometric Functions |

|
Definition--Rationals and Radicals--Irrational Number | Irrational NumberTopicRationals and Radicals DefinitionAn irrational number is a number that cannot be expressed as a ratio of two integers. Its decimal form is non-repeating and non-terminating. |
Rational Expressions |

|
Math Example--Rational Concepts--Rational vs Irrational--Example 1 | Math Example--Rational Concepts--Rational vs Irrational--Example 1
This is part of a collection of math examples that focus on rational number concepts. This includes rational numbers, expressions, and functions. |
Rational Expressions |

|
Math Example--Rational Concepts--Rational vs Irrational--Example 2 | Math Example--Rational Concepts--Rational vs Irrational--Example 2
This is part of a collection of math examples that focus on rational number concepts. This includes rational numbers, expressions, and functions. |
Rational Expressions |

|
Math Example--Rational Concepts--Rational vs Irrational--Example 3 | Math Example--Rational Concepts--Rational vs Irrational--Example 3
This is part of a collection of math examples that focus on rational number concepts. This includes rational numbers, expressions, and functions. |
Rational Expressions |

|
Math Example--Rational Concepts--Rational vs Irrational--Example 4 | Math Example--Rational Concepts--Rational vs Irrational--Example 4
This is part of a collection of math examples that focus on rational number concepts. This includes rational numbers, expressions, and functions. |
Rational Expressions |

|
Math Example--Exponential Concepts--Rational Exponents--Example 1 | Math Example--Exponential Concepts--Rational Exponents--Example 1TopicExponents DescriptionThe image shows Example 1 of simplifying a rational exponent expression. The expression is 251/2. The solution involves converting the exponent to a square root. Solution: Convert the rational exponent to an nth root. 251/2 = √(25) = 5. This example involves simplifying expressions with rational exponents, providing a concrete application of exponent properties and the concept of roots. The method breaks down complex expressions into simpler terms, illustrating step-by-step how to handle rational exponents. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Rational Exponents--Example 2 | Math Example--Exponential Concepts--Rational Exponents--Example 2TopicExponents DescriptionThe image shows Example 2 of simplifying a rational exponent expression. The expression is 1251/3. The solution involves converting the exponent to a cube root. Example 2. Simplify the expression: 1251/3. Solution: Convert the rational exponent to an nth root. 1251/3 = ∛125 = 5. This example involves simplifying expressions with rational exponents, providing a concrete application of exponent properties and the concept of roots. The method breaks down complex expressions into simpler terms, illustrating step-by-step how to handle rational exponents. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Rational Exponents--Example 3 | Math Example--Exponential Concepts--Rational Exponents--Example 3TopicExponents DescriptionThe image shows Example 3 of simplifying a rational exponent expression. The expression is 161/4. The solution involves converting the exponent to a fourth root. Solution: Convert the rational exponent to an nth root. 161/4 = 4√16 = 2. This example involves simplifying expressions with rational exponents, providing a concrete application of exponent properties and the concept of roots. The method breaks down complex expressions into simpler terms, illustrating step-by-step how to handle rational exponents. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Rational Exponents--Example 4 | Math Example--Exponential Concepts--Rational Exponents--Example 4TopicExponents DescriptionThe image shows Example 4 of simplifying a rational exponent expression. The expression is 321/5. The solution involves converting the exponent to a fifth root. Solution: Convert the rational exponent to an nth root. 321/5 = 5√32 = 2. This example involves simplifying expressions with rational exponents, providing a concrete application of exponent properties and the concept of roots. The method breaks down complex expressions into simpler terms, illustrating step-by-step how to handle rational exponents. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Rational Exponents--Example 5 | Math Example--Exponential Concepts--Rational Exponents--Example 5TopicExponents |
Laws of Exponents |

|
Math Example--Exponential Concepts--Rational Exponents--Example 6 | Math Example--Exponential Concepts--Rational Exponents--Example 6TopicExponents |
Laws of Exponents |

|
Math Example--Exponential Concepts--Rational Exponents--Example 7 | Math Example--Exponential Concepts--Rational Exponents--Example 7TopicExponents |
Laws of Exponents |

|
Math Example--Exponential Concepts--Rational Exponents--Example 8 | Math Example--Exponential Concepts--Rational Exponents--Example 8TopicExponents |
Laws of Exponents |

|
Math Example--Exponential Concepts--Rational Exponents--Example 9 | Math Example--Exponential Concepts--Rational Exponents--Example 9TopicExponents DescriptionThe image shows Example 9 from a series of math problems on simplifying expressions with rational exponents. It includes a step-by-step solution for simplifying 272/3. The problem asks to simplify 272/3. The solution involves converting the fractional exponent to a root and exponent form: 272/3 = ∛272 = 32 = 9. This example involves simplifying expressions with rational exponents, providing a concrete application of exponent properties and the concept of roots. The method breaks down complex expressions into simpler terms, illustrating step-by-step how to handle rational exponents. |
Laws of Exponents |

|
Math Example--Exponential Concepts--Rational Exponents--Example 10 | Math Example--Exponential Concepts--Rational Exponents--Example 10TopicExponents DescriptionThe image shows Example 10 from a series of math problems on simplifying expressions with rational exponents. It includes a step-by-step solution for simplifying 813/4. The solution involves converting the fractional exponent to a root and exponent form: 813/4 = 4√813 = 33 = 27. This example involves simplifying expressions with rational exponents, providing a concrete application of exponent properties and the concept of roots. The method breaks down complex expressions into simpler terms, illustrating step-by-step how to handle rational exponents. |
Laws of Exponents |
|
Math Example: Fraction Operations--Multiplying Fractions with Whole Numbers--Example 1 | Multiplying Fractions with Whole Numbers--Example 1TopicFraction Operations |
Fractions and Mixed Numbers |
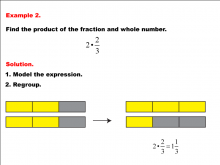
|
Math Example: Fraction Operations--Multiplying Fractions with Whole Numbers--Example 2 | Multiplying Fractions with Whole Numbers--Example 2TopicFraction Operations |
Fractions and Mixed Numbers |
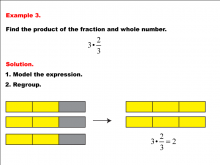
|
Math Example: Fraction Operations--Multiplying Fractions with Whole Numbers--Example 3 | Multiplying Fractions with Whole Numbers--Example 3TopicFraction Operations |
Fractions and Mixed Numbers |
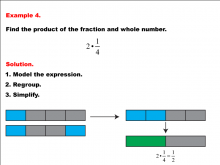
|
Math Example: Fraction Operations--Multiplying Fractions with Whole Numbers--Example 4 | Multiplying Fractions with Whole Numbers--Example 4TopicFraction Operations |
Fractions and Mixed Numbers |
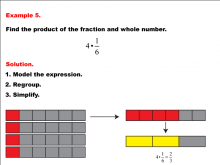
|
Math Example: Fraction Operations--Multiplying Fractions with Whole Numbers--Example 5 | Multiplying Fractions with Whole Numbers--Example 5TopicFraction Operations |
Fractions and Mixed Numbers |

|
Video Transcript: Rational Numbers: Adding Rational Numbers | Video Transcript: Rational Numbers: Adding Rational Numbers
What Are Rational Numbers?A Brief Review of Integers |
Rational Expressions |

|
Video Transcript: Rational Numbers: Comparing and Ordering Rational Numbers | Video Transcript: Rational Numbers: Comparing and Ordering Rational Numbers
This is the transcript that goes with the video segment entitled Video: Rational Numbers: Comparing and Ordering Rational Numbers. This is part of a collection of video transcripts for the video tutorial series on Rational Numbers. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Rational Expressions |

|
Video Transcript: Rational Numbers: Dividing Rational Numbers | Video Transcript: Rational Numbers: Dividing Rational Numbers
This is the transcript that goes with the video segment entitled Video: Rational Numbers: Dividing Rational Numbers. This is part of a collection of video transcripts for the video tutorial series on Rational Numbers. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Rational Expressions |

|
Video Transcript: Ratios: Equivalent Ratios | Video Transcript: Ratios: Equivalent Ratios
What Are Ratios?A ratio is the relationship between two or more quantities among a group of items. |
Ratios and Rates |

|
Video Transcript: Ratios: Fractional Ratios | Video Transcript: Ratios: Fractional Ratios
What Are Ratios?A ratio is the relationship between two or more quantities among a group of items. |
Ratios and Rates |

|
Video Transcript: Rational Numbers: Multiplying Rational Numbers | Video Transcript: Rational Numbers: Multiplying Rational Numbers
This is the transcript that goes with the video segment entitled Video: Rational Numbers: Multiplying Rational Numbers. This is part of a collection of video transcripts for the video tutorial series on Rational Numbers. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Rational Expressions |

|
Video Transcript: Rational Numbers: Numerical Expressions with Rational Numbers | Video Transcript: Rational Numbers: Numerical Expressions with Rational Numbers
This is the transcript that goes with the video segment entitled Video: Rational Numbers: Numerical Expressions with Rational Numbers. This is part of a collection of video transcripts for the video tutorial series on Rational Numbers. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Rational Expressions |

|
Video Transcript: Ratios: Numerical Models for Ratios | Video Transcript: Ratios: Numerical Models for Ratios
What Are Ratios?A ratio is the relationship between two or more quantities among a group of items. |
Ratios and Rates |

|
Video Transcript: Rational Numbers: Rational Numbers and Exponents | Video Transcript: Rational Numbers: Rational Numbers and Exponents
This is the transcript that goes with the video segment entitled Video Tutorial: Rational Numbers: Rational Numbers and Exponents. This is part of a collection of video transcripts for the video tutorial series on Rational Numbers. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Rational Expressions |

|
Video Transcript: Rational Numbers: Rational Numbers and Absolute Value | Video Transcript: Rational Numbers: Rational Numbers and Absolute Value
This is the transcript that goes with the video segment entitled Video Tutorial: Rational Numbers: Rational Numbers and Absolute Value. This is part of a collection of video transcripts for the video tutorial series on Rational Numbers. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Rational Expressions |

|
Video Transcript: Rational Numbers: Rational Numbers and Irrational Numbers | Video Transcript: Rational Numbers: Rational Numbers and Irrational Numbers
This is the transcript that goes with the video segment entitled Video Tutorial: Rational Numbers: Rational Numbers and Irrational Numbers. This is part of a collection of video transcripts for the video tutorial series on Rational Numbers. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Rational Expressions |

|
Video Transcript: Rational Numbers: Rational Numbers On a Number Line | Video Transcript: Rational Numbers: Rational Numbers On a Number Line
Distance Measures on a Number LineA Brief Review of Number Lines |
Rational Expressions |

|
Video Transcript: Rational Numbers: Rational Numbers On the Cartesian Coordinate System | Video Transcript: Rational Numbers: Rational Numbers On the Cartesian Coordinate System
This is the transcript that goes with the video segment entitled Video Tutorial: Rational Numbers: Rational Numbers On the Cartesian Coordinate System. This is part of a collection of video transcripts for the video tutorial series on Rational Numbers. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Rational Expressions |

|
Video Transcript: Ratios: Ratios and Fractions | Video Transcript: Ratios: Ratios and Fractions
What Are Ratios?A ratio is the relationship between two or more quantities among a group of items. |
Ratios and Rates |

|
Video Transcript: Ratios and Rates: Ratios as Decimals | Video Transcript: Ratios and Rates: Ratios as Decimals
This is the transcript that goes with the video segment entitled Video Tutorials: Ratios and Rates: Ratios and Decimals. This is part of a collection of video transcripts for the video tutorial series on Ratios. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. Video LibraryTo see the complete collection of videos in the Video Library, click on this link. |
Ratios and Rates |

|
Video Transcript: Ratios and Rates: Converting Measurement Units | Video Transcript: Ratios and Rates: Converting Measurement Units
This is the transcript that goes with the video segment entitled Video Tutorial: Ratios and Rates: Converting Measurement Units. This is part of a collection of video transcripts for the video tutorial series on Ratios. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Ratios and Rates |

|
Video Transcript: Ratios, Proportions, and Percents: Calculating Percents | Video Transcript: Ratios, Proportions, and Percents: Calculating Percents
This is the transcript that goes with the video segment entitled Video Tutorials: Ratios, Proportions, and Percents: Calculating Percents. This is part of a collection of video transcripts for the video tutorial series on Ratios. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Ratios and Rates |