
Illustrative Math Alignment: Grade 7 Unit 9
Putting it All Together
Lesson 5: How Crowded Is this Neighborhood?
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|
|
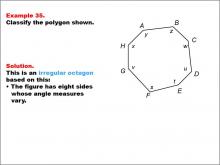
Math Example--Polygons--Polygon Classification: Example 35 | Math Example--Polygons--Polygon Classification: Example 35TopicPolygons DescriptionThis example presents an octagon with sides labeled as x, y, z, w, u, t, s, and v. The sides are of varying lengths and angles, indicating it is irregular. It illustrates how using different variables for both side lengths and angles can suggest irregularity in a polygon. Polygon classification is a crucial topic in geometry that helps students distinguish between regular and irregular shapes. This collection of examples provides a comprehensive look at various types of octagons, highlighting the importance of considering both side lengths and angle measures in determining regularity. |
Definition of a Polygon |
|
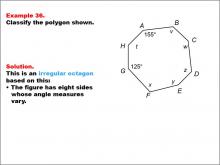
Math Example--Polygons--Polygon Classification: Example 36 | Math Example--Polygons--Polygon Classification: Example 36TopicPolygons DescriptionThis example features an octagon with angles labeled as 155° and 125°, and other angles labeled with variables t, v, w, x, y, and z. The angles vary, indicating it is irregular. It demonstrates how a combination of specific angle measures and variables can be used to show irregularity in polygons. Understanding polygon classification is essential in geometry as it helps students recognize and categorize shapes based on their properties. This collection of examples highlights the importance of angle measures in determining the regularity of octagons, showing that even partial information can be sufficient for classification. |
Definition of a Polygon |

|
Math Example--Polygons--Polygon Classification: Example 36 | Math Example--Polygons--Polygon Classification: Example 36TopicPolygons DescriptionThis example features an octagon with angles labeled as 155° and 125°, and other angles labeled with variables t, v, w, x, y, and z. The angles vary, indicating it is irregular. It demonstrates how a combination of specific angle measures and variables can be used to show irregularity in polygons. Understanding polygon classification is essential in geometry as it helps students recognize and categorize shapes based on their properties. This collection of examples highlights the importance of angle measures in determining the regularity of octagons, showing that even partial information can be sufficient for classification. |
Definition of a Polygon |
|
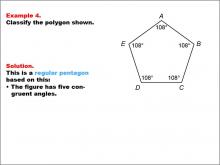
Math Example--Polygons--Polygon Classification: Example 4 | Math Example--Polygons--Polygon Classification: Example 4TopicPolygons DescriptionThis example showcases a pentagon with each angle labeled as 108 degrees, indicating congruent angles. It demonstrates that a regular pentagon can be identified not only by equal side lengths but also by equal angle measures. Understanding polygon classification is crucial in geometry as it helps students recognize and categorize shapes based on their properties. This collection of examples highlights different aspects of regular pentagons, emphasizing both side length and angle measure as defining characteristics. |
Definition of a Polygon |

|
Math Example--Polygons--Polygon Classification: Example 4 | Math Example--Polygons--Polygon Classification: Example 4TopicPolygons DescriptionThis example showcases a pentagon with each angle labeled as 108 degrees, indicating congruent angles. It demonstrates that a regular pentagon can be identified not only by equal side lengths but also by equal angle measures. Understanding polygon classification is crucial in geometry as it helps students recognize and categorize shapes based on their properties. This collection of examples highlights different aspects of regular pentagons, emphasizing both side length and angle measure as defining characteristics. |
Definition of a Polygon |
|
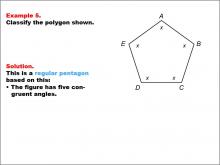
Math Example--Polygons--Polygon Classification: Example 5 | Math Example--Polygons--Polygon Classification: Example 5TopicPolygons DescriptionThis example presents a pentagon labeled with vertices A, B, C, D, and E, with each side marked with an "x" to indicate equal length. It reinforces the concept of a regular pentagon by visually representing the equality of all sides. Polygon classification is a fundamental topic in geometry that helps students understand the properties and relationships of different shapes. This collection of examples provides various representations of regular pentagons, emphasizing the importance of side length equality in classification. |
Definition of a Polygon |

|
Math Example--Polygons--Polygon Classification: Example 5 | Math Example--Polygons--Polygon Classification: Example 5TopicPolygons DescriptionThis example presents a pentagon labeled with vertices A, B, C, D, and E, with each side marked with an "x" to indicate equal length. It reinforces the concept of a regular pentagon by visually representing the equality of all sides. Polygon classification is a fundamental topic in geometry that helps students understand the properties and relationships of different shapes. This collection of examples provides various representations of regular pentagons, emphasizing the importance of side length equality in classification. |
Definition of a Polygon |

|
Math Example--Polygons--Polygon Classification: Example 6 | Math Example--Polygons--Polygon Classification: Example 6TopicPolygons DescriptionThis example features a pentagon labeled with vertices A, B, C, D, and E, where two angles are marked as 108 degrees, and the other angles are marked as "x". It demonstrates how to determine if a pentagon is regular by solving for the unknown angle measures. Understanding polygon classification is essential in geometry as it helps students analyze and categorize shapes based on their properties. This collection of examples highlights different aspects of regular pentagons, emphasizing both side length and angle measure as defining characteristics. |
Definition of a Polygon |

|
Math Example--Polygons--Polygon Classification: Example 6 | Math Example--Polygons--Polygon Classification: Example 6TopicPolygons DescriptionThis example features a pentagon labeled with vertices A, B, C, D, and E, where two angles are marked as 108 degrees, and the other angles are marked as "x". It demonstrates how to determine if a pentagon is regular by solving for the unknown angle measures. Understanding polygon classification is essential in geometry as it helps students analyze and categorize shapes based on their properties. This collection of examples highlights different aspects of regular pentagons, emphasizing both side length and angle measure as defining characteristics. |
Definition of a Polygon |
|
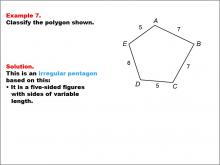
Math Example--Polygons--Polygon Classification: Example 7 | Math Example--Polygons--Polygon Classification: Example 7TopicPolygons DescriptionThis example showcases a pentagon labeled with vertices A, B, C, D, and E, where the sides have different lengths: 5, 7, 7, 5, and 6 units. It illustrates an irregular pentagon, demonstrating that not all five-sided figures are regular. Polygon classification is a crucial topic in geometry that helps students distinguish between regular and irregular shapes. This collection of examples provides a comprehensive look at various types of pentagons, highlighting the differences between regular and irregular polygons based on side lengths and angle measures. |
Definition of a Polygon |

|
Math Example--Polygons--Polygon Classification: Example 7 | Math Example--Polygons--Polygon Classification: Example 7TopicPolygons DescriptionThis example showcases a pentagon labeled with vertices A, B, C, D, and E, where the sides have different lengths: 5, 7, 7, 5, and 6 units. It illustrates an irregular pentagon, demonstrating that not all five-sided figures are regular. Polygon classification is a crucial topic in geometry that helps students distinguish between regular and irregular shapes. This collection of examples provides a comprehensive look at various types of pentagons, highlighting the differences between regular and irregular polygons based on side lengths and angle measures. |
Definition of a Polygon |
|
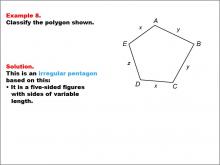
Math Example--Polygons--Polygon Classification: Example 8 | Math Example--Polygons--Polygon Classification: Example 8TopicPolygons DescriptionThis example presents a pentagon labeled with vertices A, B, C, D, and E, where the sides are marked with variables x, y, and z, indicating different lengths. It demonstrates another instance of an irregular pentagon, emphasizing that side length variation results in irregularity. Understanding polygon classification is crucial in geometry as it helps students recognize and categorize shapes based on their properties. This collection of examples highlights the differences between regular and irregular pentagons, focusing on side length as a key determining factor. |
Definition of a Polygon |

|
Math Example--Polygons--Polygon Classification: Example 8 | Math Example--Polygons--Polygon Classification: Example 8TopicPolygons DescriptionThis example presents a pentagon labeled with vertices A, B, C, D, and E, where the sides are marked with variables x, y, and z, indicating different lengths. It demonstrates another instance of an irregular pentagon, emphasizing that side length variation results in irregularity. Understanding polygon classification is crucial in geometry as it helps students recognize and categorize shapes based on their properties. This collection of examples highlights the differences between regular and irregular pentagons, focusing on side length as a key determining factor. |
Definition of a Polygon |
|
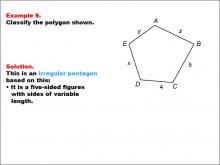
Math Example--Polygons--Polygon Classification: Example 9 | Math Example--Polygons--Polygon Classification: Example 9TopicPolygons DescriptionThis example features a pentagon labeled with vertices A, B, C, D, E, where the sides are labeled as y, z, 5, 4, and x. It illustrates another case of an irregular pentagon, demonstrating that the presence of different side lengths, including both numeric and variable representations, results in irregularity. Polygon classification is a fundamental concept in geometry that helps students analyze and categorize shapes based on their properties. This collection of examples provides a comprehensive look at various types of pentagons, emphasizing the importance of side length equality in determining regularity. |
Definition of a Polygon |

|
Math Example--Polygons--Polygon Classification: Example 9 | Math Example--Polygons--Polygon Classification: Example 9TopicPolygons DescriptionThis example features a pentagon labeled with vertices A, B, C, D, E, where the sides are labeled as y, z, 5, 4, and x. It illustrates another case of an irregular pentagon, demonstrating that the presence of different side lengths, including both numeric and variable representations, results in irregularity. Polygon classification is a fundamental concept in geometry that helps students analyze and categorize shapes based on their properties. This collection of examples provides a comprehensive look at various types of pentagons, emphasizing the importance of side length equality in determining regularity. |
Definition of a Polygon |
|
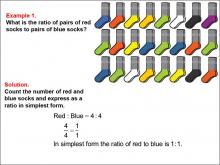
Math Example--Ratios and Rates--Example 1 | Math Example--Ratios and Rates--Example 1TopicRatios and Rates DescriptionThis math example focuses on understanding ratios using colored socks. The image displays a collection of red and blue socks, and students are asked to determine the ratio of red socks to blue socks. The solution demonstrates that there are 4 pairs of red socks and 4 pairs of blue socks, resulting in a ratio of 4 : 4, which simplifies to 1 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 1 | Math Example--Ratios and Rates--Example 1TopicRatios and Rates DescriptionThis math example focuses on understanding ratios using colored socks. The image displays a collection of red and blue socks, and students are asked to determine the ratio of red socks to blue socks. The solution demonstrates that there are 4 pairs of red socks and 4 pairs of blue socks, resulting in a ratio of 4 : 4, which simplifies to 1 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 1 | Math Example--Ratios and Rates--Example 1TopicRatios and Rates DescriptionThis math example focuses on understanding ratios using colored socks. The image displays a collection of red and blue socks, and students are asked to determine the ratio of red socks to blue socks. The solution demonstrates that there are 4 pairs of red socks and 4 pairs of blue socks, resulting in a ratio of 4 : 4, which simplifies to 1 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 1 | Math Example--Ratios and Rates--Example 1TopicRatios and Rates DescriptionThis math example focuses on understanding ratios using colored socks. The image displays a collection of red and blue socks, and students are asked to determine the ratio of red socks to blue socks. The solution demonstrates that there are 4 pairs of red socks and 4 pairs of blue socks, resulting in a ratio of 4 : 4, which simplifies to 1 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 1 | Math Example--Ratios and Rates--Example 1TopicRatios and Rates DescriptionThis math example focuses on understanding ratios using colored socks. The image displays a collection of red and blue socks, and students are asked to determine the ratio of red socks to blue socks. The solution demonstrates that there are 4 pairs of red socks and 4 pairs of blue socks, resulting in a ratio of 4 : 4, which simplifies to 1 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 1 | Math Example--Ratios and Rates--Example 1TopicRatios and Rates DescriptionThis math example focuses on understanding ratios using colored socks. The image displays a collection of red and blue socks, and students are asked to determine the ratio of red socks to blue socks. The solution demonstrates that there are 4 pairs of red socks and 4 pairs of blue socks, resulting in a ratio of 4 : 4, which simplifies to 1 : 1. |
Ratios and Rates |
|
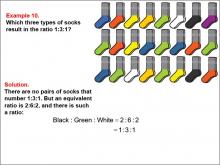
Math Example--Ratios and Rates--Example 10 | Math Example--Ratios and Rates--Example 10TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 3 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 10 | Math Example--Ratios and Rates--Example 10TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 3 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 10 | Math Example--Ratios and Rates--Example 10TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 3 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 10 | Math Example--Ratios and Rates--Example 10TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 3 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 10 | Math Example--Ratios and Rates--Example 10TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 3 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 10 | Math Example--Ratios and Rates--Example 10TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 3 : 1. |
Ratios and Rates |
|
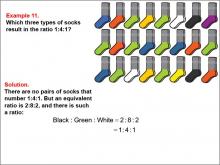
Math Example--Ratios and Rates--Example 11 | Math Example--Ratios and Rates--Example 11TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 4 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 11 | Math Example--Ratios and Rates--Example 11TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 4 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 11 | Math Example--Ratios and Rates--Example 11TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 4 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 11 | Math Example--Ratios and Rates--Example 11TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 4 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 11 | Math Example--Ratios and Rates--Example 11TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 4 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 11 | Math Example--Ratios and Rates--Example 11TopicRatios and Rates DescriptionThis example focuses on finding equivalent ratios using a collection of socks in various colors. The image shows socks arranged in rows, with black, green, white, and other colors present. Students are asked to find a ratio among three colors that is equivalent to 1 : 4 : 1. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 12 | Math Example--Ratios and Rates--Example 12TopicRatios and Rates DescriptionThis example explores the concept of similar triangles using ratios. The image shows a right triangle with sides labeled as 9, 12, and 15 units. Students are asked to determine if this triangle is similar to a standard 3-4-5 Pythagorean triplet triangle. Understanding similar triangles is an important application of ratios in geometry. This example demonstrates how ratios can be used to compare the sides of triangles and determine similarity. By scaling the sides of a known triangle (3-4-5) and comparing them to the given triangle, students can see how ratios maintain proportionality in similar shapes. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 12 | Math Example--Ratios and Rates--Example 12TopicRatios and Rates DescriptionThis example explores the concept of similar triangles using ratios. The image shows a right triangle with sides labeled as 9, 12, and 15 units. Students are asked to determine if this triangle is similar to a standard 3-4-5 Pythagorean triplet triangle. Understanding similar triangles is an important application of ratios in geometry. This example demonstrates how ratios can be used to compare the sides of triangles and determine similarity. By scaling the sides of a known triangle (3-4-5) and comparing them to the given triangle, students can see how ratios maintain proportionality in similar shapes. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 12 | Math Example--Ratios and Rates--Example 12TopicRatios and Rates DescriptionThis example explores the concept of similar triangles using ratios. The image shows a right triangle with sides labeled as 9, 12, and 15 units. Students are asked to determine if this triangle is similar to a standard 3-4-5 Pythagorean triplet triangle. Understanding similar triangles is an important application of ratios in geometry. This example demonstrates how ratios can be used to compare the sides of triangles and determine similarity. By scaling the sides of a known triangle (3-4-5) and comparing them to the given triangle, students can see how ratios maintain proportionality in similar shapes. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 12 | Math Example--Ratios and Rates--Example 12TopicRatios and Rates DescriptionThis example explores the concept of similar triangles using ratios. The image shows a right triangle with sides labeled as 9, 12, and 15 units. Students are asked to determine if this triangle is similar to a standard 3-4-5 Pythagorean triplet triangle. Understanding similar triangles is an important application of ratios in geometry. This example demonstrates how ratios can be used to compare the sides of triangles and determine similarity. By scaling the sides of a known triangle (3-4-5) and comparing them to the given triangle, students can see how ratios maintain proportionality in similar shapes. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 12 | Math Example--Ratios and Rates--Example 12TopicRatios and Rates DescriptionThis example explores the concept of similar triangles using ratios. The image shows a right triangle with sides labeled as 9, 12, and 15 units. Students are asked to determine if this triangle is similar to a standard 3-4-5 Pythagorean triplet triangle. Understanding similar triangles is an important application of ratios in geometry. This example demonstrates how ratios can be used to compare the sides of triangles and determine similarity. By scaling the sides of a known triangle (3-4-5) and comparing them to the given triangle, students can see how ratios maintain proportionality in similar shapes. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 12 | Math Example--Ratios and Rates--Example 12TopicRatios and Rates DescriptionThis example explores the concept of similar triangles using ratios. The image shows a right triangle with sides labeled as 9, 12, and 15 units. Students are asked to determine if this triangle is similar to a standard 3-4-5 Pythagorean triplet triangle. Understanding similar triangles is an important application of ratios in geometry. This example demonstrates how ratios can be used to compare the sides of triangles and determine similarity. By scaling the sides of a known triangle (3-4-5) and comparing them to the given triangle, students can see how ratios maintain proportionality in similar shapes. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 13 | Math Example--Ratios and Rates--Example 13TopicRatios and Rates DescriptionThis example focuses on verifying the similarity of triangles using ratios. The image shows a right triangle with sides labeled 25, 60, and 65 units. Students are guided through a step-by-step process to determine if this triangle is similar to a 5-12-13 right triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 13 | Math Example--Ratios and Rates--Example 13TopicRatios and Rates DescriptionThis example focuses on verifying the similarity of triangles using ratios. The image shows a right triangle with sides labeled 25, 60, and 65 units. Students are guided through a step-by-step process to determine if this triangle is similar to a 5-12-13 right triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 13 | Math Example--Ratios and Rates--Example 13TopicRatios and Rates DescriptionThis example focuses on verifying the similarity of triangles using ratios. The image shows a right triangle with sides labeled 25, 60, and 65 units. Students are guided through a step-by-step process to determine if this triangle is similar to a 5-12-13 right triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 13 | Math Example--Ratios and Rates--Example 13TopicRatios and Rates DescriptionThis example focuses on verifying the similarity of triangles using ratios. The image shows a right triangle with sides labeled 25, 60, and 65 units. Students are guided through a step-by-step process to determine if this triangle is similar to a 5-12-13 right triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 13 | Math Example--Ratios and Rates--Example 13TopicRatios and Rates DescriptionThis example focuses on verifying the similarity of triangles using ratios. The image shows a right triangle with sides labeled 25, 60, and 65 units. Students are guided through a step-by-step process to determine if this triangle is similar to a 5-12-13 right triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 13 | Math Example--Ratios and Rates--Example 13TopicRatios and Rates DescriptionThis example focuses on verifying the similarity of triangles using ratios. The image shows a right triangle with sides labeled 25, 60, and 65 units. Students are guided through a step-by-step process to determine if this triangle is similar to a 5-12-13 right triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 14 | Math Example--Ratios and Rates--Example 14TopicRatios and Rates DescriptionThis example explores the concept of special right triangles using ratios. The image shows a right triangle with sides labeled 3, 3 2 2 , and 6 units. Students are guided through a step-by-step process to verify if this triangle is similar to a 30°-60°-90° triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 14 | Math Example--Ratios and Rates--Example 14TopicRatios and Rates DescriptionThis example explores the concept of special right triangles using ratios. The image shows a right triangle with sides labeled 3, 3 2 2 , and 6 units. Students are guided through a step-by-step process to verify if this triangle is similar to a 30°-60°-90° triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 14 | Math Example--Ratios and Rates--Example 14TopicRatios and Rates DescriptionThis example explores the concept of special right triangles using ratios. The image shows a right triangle with sides labeled 3, 3 2 2 , and 6 units. Students are guided through a step-by-step process to verify if this triangle is similar to a 30°-60°-90° triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 14 | Math Example--Ratios and Rates--Example 14TopicRatios and Rates DescriptionThis example explores the concept of special right triangles using ratios. The image shows a right triangle with sides labeled 3, 3 2 2 , and 6 units. Students are guided through a step-by-step process to verify if this triangle is similar to a 30°-60°-90° triangle. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 14 | Math Example--Ratios and Rates--Example 14TopicRatios and Rates DescriptionThis example explores the concept of special right triangles using ratios. The image shows a right triangle with sides labeled 3, 3 2 2 , and 6 units. Students are guided through a step-by-step process to verify if this triangle is similar to a 30°-60°-90° triangle. |
Ratios and Rates |