
Illustrative Math Alignment: Grade 8 Unit 3
Linear Relationships
Lesson 11: Equations of All Kinds of Lines
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|

|
Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form | Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form
TopicLinear Functions DescriptionThe term is "Converting from Standard Form to Slope-Intercept Form," defined as rewriting a linear equation in the form y = mx + b using the constants from the standard form Ax + By = C. The conversion formula is y = -A/B * x + C/B. This term is crucial for transitioning between different representations of linear equations, enabling easier analysis and graphing. |
Slope-Intercept Form |

|
Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form | Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form
TopicLinear Functions DescriptionThe term is "Converting from Standard Form to Slope-Intercept Form," defined as rewriting a linear equation in the form y = mx + b using the constants from the standard form Ax + By = C. The conversion formula is y = -A/B * x + C/B. This term is crucial for transitioning between different representations of linear equations, enabling easier analysis and graphing. |
Slope-Intercept Form |

|
Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form | Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form
TopicLinear Functions DescriptionThe term is "Converting from Standard Form to Slope-Intercept Form," defined as rewriting a linear equation in the form y = mx + b using the constants from the standard form Ax + By = C. The conversion formula is y = -A/B * x + C/B. This term is crucial for transitioning between different representations of linear equations, enabling easier analysis and graphing. |
Slope-Intercept Form |

|
Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form | Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form
TopicLinear Functions DescriptionThe term is "Converting from Standard Form to Slope-Intercept Form," defined as rewriting a linear equation in the form y = mx + b using the constants from the standard form Ax + By = C. The conversion formula is y = -A/B * x + C/B. This term is crucial for transitioning between different representations of linear equations, enabling easier analysis and graphing. |
Slope-Intercept Form |

|
Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form | Video Definition 16--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form
TopicLinear Functions DescriptionThe term is "Converting from Standard Form to Slope-Intercept Form," defined as rewriting a linear equation in the form y = mx + b using the constants from the standard form Ax + By = C. The conversion formula is y = -A/B * x + C/B. This term is crucial for transitioning between different representations of linear equations, enabling easier analysis and graphing. |
Slope-Intercept Form |
|
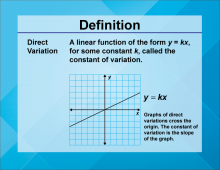
Video Definition 17--Linear Function Concepts--Direct Variation | Video Definition 17--Linear Function Concepts--Direct Variation
TopicLinear Functions DescriptionThe term is "Direct Variation," defined as a linear function of the form y = kx, where k is the constant of variation. Graphs of direct variations cross the origin, and the constant of variation is the slope of the graph. This term emphasizes proportional relationships in linear functions, highlighting their simplicity and graphical characteristics. |
Slope-Intercept Form |

|
Video Definition 17--Linear Function Concepts--Direct Variation | Video Definition 17--Linear Function Concepts--Direct Variation
TopicLinear Functions DescriptionThe term is "Direct Variation," defined as a linear function of the form y = kx, where k is the constant of variation. Graphs of direct variations cross the origin, and the constant of variation is the slope of the graph. This term emphasizes proportional relationships in linear functions, highlighting their simplicity and graphical characteristics. |
Slope-Intercept Form |

|
Video Definition 17--Linear Function Concepts--Direct Variation | Video Definition 17--Linear Function Concepts--Direct Variation
TopicLinear Functions DescriptionThe term is "Direct Variation," defined as a linear function of the form y = kx, where k is the constant of variation. Graphs of direct variations cross the origin, and the constant of variation is the slope of the graph. This term emphasizes proportional relationships in linear functions, highlighting their simplicity and graphical characteristics. |
Slope-Intercept Form |

|
Video Definition 17--Linear Function Concepts--Direct Variation | Video Definition 17--Linear Function Concepts--Direct Variation
TopicLinear Functions DescriptionThe term is "Direct Variation," defined as a linear function of the form y = kx, where k is the constant of variation. Graphs of direct variations cross the origin, and the constant of variation is the slope of the graph. This term emphasizes proportional relationships in linear functions, highlighting their simplicity and graphical characteristics. |
Slope-Intercept Form |

|
Video Definition 17--Linear Function Concepts--Direct Variation | Video Definition 17--Linear Function Concepts--Direct Variation
TopicLinear Functions DescriptionThe term is "Direct Variation," defined as a linear function of the form y = kx, where k is the constant of variation. Graphs of direct variations cross the origin, and the constant of variation is the slope of the graph. This term emphasizes proportional relationships in linear functions, highlighting their simplicity and graphical characteristics. |
Slope-Intercept Form |

|
Video Definition 17--Linear Function Concepts--Direct Variation | Video Definition 17--Linear Function Concepts--Direct Variation
TopicLinear Functions DescriptionThe term is "Direct Variation," defined as a linear function of the form y = kx, where k is the constant of variation. Graphs of direct variations cross the origin, and the constant of variation is the slope of the graph. This term emphasizes proportional relationships in linear functions, highlighting their simplicity and graphical characteristics. |
Slope-Intercept Form |

|
Video Definition 18--Linear Function Concepts--Line of Best Fit | Video Definition 18--Linear Function Concepts--Line of Best Fit
TopicLinear Functions DescriptionThe term is "Line of Best Fit," defined as a linear function that serves as a model for a set of scatterplot data. It helps represent the central trend of data points. This term enhances understanding of linear functions in the context of data analysis and graphical trends. |
Graphs of Linear Functions |

|
Video Definition 18--Linear Function Concepts--Line of Best Fit | Video Definition 18--Linear Function Concepts--Line of Best Fit
TopicLinear Functions DescriptionThe term is "Line of Best Fit," defined as a linear function that serves as a model for a set of scatterplot data. It helps represent the central trend of data points. This term enhances understanding of linear functions in the context of data analysis and graphical trends. |
Graphs of Linear Functions |

|
Video Definition 18--Linear Function Concepts--Line of Best Fit | Video Definition 18--Linear Function Concepts--Line of Best Fit
TopicLinear Functions DescriptionThe term is "Line of Best Fit," defined as a linear function that serves as a model for a set of scatterplot data. It helps represent the central trend of data points. This term enhances understanding of linear functions in the context of data analysis and graphical trends. |
Graphs of Linear Functions |

|
Video Definition 18--Linear Function Concepts--Line of Best Fit | Video Definition 18--Linear Function Concepts--Line of Best Fit
TopicLinear Functions DescriptionThe term is "Line of Best Fit," defined as a linear function that serves as a model for a set of scatterplot data. It helps represent the central trend of data points. This term enhances understanding of linear functions in the context of data analysis and graphical trends. |
Graphs of Linear Functions |

|
Video Definition 18--Linear Function Concepts--Line of Best Fit | Video Definition 18--Linear Function Concepts--Line of Best Fit
TopicLinear Functions DescriptionThe term is "Line of Best Fit," defined as a linear function that serves as a model for a set of scatterplot data. It helps represent the central trend of data points. This term enhances understanding of linear functions in the context of data analysis and graphical trends. |
Graphs of Linear Functions |

|
Video Definition 18--Linear Function Concepts--Line of Best Fit | Video Definition 18--Linear Function Concepts--Line of Best Fit
TopicLinear Functions DescriptionThe term is "Line of Best Fit," defined as a linear function that serves as a model for a set of scatterplot data. It helps represent the central trend of data points. This term enhances understanding of linear functions in the context of data analysis and graphical trends. |
Graphs of Linear Functions |
|
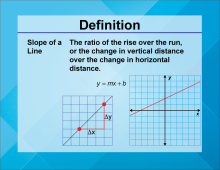
Video Definition 19--Linear Function Concepts--Slope | Video Definition 19--Linear Function Concepts--Slope
TopicLinear Functions DescriptionThe term is "Slope of a Line," defined as the ratio of the rise over the run, or the change in vertical distance over the change in horizontal distance. Represented as m in the equation y = mx + b. This term is a fundamental component of linear equations, providing a measure of steepness and direction. |
Slope |

|
Video Definition 19--Linear Function Concepts--Slope | Video Definition 19--Linear Function Concepts--Slope
TopicLinear Functions DescriptionThe term is "Slope of a Line," defined as the ratio of the rise over the run, or the change in vertical distance over the change in horizontal distance. Represented as m in the equation y = mx + b. This term is a fundamental component of linear equations, providing a measure of steepness and direction. |
Slope |

|
Video Definition 19--Linear Function Concepts--Slope | Video Definition 19--Linear Function Concepts--Slope
TopicLinear Functions DescriptionThe term is "Slope of a Line," defined as the ratio of the rise over the run, or the change in vertical distance over the change in horizontal distance. Represented as m in the equation y = mx + b. This term is a fundamental component of linear equations, providing a measure of steepness and direction. |
Slope |

|
Video Definition 19--Linear Function Concepts--Slope | Video Definition 19--Linear Function Concepts--Slope
TopicLinear Functions DescriptionThe term is "Slope of a Line," defined as the ratio of the rise over the run, or the change in vertical distance over the change in horizontal distance. Represented as m in the equation y = mx + b. This term is a fundamental component of linear equations, providing a measure of steepness and direction. |
Slope |

|
Video Definition 19--Linear Function Concepts--Slope | Video Definition 19--Linear Function Concepts--Slope
TopicLinear Functions DescriptionThe term is "Slope of a Line," defined as the ratio of the rise over the run, or the change in vertical distance over the change in horizontal distance. Represented as m in the equation y = mx + b. This term is a fundamental component of linear equations, providing a measure of steepness and direction. |
Slope |

|
Video Definition 19--Linear Function Concepts--Slope | Video Definition 19--Linear Function Concepts--Slope
TopicLinear Functions DescriptionThe term is "Slope of a Line," defined as the ratio of the rise over the run, or the change in vertical distance over the change in horizontal distance. Represented as m in the equation y = mx + b. This term is a fundamental component of linear equations, providing a measure of steepness and direction. |
Slope |
|
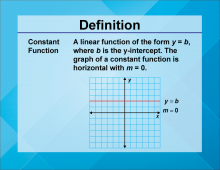
Video Definition 2--Linear Function Concepts--Constant Function | Video Definition 2--Linear Function Concepts--Constant Function
TopicLinear Functions DescriptionThe term is "Constant Function," defined as a linear function of the form y = b, where b is the y-intercept. The graph of a constant function is horizontal with a slope of m = 0. An example graph is shown with a horizontal line at y = b. This term demonstrates a special case of linear functions, emphasizing the role of constant values and their graphical representation as horizontal lines. |
Slope-Intercept Form |

|
Video Definition 2--Linear Function Concepts--Constant Function | Video Definition 2--Linear Function Concepts--Constant Function
TopicLinear Functions DescriptionThe term is "Constant Function," defined as a linear function of the form y = b, where b is the y-intercept. The graph of a constant function is horizontal with a slope of m = 0. An example graph is shown with a horizontal line at y = b. This term demonstrates a special case of linear functions, emphasizing the role of constant values and their graphical representation as horizontal lines. |
Slope-Intercept Form |

|
Video Definition 2--Linear Function Concepts--Constant Function | Video Definition 2--Linear Function Concepts--Constant Function
TopicLinear Functions DescriptionThe term is "Constant Function," defined as a linear function of the form y = b, where b is the y-intercept. The graph of a constant function is horizontal with a slope of m = 0. An example graph is shown with a horizontal line at y = b. This term demonstrates a special case of linear functions, emphasizing the role of constant values and their graphical representation as horizontal lines. |
Slope-Intercept Form |

|
Video Definition 2--Linear Function Concepts--Constant Function | Video Definition 2--Linear Function Concepts--Constant Function
TopicLinear Functions DescriptionThe term is "Constant Function," defined as a linear function of the form y = b, where b is the y-intercept. The graph of a constant function is horizontal with a slope of m = 0. An example graph is shown with a horizontal line at y = b. This term demonstrates a special case of linear functions, emphasizing the role of constant values and their graphical representation as horizontal lines. |
Slope-Intercept Form |

|
Video Definition 2--Linear Function Concepts--Constant Function | Video Definition 2--Linear Function Concepts--Constant Function
TopicLinear Functions DescriptionThe term is "Constant Function," defined as a linear function of the form y = b, where b is the y-intercept. The graph of a constant function is horizontal with a slope of m = 0. An example graph is shown with a horizontal line at y = b. This term demonstrates a special case of linear functions, emphasizing the role of constant values and their graphical representation as horizontal lines. |
Slope-Intercept Form |

|
Video Definition 2--Linear Function Concepts--Constant Function | Video Definition 2--Linear Function Concepts--Constant Function
TopicLinear Functions DescriptionThe term is "Constant Function," defined as a linear function of the form y = b, where b is the y-intercept. The graph of a constant function is horizontal with a slope of m = 0. An example graph is shown with a horizontal line at y = b. This term demonstrates a special case of linear functions, emphasizing the role of constant values and their graphical representation as horizontal lines. |
Slope-Intercept Form |

|
Video Definition 20--Linear Function Concepts--System of Linear Equations | Video Definition 20--Linear Function Concepts--System of Linear Equations
TopicLinear Functions DescriptionThe term is "System of Linear Equations," defined as a collection of linear equations where the number of equations matches the number of variables, allowing for an algebraic solution. Examples include 2-variable and 3-variable systems. This term introduces the concept of interacting linear equations, paving the way for multi-variable problem-solving. |
Applications of Linear Functions and Graphs of Linear Functions |

|
Video Definition 20--Linear Function Concepts--System of Linear Equations | Video Definition 20--Linear Function Concepts--System of Linear Equations
TopicLinear Functions DescriptionThe term is "System of Linear Equations," defined as a collection of linear equations where the number of equations matches the number of variables, allowing for an algebraic solution. Examples include 2-variable and 3-variable systems. This term introduces the concept of interacting linear equations, paving the way for multi-variable problem-solving. |
Applications of Linear Functions and Graphs of Linear Functions |

|
Video Definition 20--Linear Function Concepts--System of Linear Equations | Video Definition 20--Linear Function Concepts--System of Linear Equations
TopicLinear Functions DescriptionThe term is "System of Linear Equations," defined as a collection of linear equations where the number of equations matches the number of variables, allowing for an algebraic solution. Examples include 2-variable and 3-variable systems. This term introduces the concept of interacting linear equations, paving the way for multi-variable problem-solving. |
Applications of Linear Functions and Graphs of Linear Functions |

|
Video Definition 20--Linear Function Concepts--System of Linear Equations | Video Definition 20--Linear Function Concepts--System of Linear Equations
TopicLinear Functions DescriptionThe term is "System of Linear Equations," defined as a collection of linear equations where the number of equations matches the number of variables, allowing for an algebraic solution. Examples include 2-variable and 3-variable systems. This term introduces the concept of interacting linear equations, paving the way for multi-variable problem-solving. |
Applications of Linear Functions and Graphs of Linear Functions |

|
Video Definition 20--Linear Function Concepts--System of Linear Equations | Video Definition 20--Linear Function Concepts--System of Linear Equations
TopicLinear Functions DescriptionThe term is "System of Linear Equations," defined as a collection of linear equations where the number of equations matches the number of variables, allowing for an algebraic solution. Examples include 2-variable and 3-variable systems. This term introduces the concept of interacting linear equations, paving the way for multi-variable problem-solving. |
Applications of Linear Functions and Graphs of Linear Functions |

|
Video Definition 20--Linear Function Concepts--System of Linear Equations | Video Definition 20--Linear Function Concepts--System of Linear Equations
TopicLinear Functions DescriptionThe term is "System of Linear Equations," defined as a collection of linear equations where the number of equations matches the number of variables, allowing for an algebraic solution. Examples include 2-variable and 3-variable systems. This term introduces the concept of interacting linear equations, paving the way for multi-variable problem-solving. |
Applications of Linear Functions and Graphs of Linear Functions |

|
Video Definition 21--Linear Function Concepts--Solution to a Linear Equation | Video Definition 21--Linear Function Concepts--Solution to a Linear Equation
TopicLinear Functions DescriptionThe term is "Solution to a Linear Equation," defined as finding the value(s) of a variable that make the equation true. An example includes solving 2x + 5 = 15 step-by-step to find x = 5. This term explains how linear equations are solved, demonstrating the logical process of isolating variables. |
Solving Systems of Equations |

|
Video Definition 21--Linear Function Concepts--Solution to a Linear Equation | Video Definition 21--Linear Function Concepts--Solution to a Linear Equation
TopicLinear Functions DescriptionThe term is "Solution to a Linear Equation," defined as finding the value(s) of a variable that make the equation true. An example includes solving 2x + 5 = 15 step-by-step to find x = 5. This term explains how linear equations are solved, demonstrating the logical process of isolating variables. |
Solving Systems of Equations |

|
Video Definition 21--Linear Function Concepts--Solution to a Linear Equation | Video Definition 21--Linear Function Concepts--Solution to a Linear Equation
TopicLinear Functions DescriptionThe term is "Solution to a Linear Equation," defined as finding the value(s) of a variable that make the equation true. An example includes solving 2x + 5 = 15 step-by-step to find x = 5. This term explains how linear equations are solved, demonstrating the logical process of isolating variables. |
Solving Systems of Equations |

|
Video Definition 21--Linear Function Concepts--Solution to a Linear Equation | Video Definition 21--Linear Function Concepts--Solution to a Linear Equation
TopicLinear Functions DescriptionThe term is "Solution to a Linear Equation," defined as finding the value(s) of a variable that make the equation true. An example includes solving 2x + 5 = 15 step-by-step to find x = 5. This term explains how linear equations are solved, demonstrating the logical process of isolating variables. |
Solving Systems of Equations |

|
Video Definition 21--Linear Function Concepts--Solution to a Linear Equation | Video Definition 21--Linear Function Concepts--Solution to a Linear Equation
TopicLinear Functions DescriptionThe term is "Solution to a Linear Equation," defined as finding the value(s) of a variable that make the equation true. An example includes solving 2x + 5 = 15 step-by-step to find x = 5. This term explains how linear equations are solved, demonstrating the logical process of isolating variables. |
Solving Systems of Equations |

|
Video Definition 21--Linear Function Concepts--Solution to a Linear Equation | Video Definition 21--Linear Function Concepts--Solution to a Linear Equation
TopicLinear Functions DescriptionThe term is "Solution to a Linear Equation," defined as finding the value(s) of a variable that make the equation true. An example includes solving 2x + 5 = 15 step-by-step to find x = 5. This term explains how linear equations are solved, demonstrating the logical process of isolating variables. |
Solving Systems of Equations |

|
Video Definition 22--Linear Function Concepts--Proportional Relationship | Video Definition 22--Linear Function Concepts--Proportional Relationship
TopicLinear Functions DescriptionThe term is "Proportional Relationship," defined as a type of linear relationship where two variables maintain a constant ratio. Represented as y = kx, where k is the constant ratio. This term establishes the foundation for understanding direct variation and proportionality in linear relationships. |
Proportions |

|
Video Definition 22--Linear Function Concepts--Proportional Relationship | Video Definition 22--Linear Function Concepts--Proportional Relationship
TopicLinear Functions DescriptionThe term is "Proportional Relationship," defined as a type of linear relationship where two variables maintain a constant ratio. Represented as y = kx, where k is the constant ratio. This term establishes the foundation for understanding direct variation and proportionality in linear relationships. |
Proportions |

|
Video Definition 22--Linear Function Concepts--Proportional Relationship | Video Definition 22--Linear Function Concepts--Proportional Relationship
TopicLinear Functions DescriptionThe term is "Proportional Relationship," defined as a type of linear relationship where two variables maintain a constant ratio. Represented as y = kx, where k is the constant ratio. This term establishes the foundation for understanding direct variation and proportionality in linear relationships. |
Proportions |

|
Video Definition 22--Linear Function Concepts--Proportional Relationship | Video Definition 22--Linear Function Concepts--Proportional Relationship
TopicLinear Functions DescriptionThe term is "Proportional Relationship," defined as a type of linear relationship where two variables maintain a constant ratio. Represented as y = kx, where k is the constant ratio. This term establishes the foundation for understanding direct variation and proportionality in linear relationships. |
Proportions |

|
Video Definition 22--Linear Function Concepts--Proportional Relationship | Video Definition 22--Linear Function Concepts--Proportional Relationship
TopicLinear Functions DescriptionThe term is "Proportional Relationship," defined as a type of linear relationship where two variables maintain a constant ratio. Represented as y = kx, where k is the constant ratio. This term establishes the foundation for understanding direct variation and proportionality in linear relationships. |
Proportions |

|
Video Definition 22--Linear Function Concepts--Proportional Relationship | Video Definition 22--Linear Function Concepts--Proportional Relationship
TopicLinear Functions DescriptionThe term is "Proportional Relationship," defined as a type of linear relationship where two variables maintain a constant ratio. Represented as y = kx, where k is the constant ratio. This term establishes the foundation for understanding direct variation and proportionality in linear relationships. |
Proportions |
|
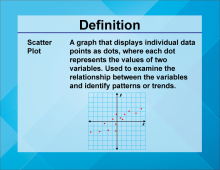
Video Definition 23--Linear Function Concepts--Scatter Plot | Video Definition 23--Linear Function Concepts--Scatter Plot
TopicLinear Functions DescriptionThe term is "Scatter Plot," defined as a graph displaying individual data points as dots, where each dot represents the values of two variables. Used to examine relationships and identify patterns or trends. This term provides a graphical tool to visualize and analyze linear relationships in data. |
Data Analysis |

|
Video Definition 23--Linear Function Concepts--Scatter Plot | Video Definition 23--Linear Function Concepts--Scatter Plot
TopicLinear Functions DescriptionThe term is "Scatter Plot," defined as a graph displaying individual data points as dots, where each dot represents the values of two variables. Used to examine relationships and identify patterns or trends. This term provides a graphical tool to visualize and analyze linear relationships in data. |
Data Analysis |

|
Video Definition 23--Linear Function Concepts--Scatter Plot | Video Definition 23--Linear Function Concepts--Scatter Plot
TopicLinear Functions DescriptionThe term is "Scatter Plot," defined as a graph displaying individual data points as dots, where each dot represents the values of two variables. Used to examine relationships and identify patterns or trends. This term provides a graphical tool to visualize and analyze linear relationships in data. |
Data Analysis |