
Illustrative Math Alignment: Grade 8 Unit 3
Functions and Volume
Lesson 13: The Volume of a Cylinder
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|

|
Holiday Themed Resource Collection: Math in the News |
This is a collection of Holiday-Themed issues of Math in the News.
|
Data Analysis, Applications of Exponential and Logarithmic Functions, Surface Area and Volume |
|
Math Examples Collection: Circular Area and Circumference | OverviewThis collection aggregates all the math examples around the topic of Circular Area and Circumference. There are a total of 23 Math Examples. This collection of resources is made up of downloadable PNG images that you can easily incorporate into your lesson plans. |
Area and Circumference |

|
Math Examples Collection: Modeling Volume | OverviewThis collection of math examples on the topic of Volume provides a comprehensive approach to understanding and mastering volume calculations. These examples increase in complexity and cover a range of essential skills, from basic volume calculations of simple shapes to more advanced composite figures. Using visual models, these examples simplify complex concepts, helping students grasp the intricacies of volume calculation through clear, engaging representations. |
Volume |

|
Math Examples Collection: Volume | OverviewThis collection of math examples on the topic of Volume provides students with an in-depth exploration of volume concepts through a variety of visual models. Covering a range of skills and increasing in complexity, this collection helps students master foundational and advanced topics in volume. From calculating the volume of basic geometric shapes to applying volume formulas to complex objects, these examples build confidence and enhance comprehension by breaking down challenging concepts visually. |
Volume |

|
Math Video Collection: Geometry Applications Video Series: 3D Geometry | OverviewThis collection aggregates all the math videos and resources in this series: Geometry Applications Video Series: 3D Geometry. There are a total of 18 resources. This collection of resources is made up of downloadable MP4, transcripts, and other resources files that you can easily incorporate into a presentation.
|
3-Dimensional Figures, Applications of 3D Geometry, Pyramids, Cylinders, Rectangular Prisms and Triangular Prisms |

|
Math Video Collection: Geometry Applications Video Series: Circles |
OverviewThis collection aggregates all the math videos and resources in this series: Geometry Applications Video Series: Circles. There are a total of 13 resources. This collection of resources is made up of downloadable MP4, transcripts, and other resources files that you can easily incorporate into a presentation.
|
Applications of Circles and Definition of a Circle |

|
Math Video Collection: 3D Geometry Animations |
OverviewThis collection aggregates all the math videos and resources in this series: 3D Geometry Animations. There are a total of 10 resources. This collection of resources is made up of downloadable MP4, transcripts, and other resources files that you can easily incorporate into a presentation.
|
3-Dimensional Figures, Cubes, Cones, Triangular Prisms, Pyramids, Cylinders and Rectangular Prisms |
|
Math Definitions Collection: Circles | OverviewThe collection of definitions on the topic of circles provided by Media4Math is an invaluable resource for students and educators alike. This comprehensive set includes essential terms such as radius, diameter, circumference, chord, and tangent, each explained with clarity and precision. Understanding these fundamental concepts is crucial for students as they form the building blocks for more advanced studies in geometry and algebra. |
Definition of a Circle |

|
Math in the News Collection: Applications of Data Analysis |
OverviewThis is a collection of Math in the News stories that focus on the topic of Data Analysis.
|
Data Analysis, Data Gathering, Probability, Percents and Ratios and Rates |

|
Math in the News Collection: Business Math |
OverviewThis is a collection of issues of Math in the News that deal with business applications.
|
Applications of Exponential and Logarithmic Functions, Data Analysis and Volume |

|
Math in the News Collection: Applications of 3D Geometry |
OverviewThis is a collection of issues of Math in the News that deal with applications of 3D geometry.
|
3-Dimensional Figures and Applications of 3D Geometry |

|
Math in the News Collection: Applications of Surface Area and Volume |
OverviewThis is a collection of issues of Math in the News that deal with applications of Surface Area and Volume.
|
Surface Area and Volume |

|
Lesson Plan Collection: Cross-Sections of 3D Figures | Cross-Sections of 3D Figures | 3-Lesson Unit This collection of geometry lesson plans explores how slicing three-dimensional figures produces different cross-sections. |
3-Dimensional Figures and Applications of 3D Geometry |

|
VIDEO: 3D Geometry Animation: Antiprism | VIDEO: 3D Geometry Animation: Antiprism
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures and Triangular Prisms |

|
VIDEO: 3D Geometry Animation: Cone | VIDEO: 3D Geometry Animation: Cone
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures and Cones |

|
VIDEO: 3D Geometry Animation: Cube | VIDEO: 3D Geometry Animation: Cube
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures and Cubes |

|
VIDEO: 3D Geometry Animation: Cylinder | 3D Geometry Animation: Cylinder
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures and Cylinders |

|
VIDEO: 3D Geometry Animation: Octahedron | VIDEO: 3D Geometry Animation: Octahedron
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures |

|
VIDEO: 3D Geometry Animation: Pyramid | VIDEO: 3D Geometry Animation: Pyramid
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures and Pyramids |

|
VIDEO: 3D Geometry Animation: Rectangular Prism | VIDEO: 3D Geometry Animation: Rectangular Prism
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures and Rectangular Prisms |

|
VIDEO: 3D Geometry Animation: Tetrahedron | VIDEO: 3D Geometry Animation: Tetrahedron
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures |

|
VIDEO: 3D Geometry Animation: Triangular Prism | VIDEO: 3D Geometry Animation: Triangular Prism
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Cube with Horizontal Cross-Section | Cube with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows a cube with a horizontal cross-section, illustrating how slicing the cube parallel to its base results in a square cross-section. This is useful for visualizing and understanding the internal structure and symmetry of cubes. Using animated math clip art like this helps students visualize the concept of cross-sections and their applications in geometry. Teachers can use this image to explain how horizontal cross-sections reveal the internal structure of cubes. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Dodecahedron | DodecahedronTopic3D Geometry DescriptionA dodecahedron is a three-dimensional shape with twelve flat faces, each a regular pentagon. This animated clip art shows the rotation of a dodecahedron, highlighting its symmetry and geometric properties. Animated math clip art like this is crucial for teaching as it allows students to visualize complex polyhedra, enhancing their understanding of geometric concepts. Teachers can use this image to explain the structure and characteristics of dodecahedrons. Here is a potential script for teachers: "Today, we are going to explore the geometry of a dodecahedron. Notice how the twelve pentagonal faces form a symmetrical shape. This helps us understand the properties of polyhedra." |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Horizontal Cross-Section of a Rectangular Prism | Animated Math Clip Art: Horizontal Cross-Section of a Rectangular Prism This animated math clip art illustrates how a horizontal plane intersects a rectangular prism, forming a two-dimensional cross-section. As the plane moves through the prism, students can observe that the resulting cross-section is always a rectangle, reinforcing key geometric concepts related to slicing three-dimensional shapes. Ideal for classroom instruction, this GIF animation helps students develop spatial reasoning by demonstrating how horizontal slices interact with the structure of a rectangular prism. This visual aid is particularly useful for teaching cross-sections in geometry, emphasizing how different slicing directions produce distinct 2D shapes. |
Rectangular Prisms |

|
Animated Math Clip Art--3D Geometry--Rotating a Rectangle to Form a Cylinder | Rotating a Rectangle to Form a CylinderTopic3D Geometry DescriptionThis animation demonstrates how rotating a rectangle around one of its sides creates a cylinder. It's a powerful visualization of how 2D shapes can generate 3D objects through rotation. Using animated math clip art like this helps students understand the concept of solids of revolution. Teachers can use this to introduce topics in calculus, such as finding volumes using the disk or washer methods. |
Cylinders |
|
Animated Math Clip Art--3D Geometry--Rotating a Rectangle to Form an Open Cylinder | Rotating a Rectangle to Form an Open CylinderTopic3D Geometry DescriptionThis animation shows how rotating a rectangular strip around an axis parallel to one of its sides creates an open cylinder. It illustrates the concept of surface area of revolution. Animated math clip art like this is valuable for teaching as it helps students visualize how 2D shapes can generate 3D surfaces. Teachers can use this to introduce topics in calculus, such as finding surface areas of revolution. |
Cylinders |

|
Animated Math Clip Art--3D Geometry--Rotating a Square to Form a Cylinder | Rotating a Square to Form a CylinderTopic3D Geometry DescriptionThis animation demonstrates how rotating a square around one of its sides creates a cylinder. It's a special case of rotating a rectangle, where the height and diameter of the resulting cylinder are equal. Using animated math clip art like this helps students understand the relationship between 2D and 3D shapes. Teachers can use this to discuss how the dimensions of the original shape relate to the dimensions of the resulting solid. |
Cylinders |

|
Animated Math Clip Art--3D Geometry--Vertical Cross-Section of a Rectangular Prism | Animated Math Clip Art: Vertical Cross-Section of a Rectangular Prism This animated math clip art visually demonstrates how a vertical plane intersects a rectangular prism, producing a two-dimensional cross-section. As the plane moves through the 3D shape, students can observe how the resulting cross-section remains a rectangle, reinforcing key geometric concepts related to slicing three-dimensional figures. Perfect for classroom instruction, this GIF animation helps students develop spatial reasoning by illustrating how a vertical slice interacts with the faces of a rectangular prism. This resource is particularly useful for teaching cross-sections in geometry and preparing students to understand more complex 3D slicing concepts. |
Rectangular Prisms |

|
Closed Captioned Video: Geometry Applications: 3D Geometry | Closed Captioned Video: Geometry Applications: 3D GeometryTopic3D Geometry |
3-Dimensional Figures and Applications of 3D Geometry |

|
Closed Captioned Video: Geometry Applications: 3D Geometry, 1 | Closed Captioned Video: Geometry Applications: 3D Geometry, 1Topic3D Geometry DescriptionDescription: This segment introduces Platonic solids, including tetrahedron, cube, and dodecahedron, emphasizing their congruent edges, vertices, and angles. It connects these geometric figures to both natural and man-made structures, setting the stage for exploring 3D geometry applications. |
3-Dimensional Figures and Applications of 3D Geometry |

|
Closed Captioned Video: Geometry Applications: 3D Geometry, 2 | Closed Captioned Video: Geometry Applications: 3D Geometry, 2Topic3D Geometry DescriptionDescription: This segment explores pyramids, particularly Mayan and Egyptian structures, to distinguish rectangular and square bases. It discusses the volume of truncated pyramids using proportional relationships and introduces the concept of tiered volume reduction through geometric sequences. |
3-Dimensional Figures, Pyramids and Applications of 3D Geometry |

|
Closed Captioned Video: Geometry Applications: 3D Geometry, 3 | Closed Captioned Video: Geometry Applications: 3D Geometry, 3Topic3D Geometry DescriptionDescription: This segment examines cylinders, using the Shanghai Tower as a case study. It highlights how adjustments to height and radius affect volume and surface area, demonstrating these principles with geometric calculations and their architectural applications in energy efficiency. |
3-Dimensional Figures, Cylinders and Applications of 3D Geometry |

|
Definition--3D Geometry Concepts--Cylinder | CylinderTopic3D Geometry DefinitionA cylinder is a three-dimensional geometric figure with two parallel circular bases connected by a curved surface at a fixed distance from each other. DescriptionIn the realm of three-dimensional geometry, a cylinder is a fundamental shape characterized by its two identical, parallel circular bases and a curved surface that connects these bases. The line segment joining the centers of the bases is called the axis of the cylinder, and it is perpendicular to the bases. The distance between the bases is the height of the cylinder, while the radius is the distance from the center to the edge of the base. |
Cylinders |
|
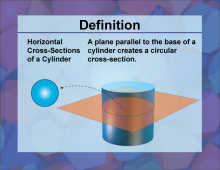
Definition--3D Geometry Concepts--Horizontal Cross-Sections of a Cylinder | Horizontal Cross Sections of a CylinderTopic3D Geometry DefinitionA horizontal cross-section of a cylinder is the intersection of the cylinder with a plane that is parallel to the base of the cylinder. DescriptionIn the context of three-dimensional geometry, understanding the concept of cross-sections is crucial. A cylinder is a three-dimensional shape with two parallel circular bases connected by a curved surface. When a horizontal plane intersects a cylinder, the resulting cross-section is a circle. This concept is not only fundamental in geometry but also has practical applications in various fields. |
Cylinders |
|
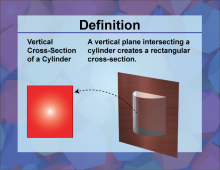
Definition--3D Geometry Concepts--Vertical Cross-Sections of a Cylinder | Vertical Cross Sections of a CylinderTopic3D Geometry DefinitionA vertical cross-section of a cylinder is the intersection of the cylinder with a plane that is parallel to its axis. This cross-section is typically a rectangle if the plane cuts through the entire height of the cylinder. |
Cylinders |
|
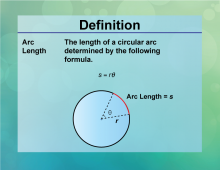
Definition--Circle Concepts--Arc Length | Arc LengthTopicCircles DefinitionAn arc length is the distance along the curved line making up the arc. DescriptionThe arc length is a crucial concept in geometry, particularly when dealing with circles. It is calculated using the formula L = rθ |
Definition of a Circle |
|
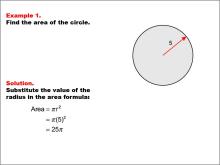
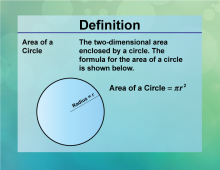
Definition--Circle Concepts--Area of a Circle | Area of a CircleTopicCircles DefinitionThe area of a circle is the space contained within its circumference, calculated as A = πr2 DescriptionThe area of a circle is a fundamental concept in geometry, representing the space enclosed by the circle's boundary. This concept is widely applicable in fields such as physics, engineering, and design, where understanding the area is crucial for tasks like calculating material quantities or designing circular components. The formula A = πr2 |
Definition of a Circle |
|
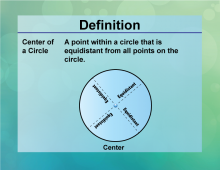
Definition--Circle Concepts--Center of a Circle | Center of a CircleTopicCircles DefinitionThe center of a circle is the point equidistant from all points on the circle. DescriptionThe center of a circle is a pivotal concept in geometry, serving as the reference point from which the radius is measured. It is crucial in defining the circle's position in a plane and is used in various applications such as navigation, where the center can represent a central point of rotation or balance. In mathematical terms, the center is often denoted as the point (h , k) in the Cartesian coordinate system, where all points on the circle satisfy the equation |
Definition of a Circle |

|
Definition--Circle Concepts--Chord | ChordTopicCircles DefinitionA chord is a line segment with both endpoints on the circle. |
Definition of a Circle |
|
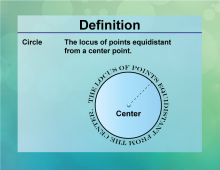
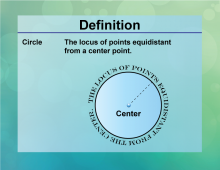
Definition--Circle Concepts--Circle | CircleTopicCircles DefinitionA circle is a set of all points in a plane equidistant from a given point, the center. DescriptionThe circle is one of the most fundamental shapes in geometry, characterized by its symmetry and uniformity. It is used extensively in various fields, including engineering, design, and astronomy, where its properties are applied to create wheels, gears, and orbits. Mathematically, a circle is defined by its center and radius, and its equation in a plane is (x − h)2 + (y − k)2 = r2 |
Definition of a Circle |

|
Definition--Circle Concepts--Circle Inscribed in a Square | Circle Inscribed in a SquareTopicCircles DefinitionA circle inscribed in a square touches all four sides of the square at exactly one point each. |
Definition of a Circle |
|
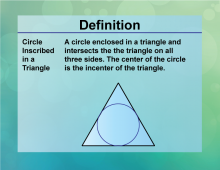
Definition--Circle Concepts--Circle Inscribed in a Triangle | Circle Inscribed in a TriangleTopicCircles DefinitionAn inscribed circle in a triangle is tangent to each of the triangle's sides. |
Definition of a Circle |
|
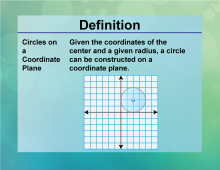
Definition--Circle Concepts--Circles on a Coordinate Plane | Circles on a Coordinate PlaneTopicCircles DefinitionCircles on a coordinate plane are defined by their center coordinates and radius. DescriptionUnderstanding circles on a coordinate plane is essential for analyzing geometric shapes in a mathematical context. This concept is widely used in computer graphics, navigation systems, and physics simulations, where precise positioning and movement of circular objects are required. The standard equation of a circle in the coordinate plane is (x − h)2 + (y − k)2 = r2 |
Definition of a Circle |
|
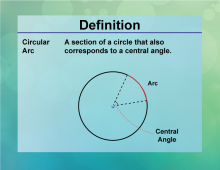
Definition--Circle Concepts--Circular Arc | Circular ArcTopicCircles DefinitionA circular arc is a portion of the circumference of a circle. DescriptionCircular arcs are segments of a circle's circumference, used extensively in design, architecture, and engineering to create curved structures and paths. The length of an arc is determined by the central angle and the circle's radius, calculated using the formula L = rθ |
Definition of a Circle |

|
Definition--Circle Concepts--Circular Composite Figures | Circular Composite FiguresTopicCircles DefinitionCircular composite figures are shapes that include circles or parts of circles combined with other geometric figures. |
Definition of a Circle |
|
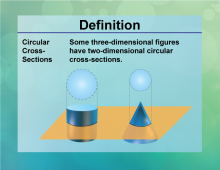
Definition--Circle Concepts--Circular Cross-Sections | Circular Cross-SectionsTopicCircles DefinitionCircular cross-sections are the intersections of a plane with a solid that result in a circle. |
Definition of a Circle |
|
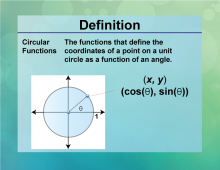
Definition--Circle Concepts--Circular Functions | Circular FunctionsTopicCircles DefinitionCircular functions are trigonometric functions that relate angles to ratios of sides in a right triangle. |
Definition of a Circle |

|
Definition--Circle Concepts--Circular Models | Circular ModelsTopicCircles DefinitionCircular models are representations of circular phenomena using mathematical equations and diagrams. |
Definition of a Circle |

|
Definition--Circle Concepts--Circular Numerical Models | Circular Numerical ModelsTopicCircles DefinitionCircular numerical models use numerical methods to analyze and simulate circular phenomena. |
Definition of a Circle |