
Illustrative Math Alignment: Grade 7 Unit 7
Angles, Triangles, and Prisms
Lesson 11: Slicing Solids
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Nodes |
|---|---|---|---|

|
VIDEO: 3D Geometry Animation: Antiprism | VIDEO: 3D Geometry Animation: Antiprism
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures and Triangular Prisms |

|
VIDEO: 3D Geometry Animation: Rectangular Prism | VIDEO: 3D Geometry Animation: Rectangular Prism
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures and Rectangular Prisms |

|
VIDEO: 3D Geometry Animation: Triangular Prism | VIDEO: 3D Geometry Animation: Triangular Prism
This is part of a series of video animations of three-dimensional figures. These animations show different views of these figures: top, side, and bottom. Many of these figures are a standard part of the geometry curriculum and being able to recognize them is important. — CLICK THE PREVIEW BUTTON TO SEE THE VIDEO ANIMATION —Study these animations to learn the basic properties of these 3D figures. In particular, make a note of their sides, edges, and vertices. Look for any symmetries they have. Look for polygon shapes that are familiar. Finally, think of real-world examples that use these figures. Below we also include information about Platonic solids and 2D nets of these 3D figures. To get a better understanding of these 3D figures, study these basic forms. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Antiprism with Horizontal Cross-Section | Antiprism with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows an antiprism with a horizontal cross-section, revealing its internal structure when sliced parallel to its bases. This visualization aids in understanding the spatial relationships and symmetry within the antiprism, making it a valuable teaching tool for discussing cross-sections and geometry. Using animated math clip art like this helps students grasp complex spatial concepts by breaking them down into visual components. Teachers can use this image to explain how cross-sections can provide insight into the internal geometry of polyhedra. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Antiprism with Vertical Cross-Section | Antiprism with Vertical Cross-SectionTopic3D Geometry DescriptionThis animation depicts an antiprism with a vertical cross-section, showing its internal structure when sliced perpendicular to its bases. This view is essential for understanding the vertical symmetry and arrangement of triangular faces, providing a deeper insight into the geometry of antiprisms. Animated math clip art like this is important for teaching as it allows students to visualize and explore the internal structures of complex shapes dynamically. Teachers can use this image to discuss how vertical cross-sections reveal different aspects of a shape's geometry. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Cone with Horizontal Cross-Section | Cone with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows a cone with a horizontal cross-section, illustrating how slicing the cone parallel to its base results in a circular cross-section. This concept is vital for understanding the properties of cones and is used in calculus to calculate volumes using integral methods. Using animated math clip art like this helps students visualize the concept of cross-sections and their applications in geometry and calculus. Teachers can use this image to explain how horizontal cross-sections reveal the internal structure of cones. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Cone with Vertical Cross-Section | Cone with Vertical Cross-SectionTopic3D Geometry DescriptionThis image depicts a vertical cross-section of a cone, showing a triangular section. This view is essential for understanding the symmetry and geometry of cones, as well as for solving problems involving conic sections and calculating the slant height. Animated math clip art like this is crucial for teaching as it provides a dynamic way to explore the internal geometry of cones. Teachers can use this image to discuss how vertical cross-sections reveal different aspects of a cone's structure. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Cube with Horizontal Cross-Section | Cube with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows a cube with a horizontal cross-section, illustrating how slicing the cube parallel to its base results in a square cross-section. This is useful for visualizing and understanding the internal structure and symmetry of cubes. Using animated math clip art like this helps students visualize the concept of cross-sections and their applications in geometry. Teachers can use this image to explain how horizontal cross-sections reveal the internal structure of cubes. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Cube with Vertical Cross-Section | Cube with Vertical Cross-SectionTopic3D Geometry DescriptionThis depiction of a cube with a vertical cross-section shows how slicing through the cube perpendicular to its base results in a square cross-section. This view helps in understanding the geometric properties and symmetry of cubes. Animated math clip art like this is crucial for teaching as it provides a dynamic way to explore the internal geometry of cubes. Teachers can use this image to discuss how vertical cross-sections reveal different aspects of a cube's structure. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Cylinder with Horizontal Cross-Section | Cylinder with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows a cylinder with a horizontal cross-section, illustrating how slicing the cylinder parallel to its bases results in a circular cross-section. This visualization is essential for understanding the internal structure and properties of cylinders. Using animated math clip art helps students grasp the concept of cross-sections, making it easier to understand how shapes are constructed. Teachers can use this image to explain the significance of horizontal cross-sections in geometry. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Cylinder with Vertical Cross-Section | Cylinder with Vertical Cross-SectionTopic3D Geometry DescriptionThis animation depicts a vertical cross-section of a cylinder, showing a rectangular section. This view is crucial for understanding the symmetry and geometry of cylinders, as well as for solving problems involving volume and surface area. Animated math clip art like this is important for teaching as it provides a dynamic way to explore the internal geometry of cylinders. Teachers can use this image to discuss how vertical cross-sections reveal different aspects of a cylinder's structure. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Dodecahedron with Horizontal Cross-Section | Dodecahedron with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows a dodecahedron with a horizontal cross-section, illustrating how slicing the shape parallel to its base reveals a pentagonal cross-section. This visualization helps in understanding the internal structure and symmetry of dodecahedrons. Using animated math clip art helps students visualize the concept of cross-sections, making it easier to understand how shapes are constructed. Teachers can use this image to explain the significance of horizontal cross-sections in polyhedra. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Dodecahedron with Vertical Cross-Section | Dodecahedron with Vertical Cross-SectionTopic3D Geometry DescriptionThis animation depicts a vertical cross-section of a dodecahedron, showing a section that reveals the internal arrangement of faces. This view is crucial for understanding the symmetry and geometry of dodecahedrons. Animated math clip art like this is important for teaching as it provides a dynamic way to explore the internal geometry of polyhedra. Teachers can use this image to discuss how vertical cross-sections reveal different aspects of a dodecahedron's structure. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Horizontal Cross-Section of a Rectangular Prism | Animated Math Clip Art: Horizontal Cross-Section of a Rectangular Prism This animated math clip art illustrates how a horizontal plane intersects a rectangular prism, forming a two-dimensional cross-section. As the plane moves through the prism, students can observe that the resulting cross-section is always a rectangle, reinforcing key geometric concepts related to slicing three-dimensional shapes. Ideal for classroom instruction, this GIF animation helps students develop spatial reasoning by demonstrating how horizontal slices interact with the structure of a rectangular prism. This visual aid is particularly useful for teaching cross-sections in geometry, emphasizing how different slicing directions produce distinct 2D shapes. |
Rectangular Prisms |

|
Animated Math Clip Art--3D Geometry--Icosahedron with Horizontal Cross-Section | Icosahedron with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows an icosahedron with a horizontal cross-section, illustrating how slicing the shape parallel to its base reveals a triangular cross-section. This visualization helps in understanding the internal structure and symmetry of icosahedrons. Using animated math clip art helps students visualize the concept of cross-sections, making it easier to understand how shapes are constructed. Teachers can use this image to explain the significance of horizontal cross-sections in polyhedra. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Icosahedron with Vertical Cross-Section | Icosahedron with Vertical Cross-SectionTopic3D Geometry DescriptionThis animation depicts a vertical cross-section of an icosahedron, revealing its internal structure. An icosahedron is a polyhedron with 20 triangular faces, and this cross-section helps visualize its complex geometry. Using animated math clip art like this is crucial for teaching as it allows students to see inside complex 3D shapes, enhancing their spatial reasoning skills. Teachers can use this image to discuss symmetry, polyhedra properties, and cross-sectional geometry. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Sphere with Horizontal Cross-Section | Sphere with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows a sphere with a horizontal cross-section. It illustrates that any plane intersecting a sphere creates a circular cross-section, with the largest circle (the great circle) occurring when the plane passes through the center of the sphere. Using animated math clip art like this helps students understand the internal structure of spheres. Teachers can use this to discuss concepts such as great circles, hemispheres, and how cross-sections relate to the volume of a sphere. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Sphere with Vertical Cross-Section | Sphere with Vertical Cross-SectionTopic3D Geometry DescriptionThis animation shows a sphere with a vertical cross-section. It illustrates that any plane intersecting a sphere creates a circular cross-section, with the largest circle (the great circle) occurring when the plane passes through the center of the sphere. Using animated math clip art like this helps students understand the internal structure of spheres from a different perspective. Teachers can use this to discuss concepts such as great circles, hemispheres, and how cross-sections relate to the volume of a sphere. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Square Pyramid Vertical Cross-Section | Square Pyramid Vertical Cross-SectionTopic3D Geometry DescriptionThis animation depicts a square pyramid with a vertical cross-section. It shows how slicing the pyramid vertically through its apex results in a triangular cross-section, revealing the internal structure and symmetry of the pyramid. Animated math clip art like this is valuable for teaching as it provides a different perspective on the internal geometry of pyramids. Teachers can use this to discuss concepts such as the slant height, symmetry, and how vertical cross-sections relate to the surface area of a pyramid. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Square Pyramid Horizontal Cross-Section | Square Pyramid Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows a square pyramid with a horizontal cross-section. It illustrates how slicing the pyramid parallel to its base results in a square cross-section that decreases in size as it moves towards the apex. Using animated math clip art like this helps students visualize the internal structure of pyramids. Teachers can use this to discuss concepts such as similarity, proportions, and how cross-sections relate to the volume of a pyramid. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Tetrahedron with Horizontal Cross-Section | Tetrahedron with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation depicts a tetrahedron with a horizontal cross-section. It shows how slicing the tetrahedron parallel to its base results in a triangular cross-section that decreases in size as it moves towards the apex. Using animated math clip art like this helps students understand the internal structure of complex 3D shapes. Teachers can use this to discuss concepts such as similarity, proportions, and how cross-sections relate to the volume of a tetrahedron. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Tetrahedron with Vertical Cross-Section | Tetrahedron with Vertical Cross-SectionTopic3D Geometry DescriptionThis animation shows a tetrahedron with a vertical cross-section. It illustrates how slicing the tetrahedron vertically through its apex results in a triangular cross-section, revealing the internal structure and symmetry of the shape. Animated math clip art like this is valuable for teaching as it provides a different perspective on the internal geometry of complex 3D shapes. Teachers can use this to discuss concepts such as symmetry, slant height, and how vertical cross-sections relate to the surface area of a tetrahedron. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Triangular Prism with Horizontal Cross-Section | Triangular Prism with Horizontal Cross-SectionTopic3D Geometry DescriptionThis animation shows a triangular prism with a horizontal cross-section. It illustrates that slicing the prism parallel to its base results in a rectangular cross-section that varies in size depending on the location of the slice. Animated math clip art like this helps students understand the internal structure of prisms. Teachers can use this to discuss concepts such as congruence, cross-sectional area, and how this relates to the volume of a prism. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Triangular Prism with Vertical Cross-Section | Triangular Prism with Vertical Cross-SectionTopic3D Geometry DescriptionThis animation depicts a triangular prism with a vertical cross-section. It shows how slicing the prism vertically results in a triangular cross-section, and all such triangles are congruent along the length of the prism. Using animated math clip art like this provides students with a different perspective on the internal geometry of prisms. Teachers can use this to discuss concepts such as height, lateral area, and how vertical cross-sections relate to the surface area of a prism. |
3-Dimensional Figures |

|
Animated Math Clip Art--3D Geometry--Vertical Cross-Section of a Rectangular Prism | Animated Math Clip Art: Vertical Cross-Section of a Rectangular Prism This animated math clip art visually demonstrates how a vertical plane intersects a rectangular prism, producing a two-dimensional cross-section. As the plane moves through the 3D shape, students can observe how the resulting cross-section remains a rectangle, reinforcing key geometric concepts related to slicing three-dimensional figures. Perfect for classroom instruction, this GIF animation helps students develop spatial reasoning by illustrating how a vertical slice interacts with the faces of a rectangular prism. This resource is particularly useful for teaching cross-sections in geometry and preparing students to understand more complex 3D slicing concepts. |
Rectangular Prisms |

|
Closed Captioned Video: Geometry Applications--Antiprisms | Closed Captioned Video: Geometry Applications--Antiprisms
In this video students learn the basics of antiprisms, in the context of New York’s Freedom Tower, which has an antiprism design. They learn the properties of antiprisms, with the focus on those with a square base. |
Rectangular Prisms |

|
Closed Captioned Video: Geometry Applications--Rectangular Prisms | Closed Captioned Video: Geometry Applications--Rectangular Prisms
In this video students learn the basics of rectangular prisms, in the context of a New York residential tower: 432 Park Avenue. They learn the properties of rectangular prisms, with and without square bases, and the architectural requirements that influence the use of both types of rectangular prisms. |
Rectangular Prisms |

|
Closed Captioned Video: Geometry Applications--Triangular Prisms | Closed Captioned Video: Geometry Applications--Triangular Prisms
In this video students study a real-world application of triangular prisms: The Flat Iron Building in New York City. This building is an ideal example of a real-world prism and also provides a tie-in to right triangle geometry. This video describes the geometry of the Flat Iron Building, as well as its architecture. It includes animations that demonstrate the key features of triangular prisms. |
Triangular Prisms |

|
Closed Captioned Video: Geometry Applications--What Are Prisms? | Closed Captioned Video: Geometry Applications--What Are Prisms?
In this video students learn the basics of prisms and anti prisms. They learn the properties of triangular prisms, rectangular prisms, triangular antiprisms, and rectangular antiprisms. |
Rectangular Prisms and Triangular Prisms |

|
Definition--3D Geometry Concepts--Cross-sections of a Cube | Cross Sections of a CubeTopic3D Geometry DefinitionA cross-section of a cube is the intersection of a plane with the cube, resulting in a two-dimensional shape. DescriptionIn the study of three-dimensional geometry, understanding the cross-sections of a cube is vital for visualizing how 3D objects interact with planes. When a plane intersects a cube, the resulting shape can vary depending on the angle and position of the plane. Common cross-sections include squares, rectangles, and triangles. |
Cubes |
|
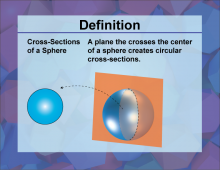
Definition--3D Geometry Concepts--Cross-Sections of a Sphere | Cross Sections of a SphereTopic3D Geometry DefinitionA cross-section of a sphere is the intersection of a plane with the sphere, resulting in a circle. DescriptionIn three-dimensional geometry, understanding the concept of cross-sections is crucial for visualizing and analyzing the properties of various 3D shapes. A sphere, which is a perfectly round geometrical object in three-dimensional space, can be intersected by a plane in various ways. When a plane cuts through a sphere, the shape of the intersection is always a circle. The size of this circle depends on the position of the plane relative to the center of the sphere. |
Spheres |
|
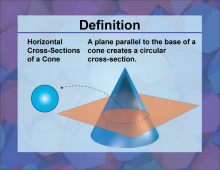
Definition--3D Geometry Concepts--Horizontal Cross-Sections of a Cone | Horizontal Cross-Sections of a ConeTopic3D Geometry DefinitionA horizontal cross-section of a cone is the circular shape obtained when a cone is intersected by a plane that is parallel to its base. |
Cones |
|
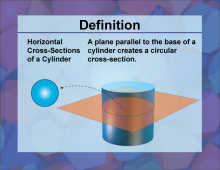
Definition--3D Geometry Concepts--Horizontal Cross-Sections of a Cylinder | Horizontal Cross Sections of a CylinderTopic3D Geometry DefinitionA horizontal cross-section of a cylinder is the intersection of the cylinder with a plane that is parallel to the base of the cylinder. DescriptionIn the context of three-dimensional geometry, understanding the concept of cross-sections is crucial. A cylinder is a three-dimensional shape with two parallel circular bases connected by a curved surface. When a horizontal plane intersects a cylinder, the resulting cross-section is a circle. This concept is not only fundamental in geometry but also has practical applications in various fields. |
Cylinders |
|
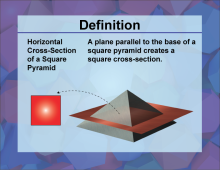
Definition--3D Geometry Concepts--Horizontal Cross-Sections of a Square Pyramid | Horizontal Cross Sections of a Square PyramidTopic3D Geometry. DefinitionA horizontal cross section of a square pyramid is a two-dimensional shape obtained by slicing the pyramid with a plane parallel to its base. |
Pyramids |
|
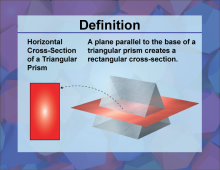
Definition--3D Geometry Concepts--Horizontal Cross-Sections of a Triangular Prism | Horizontal Cross Sections of a Triangular PrismTopic3D Geometry DefinitionA horizontal cross-section of a triangular prism is the shape obtained by cutting the prism with a plane parallel to its base. DescriptionIn three-dimensional geometry, a cross-section is the intersection of a plane with a solid figure. When this plane is parallel to the base of the solid, the resulting shape is called a horizontal cross-section. For a triangular prism with a rectangular base, this cross-section will always be a rectangle, similar in shape to the base of the prism. |
Triangular Prisms |
|
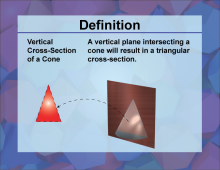
Definition--3D Geometry Concepts--Vertical Cross-Sections of a Cone | Vertical Cross Sections of a ConeTopic3D Geometry DefinitionA vertical cross section of a cone is the intersection of the cone with a plane that passes through its vertex and base, resulting in a two-dimensional shape. |
Cones |
|
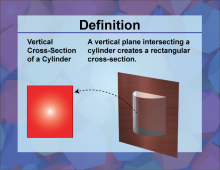
Definition--3D Geometry Concepts--Vertical Cross-Sections of a Cylinder | Vertical Cross Sections of a CylinderTopic3D Geometry DefinitionA vertical cross-section of a cylinder is the intersection of the cylinder with a plane that is parallel to its axis. This cross-section is typically a rectangle if the plane cuts through the entire height of the cylinder. |
Cylinders |
|
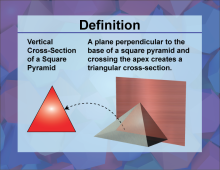
Definition--3D Geometry Concepts--Vertical Cross-Sections of a Square Pyramid | Vertical Cross Sections of a Square PyramidTopic3D Geometry DefinitionA vertical cross section of a square pyramid is the intersection of the pyramid with a vertical plane that passes through its apex and base, resulting in a two-dimensional shape. |
Pyramids |
|
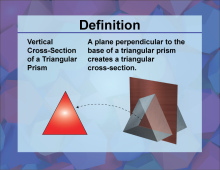
Definition--3D Geometry Concepts--Vertical Cross-Sections of a Triangular Prism | Vertical Cross Sections of a Triangular PrismTopic3D Geometry DefinitionA vertical cross section of a triangular prism is a two-dimensional shape obtained by slicing the prism parallel to its height, revealing a triangular face. |
Triangular Prisms |
|
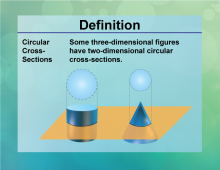
Definition--Circle Concepts--Circular Cross-Sections | Circular Cross-SectionsTopicCircles DefinitionCircular cross-sections are the intersections of a plane with a solid that result in a circle. |
Definition of a Circle |

|
Definition--Geometry Basics--Antiprism | AntiprismTopicGeometry Basics DefinitionA polyhedron with two parallel faces and other faces that are triangular. DescriptionAn antiprism is a type of polyhedron that has two parallel faces connected by an alternating band of triangles. This geometric shape is significant in advanced geometry and has applications in fields such as crystallography and molecular chemistry. Understanding antiprisms helps in comprehending the properties of polyhedra and their role in three-dimensional space. For example, the volume of an antiprism can be calculated using specific geometric formulas, making it a useful concept in various scientific and mathematical applications. |
3-Dimensional Figures |

|
Definition--Geometry Basics--Prism | PrismTopicGeometry Basics DefinitionA prism is a solid shape with two parallel, congruent bases connected by rectangular or parallelogram faces. DescriptionPrisms are significant in the study of three-dimensional shapes. They have a uniform cross-section, allowing for easy calculations of volume and surface area. The volume can be calculated with the formula V = B⋅h where B is the area of the base and h is the height. Prisms are commonly encountered in real-world applications such as packaging and structural designs, making them an essential concept in geometry. |
3-Dimensional Figures |
|
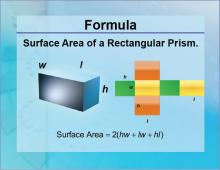
Formulas--Surface Area of a Rectangular Prism | Formulas--Surface Area of a Rectangular Prism
The formula for the Surface Area of a Rectangular Prism. This is part of a collection of math formulas. To see the complete collection of formulas, click on this link. Note: The download is a JPG file.Related ResourcesTo see resources related to this topic click on the Related Resources tab above. |
Surface Area |

|
Google Earth Voyager Story: Architectural Prisms, Part 1 | Google Earth Voyager Story: Architectural Prisms, Part 1TopicGeometric Models |
Triangular Prisms |

|
Google Earth Voyager Story: Architectural Prisms, Part 2 | Google Earth Voyager Story: Architectural Prisms, Part 2TopicGeometric Models |
Rectangular Prisms |

|
Google Earth Voyager Story: Architectural Prisms, Part 3 | Google Earth Voyager Story: Architectural Prisms, Part 3
In this Google Earth Voyager story visit architectural landmarks in New York City to learn about the geometry of prisms. In this lesson, visit the Freedom Tower in Lower Manhattan and learn about antiprisms. Then build a scale model of the Freedom Tower. This is part of a collection of Google Earth Voyager Stories that use Google's mapping technology to explore relevant math stories. To see the complete collection of the Google Earth Voyager Stories, click on this link. Note: The download is the teacher's guide.Viewing Media4Math Stories on Google EarthThese Google Earth Voyager Stories are also available on Google Earth. Best viewed in Chrome, click on the links below to view these dynamic stories using Google's technology: |
3-Dimensional Figures |

|
Lesson Plan: Cross-Sections of 3D Figures, Part 1 | Lesson Plan: Cross-Sections of 3D Figures In this engaging math lesson, students explore cross-sections of cubes, cylinders, and cones through interactive activities, real-world examples, and animated visuals. They will learn how slicing three-dimensional shapes in different ways produces a variety of two-dimensional figures, helping to develop spatial reasoning and geometric understanding. The lesson begins with a conceptual introduction to cross-sections, followed by hands-on practice with everyday objects such as gift boxes, paper towel rolls, and ice cream cones. Students will investigate how horizontal, vertical, and diagonal slices impact the resulting shapes. Animated GIFs illustrate these concepts, reinforcing learning through dynamic visual representations. |
3-Dimensional Figures |

|
Lesson Plan: Cross-Sections of 3D Figures, Part 2 | Lesson Plan: Cross-Sections of Rectangular Prisms, Spheres, and Pyramids In this engaging geometry lesson, students will explore how rectangular prisms, spheres, and pyramids are affected by different slicing planes. Using visual aids, real-world examples, and hands-on activities, students will identify the cross-sections created by horizontal, vertical, and diagonal cuts. Through interactive exploration, students will learn that a rectangular prism produces rectangular or parallelogram cross-sections, a sphere always results in a circular cross-section regardless of the slicing direction, and a pyramid creates triangles, trapezoids, or smaller versions of its base. The lesson also introduces the volume formula for a rectangular prism, reinforcing the connection between cross-sections and three-dimensional measurements. |
Applications of 3D Geometry |

|
Lesson Plan: Cross-Sections of 3D Figures, Part 3 | Lesson Plan: Cross-Sections of Triangular Prisms, Antiprisms, and Tetrahedrons In this engaging geometry lesson, students will explore cross-sections of triangular prisms, antiprisms, and tetrahedrons. Through hands-on activities, real-world examples, and animated visuals, students will investigate how different slicing planes impact three-dimensional figures. By analyzing horizontal, vertical, and diagonal cross-sections, students will develop a deeper understanding of geometric properties and spatial reasoning. |
Applications of 3D Geometry |
|
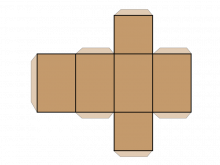
Math Clip Art--Net for a Rectangular Prism | Math Clip Art--Net for a Rectangular PrismTopicGeometry DescriptionThis math clip art image is part of a series showcasing 2D nets of 3D figures, specifically illustrating the net for a rectangular prism. The image displays a flattened representation of a rectangular prism, consisting of six connected rectangles arranged in a cross-like pattern. This visual aid is crucial for students to understand how a 3D rectangular prism can be unfolded into a 2D shape. |
3-Dimensional Figures |