
Illustrative Math Alignment: Grade 7 Unit 5
Rational Number Arithmetic
Lesson 8: Position, Speed, and Direction
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topics |
|---|---|---|---|

|
Math Example--Ratios and Rates--Example 17 | Math Example--Ratios and Rates--Example 17TopicRatios and Rates DescriptionThis example explores the concept of unit rates using the cost of gasoline. The image shows a red gasoline container, and students are asked to calculate the cost per gallon of gas given the total cost and volume. Understanding unit rates is a fundamental skill in mathematics with numerous real-world applications. This example demonstrates how to calculate a unit rate by dividing the total cost by the total quantity, illustrating the practical use of division in everyday scenarios like purchasing gasoline. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 18 | Math Example--Ratios and Rates--Example 18TopicRatios and Rates DescriptionThis example focuses on calculating unit rates in a restaurant context. The image shows a hamburger, and students are asked to determine the cost per pound of ground beef given the total cost and weight purchased. Understanding unit rates is essential in various real-world scenarios, particularly in business and economics. This example illustrates how to calculate a unit rate by dividing the total cost by the total quantity, demonstrating the practical application of division in a restaurant supply context. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 19 | Math Example--Ratios and Rates--Example 19TopicRatios and Rates DescriptionThis example explores the concept of flow rates using a backyard pool scenario. The image shows a backyard pool, and students are asked to calculate the rate of water flow in gallons per minute given the pool's capacity and the time taken to fill it. Understanding flow rates is important in various fields, including engineering and physics. This example demonstrates how to calculate a rate by dividing the total volume by the total time, illustrating the practical application of division in real-world scenarios involving fluid dynamics. |
Ratios and Rates |
|
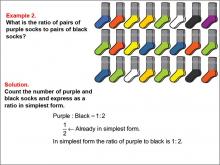
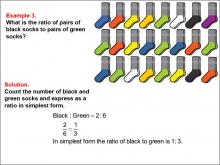
Math Example--Ratios and Rates--Example 2 | Math Example--Ratios and Rates--Example 2TopicRatios and Rates DescriptionThis example explores ratios using purple and black socks. The image shows a collection of socks, and students are asked to determine the ratio of purple socks to black socks. The solution reveals that there is 1 pair of purple socks and 2 pairs of black socks, resulting in a ratio of 1 : 2, which is already in its simplest form. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 20 | Math Example--Ratios and Rates--Example 20TopicRatios and Rates DescriptionThis example focuses on unit conversion using the height of the Statue of Liberty. The image shows the Statue of Liberty, and students are asked to convert its height from feet to inches. Understanding unit conversions is crucial in many fields, including science, engineering, and everyday life. This example demonstrates how to use a conversion factor to change units, illustrating the practical application of multiplication in real-world measurement scenarios. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 21 | Math Example--Ratios and Rates--Example 21TopicRatios and Rates DescriptionThis example focuses on unit conversion, specifically converting the height of the Statue of Liberty from feet to yards. The image shows the iconic Statue of Liberty, providing a real-world context for the mathematical problem. Understanding unit conversions is crucial in many fields, including engineering, science, and everyday life. This example demonstrates how to use a conversion factor to change units, illustrating the practical application of division and multiplication in real-world measurement scenarios. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 22 | Math Example--Ratios and Rates--Example 22TopicRatios and Rates DescriptionThis example explores unit conversion, specifically converting the height of the Statue of Liberty from feet to miles. The image depicts the Statue of Liberty, providing a tangible reference for the mathematical problem. Understanding unit conversions, especially between widely different scales like feet and miles, is important in various fields such as geography, engineering, and urban planning. This example showcases how to use a conversion factor to change units, demonstrating the practical application of division and multiplication in real-world measurement scenarios. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 23 | Math Example--Ratios and Rates--Example 23TopicRatios and Rates DescriptionThis example focuses on unit conversion, specifically converting the height of columns from inches to feet. The image shows columns, providing a visual context for the mathematical problem. Understanding unit conversions between inches and feet is crucial in many practical applications, including construction, interior design, and everyday measurements. This example demonstrates how to use a conversion factor to change units, illustrating the practical application of division and multiplication in real-world measurement scenarios. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 24 | Math Example--Ratios and Rates--Example 24TopicRatios and Rates DescriptionThis example explores unit conversion in a sports context, specifically converting a quarterback's pass distance from yards to feet. The image shows a silhouette of a quarterback throwing a football, providing a real-world scenario for the mathematical problem. Understanding unit conversions between yards and feet is crucial in many sports, especially American football. This example demonstrates how to use a conversion factor to change units, illustrating the practical application of multiplication in sports-related measurement scenarios. |
Ratios and Rates |
|
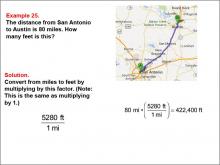
Math Example--Ratios and Rates--Example 25 | Math Example--Ratios and Rates--Example 25TopicRatios and Rates DescriptionThis example focuses on unit conversion in a geographic context, specifically converting the distance between San Antonio and Austin from miles to feet. The image shows a map of the route between these two Texas cities, providing a real-world scenario for the mathematical problem. Understanding unit conversions between miles and feet is important in various fields, including geography, transportation, and urban planning. This example demonstrates how to use a conversion factor to change units, illustrating the practical application of multiplication in distance-related measurement scenarios. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 26 | Math Example--Ratios and Rates--Example 26TopicRatios and Rates DescriptionThis example focuses on time unit conversion, specifically converting hours to minutes in the context of a standardized test duration. The image shows a clock, providing a visual representation of time for the mathematical problem. Understanding time unit conversions is crucial in many aspects of daily life, including scheduling, time management, and test-taking. This example demonstrates how to use a conversion factor to change units, illustrating the practical application of multiplication in time-related scenarios. |
Ratios and Rates |
|
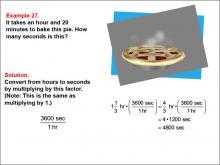
Math Example--Ratios and Rates--Example 27 | Math Example--Ratios and Rates--Example 27TopicRatios and Rates DescriptionThis example explores time unit conversion, specifically converting hours and minutes to seconds in the context of baking a pie. The image shows a steaming pie, providing a real-world scenario for the mathematical problem. Understanding time unit conversions, especially when dealing with mixed units, is important in various fields such as cooking, manufacturing, and project management. This example demonstrates how to use conversion factors to change units, illustrating the practical application of multiplication in time-related scenarios. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 28 | Math Example--Ratios and Rates--Example 28TopicRatios and Rates DescriptionThis example focuses on time unit conversion in a sports context, specifically converting minutes and seconds to seconds for a horse race lap time. The image shows a horse, providing a visual context for the mathematical problem. Understanding time unit conversions is crucial in many sports, especially those involving racing and timed events. This example demonstrates how to use conversion factors to change units and add different time units, illustrating the practical application of multiplication and addition in sports-related time scenarios. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 29 | Math Example--Ratios and Rates--Example 29TopicRatios and Rates DescriptionThis example explores time unit conversion in an IT context, specifically converting seconds to minutes for user session duration. The image shows a computer tower, providing a real-world scenario for the mathematical problem. Understanding time unit conversions is crucial in many technological fields, especially in IT and user experience analysis. This example demonstrates how to use a conversion factor to change units, illustrating the practical application of division in time-related measurement scenarios. It also introduces the concept of mixed numbers in the result. |
Ratios and Rates |
|
Math Example--Ratios and Rates--Example 3 | Math Example--Ratios and Rates--Example 3TopicRatios and Rates DescriptionThis example focuses on ratios using black and green socks. The image displays a collection of socks, and students are tasked with determining the ratio of black socks to green socks. The solution shows that there are 2 pairs of black socks and 6 pairs of green socks, resulting in a ratio of 2 : 6, which simplifies to 1 : 3. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 30 | Math Example--Ratios and Rates--Example 30TopicRatios and Rates DescriptionThis example focuses on time unit conversion in an IT context, specifically converting seconds to hours for user session duration. The image shows a computer tower, providing a real-world scenario for the mathematical problem. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 31 | Math Example--Ratios and Rates--Example 31TopicRatios and Rates DescriptionThis example focuses on temperature unit conversion, specifically converting Celsius to Fahrenheit for the boiling point of water. The image shows a beaker of boiling water, providing a visual context for the mathematical problem. Understanding temperature unit conversions is crucial in many scientific fields, including chemistry, physics, and meteorology. This example demonstrates how to use a conversion formula to change units, illustrating the practical application of mathematical equations in real-world temperature scenarios. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 32 | Math Example--Ratios and Rates--Example 32TopicRatios and Rates DescriptionThis example explores temperature unit conversion, specifically converting Fahrenheit to Celsius for the freezing point of water. The image shows icicles, providing a visual representation of the freezing temperature for the mathematical problem. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 4 | Math Example--Ratios and Rates--Example 4TopicRatios and Rates DescriptionThis example explores ratios using purple and red socks. The image shows a collection of socks, and students are asked to determine the ratio of purple socks to red socks. The solution reveals that there is 1 pair of purple socks and 4 pairs of red socks, resulting in a ratio of 1 : 4, which is already in its simplest form. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 5 | Math Example--Ratios and Rates--Example 5TopicRatios and Rates DescriptionThis example focuses on ratios using black and green socks among a variety of colored socks. The image displays pairs of socks in black, green, orange, yellow, blue, and red. Students are asked to determine the ratio of black socks to green socks. The solution shows that there are 2 pairs of black socks and 10 pairs of green socks, resulting in a ratio of 2 : 10, which simplifies to 1 : 5. |
Ratios and Rates |
|
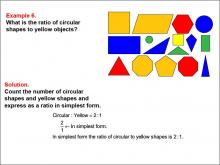
Math Example--Ratios and Rates--Example 6 | Math Example--Ratios and Rates--Example 6TopicRatios and Rates DescriptionThis example explores ratios using geometric shapes of different colors. The image displays various shapes in yellow, blue, red, and green. Students are asked to determine the ratio of circular shapes to yellow objects. The solution reveals that there are 2 circular shapes and 1 yellow object, resulting in a ratio of 2 : 1, which is already in its simplest form. |
Ratios and Rates |

|
Math Example--Ratios and Rates--Example 7 | Math Example--Ratios and Rates--Example 7TopicRatios and Rates DescriptionThis example focuses on ratios using geometric shapes. The image displays various shapes in yellow, blue, red, and green. Students are asked to determine the ratio of triangles to quadrilaterals. The solution shows that there are 4 triangles and 6 quadrilaterals, resulting in a ratio of 4 : 6, which simplifies to 2 : 3. |
Ratios and Rates |
|
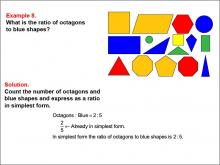
Math Example--Ratios and Rates--Example 8 | Math Example--Ratios and Rates--Example 8TopicRatios and Rates DescriptionThis example explores ratios using geometric shapes and colors. The image displays various shapes in yellow, blue, red, and green. Students are asked to determine the ratio of octagons to blue shapes. The solution reveals that there are 2 octagons and 5 blue shapes, resulting in a ratio of 2 : 5, which is already in its simplest form. |
Ratios and Rates |
|
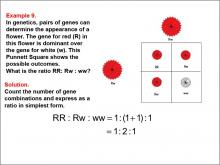
Math Example--Ratios and Rates--Example 9 | Math Example--Ratios and Rates--Example 9TopicRatios and Rates DescriptionThis example introduces the concept of ratios in genetics using a Punnett square. The image shows genetic combinations for a flower's color, including RR, Rw, and ww, with corresponding images of red and white flowers. Students are asked to determine the ratio of different gene combinations. |
Ratios and Rates |
|
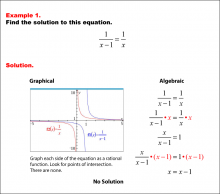
Math Example--Solving Equations--Extraneous Or No Solutions--Example 1 | Extraneous Or No Solutions--Example 1TopicEquations |
Radical Functions and Equations and Rational Functions and Equations |

|
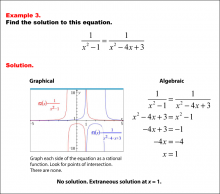
Math Example--Solving Equations--Extraneous Or No Solutions--Example 2 | Extraneous Or No Solutions--Example 2TopicEquations |
Radical Functions and Equations and Rational Functions and Equations |
|
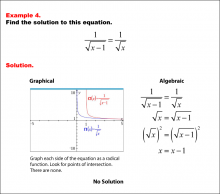
Math Example--Solving Equations--Extraneous Or No Solutions--Example 3 | Extraneous Or No Solutions--Example 3TopicEquations |
Radical Functions and Equations and Rational Functions and Equations |
|
Math Example--Solving Equations--Extraneous Or No Solutions--Example 4 | Extraneous Or No Solutions--Example 4TopicEquations |
Radical Functions and Equations and Rational Functions and Equations |

|
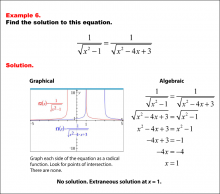
Math Example--Solving Equations--Extraneous Or No Solutions--Example 5 | Extraneous Or No Solutions--Example 5TopicEquations |
Radical Functions and Equations and Rational Functions and Equations |
|
Math Example--Solving Equations--Extraneous Or No Solutions--Example 6 | Extraneous Or No Solutions--Example 6TopicEquations |
Radical Functions and Equations and Rational Functions and Equations |

|
MATH EXAMPLES--Teacher's Guide: Graphs of Rational Functions | MATH EXAMPLES--Teacher's Guide: Graphs of Rational Functions
This Teacher's Guide provides an overview of the 28 worked-out examples that show how to graph Rational Functions. This is part of a collection of teacher's guides. To see the complete collection of teacher's guides, click on this link. Note: The download is a PDF file.Related ResourcesTo see resources related to this topic click on the Related Resources tab above. |
Rational Functions and Equations |

|
MATH EXAMPLES--Teacher's Guide: Rational Expressions | MATH EXAMPLES--Teacher's Guide: Rational Expressions
This Teacher's Guide provides an overview of the 28 worked-out examples that show how to simplify a variety of rational expressions. This is part of a collection of teacher's guides. To see the complete collection of teacher's guides, click on this link. Note: The download is a PDF file.Related ResourcesTo see resources related to this topic click on the Related Resources tab above. |
Rational Expressions and Rational Functions and Equations |

|
Math in the News: Issue 95--The Iditarod Race | Math in the News: Issue 95--The Iditarod Race
March 2014. In this issue of Math in the News we look at the Iditarod Race in Alaska. This gives us an opportunity to analyze data on average speed. We look at data in tables and line graphs and analyze the winning speeds over the history of the race. This is part of the Math in the News collection. To see the complete collection, click on this link. Note: The download is a PPT file.Related ResourcesTo see resources related to this topic click on the Related Resources tab above. |
Data Analysis |

|
Promethean Flipchart: Algebra Applications: Biology | All living things take up a certain amount of space, and therefore have volume. They also have a certain amount of surface area. The ratio of surface area to volume, which is a rational function, reveals important information about the organism. Students look at different graphs of these functions for different organisms. Note: The download for this resources is the Promethean Flipchart. To view the full video [Algebra Applications: Rational Functions, Segment 2: Biology]: https://www.media4math.com/library/algebra-applications-rational-functions-segment-2-biology |
Rational Functions and Equations |

|
Promethean Flipchart: Algebra Applications: Hubble | The Hubble Telescope, like all telescopes, relies on the lens formula to focus an image. The lens formula results in a rational equation that can be solved for determining the settings on the lens. Note: The download for this resources is the Promethean Flipchart. To access the full video [Algebra Applications: Rational Functions, Segment 3: Hubble Telescope]: https://www.media4math.com/library/algebra-applications-rational-functions-segment-3-hubble-telescope |
Rational Functions and Equations |

|
Promethean Flipchart: Algebra Applications: Submarines | In spite of their massive size, submarines are precision instruments. A submarine must withstand large amounts of water pressure; otherwise, a serious breach can occur. Rational functions are used to study the relationship between water pressure and volume. Students graph rational functions to study the forces at work with a submarine. Note: The download for this resources is the Promethean Flipchart. To access the full video [Algebra Applications: Rational Functions, Segment 1: Submarines]: https://www.media4math.com/library/algebra-applications-rational-functions-segment-1-submarines |
Rational Functions and Equations |

|
Promethean Flipchart: Algebra Nspirations: Rational Functions | After briefly reviewing the concept of inverse variation, this video explores Boyle’s law, a real world example of an inversely proportional relationship between pressure and volume of a gas. Written and hosted by internationally acclaimed math educator Dr. Monica Neagoy, it goes on to examine similarities and differences among rational functions and numbers. Finally, it takes a look at rational functions graphs and ends with a delightful example merging Euclidean and analytic geometry, thanks to the TI-Nspire technology. Concepts explored: functions, rational expressions, rational functions, asymptotes Note: The download for this resources is the Promethean Flipchart. To access the full video [Algebra Nspirations: Rational Functions and Expressions]: https://media4math.com/library/algebra-nspirations-rational-functions-and-expressions |
Rational Functions and Equations |

|
SAT Math Lesson Plan 9: Rates, Units, and Conversions | Lesson Plan: Rates, Units, and Conversions This 45-minute SAT Math lesson focuses on solving problems involving unit rates, dimensional analysis, and unit conversions. Students will learn how to calculate speed, cost per item, and population density, as well as how to convert between units using proportion and dimensional analysis. The lesson also includes multi-step word problems that combine rate calculations with percent-based reasoning—realistic scenarios frequently tested on the SAT. |
Ratios and Rates |

|
Video Definition 1--Rationals and Radicals--Addition of Rational Expressions | Video Definition 1--Rationals and Radicals--Addition of Rational Expressions
TopicRationals and Radicals DescriptionAddition of rational expressions involves combining rational expressions to create a new rational expression, often requiring a common denominator. Examples include 1 / x + 1 / 2x = 3 / 2x and 1 / (x + 1) + 1 / (x - 1) = 2x / (x2 - 1). This term introduces the concept of combining rational expressions, which is fundamental to operations involving fractions with polynomial expressions. |
Rational Functions and Equations |

|
Video Definition 1--Rationals and Radicals--Addition of Rational Expressions (Spanish Audio) | Video Definition 1--Rationals and Radicals--Addition of Rational Expressions (Spanish Audio)
TopicRationals and Radicals |
Rational Functions and Equations |
|
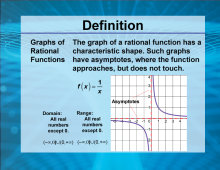
Video Definition 12--Rationals and Radicals--Graphs of Rational Functions | Video Definition 12--Rationals and Radicals--Graphs of Rational Functions
TopicRationals and Radicals DescriptionGraphs of rational functions often feature asymptotes, which are lines the graph approaches but never touches. For example, f(x) = 1 / x has vertical and horizontal asymptotes at x = 0 and y = 0, respectively. The domain and range exclude zero. Explains the unique characteristics of rational function graphs and the significance of asymptotes in defining their behavior. |
Rational Functions and Equations |

|
Video Definition 12--Rationals and Radicals--Graphs of Rational Functions (Spanish Audio) | Video Definition 12--Rationals and Radicals--Graphs of Rational Functions (Spanish Audio)
TopicRationals and Radicals DescriptionGraphs of rational functions often feature asymptotes, which are lines the graph approaches but never touches. For example, f(x) = 1 / x has vertical and horizontal asymptotes at x = 0 and y = 0, respectively. The domain and range exclude zero. Explains the unique characteristics of rational function graphs and the significance of asymptotes in defining their behavior. |
Rational Functions and Equations |
|
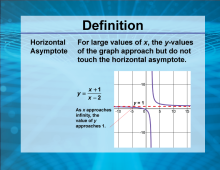
Video Definition 13--Rationals and Radicals--Horizontal Asymptote | Video Definition 13--Rationals and Radicals--Horizontal Asymptote
TopicRationals and Radicals DescriptionHorizontal asymptotes describe the behavior of a graph as x approaches infinity or negative infinity. For example, the equation y = (x + 1) / (x - 2) has a horizontal asymptote at y = 1, demonstrating long-term behavior of the function. Focuses on the role of horizontal asymptotes in describing the long-term trends of rational functions. |
Rational Functions and Equations |

|
Video Definition 13--Rationals and Radicals--Horizontal Asymptote (Spanish Audio) | Video Definition 13--Rationals and Radicals--Horizontal Asymptote (Spanish Audio)
TopicRationals and Radicals DescriptionHorizontal asymptotes describe the behavior of a graph as x approaches infinity or negative infinity. For example, the equation y = (x + 1) / (x - 2) has a horizontal asymptote at y = 1, demonstrating long-term behavior of the function. Focuses on the role of horizontal asymptotes in describing the long-term trends of rational functions. |
Rational Functions and Equations |

|
Video Definition 15--Rationals and Radicals--Integer | Video Definition 15--Rationals and Radicals--Integer
TopicRationals and Radicals DescriptionIntegers are a subset of rational numbers including positive, negative, and zero values, represented by {..., -3, -2, -1, 0, 1, 2, 3, ...}. This emphasizes their role in forming the basis of discrete mathematics. Introduces integers as a key component of number systems, aiding comprehension of rational numbers. |
Rational Functions and Equations |

|
Video Definition 15--Rationals and Radicals--Integer (Spanish Audio) | Video Definition 15--Rationals and Radicals--Integer (Spanish Audio)
TopicRationals and Radicals DescriptionIntegers are a subset of rational numbers including positive, negative, and zero values, represented by {..., -3, -2, -1, 0, 1, 2, 3, ...}. This emphasizes their role in forming the basis of discrete mathematics. Introduces integers as a key component of number systems, aiding comprehension of rational numbers. This video includes Spanish audio and can be used with multilingual learners. |
Rational Functions and Equations |
|
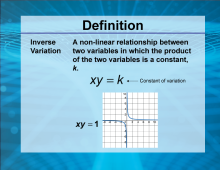
Video Definition 16--Rationals and Radicals--Inverse Variation | Video Definition 16--Rationals and Radicals--Inverse Variation
TopicRationals and Radicals DescriptionInverse variation describes a non-linear relationship where the product of two variables is a constant k. For instance, xy = k is graphically represented by a hyperbola, illustrating how one variable inversely depends on the other. Describes inverse variation as a key relationship in algebra, foundational for understanding non-linear equations. |
Rational Functions and Equations |

|
Video Definition 16--Rationals and Radicals--Inverse Variation (Spanish Audio) | Video Definition 16--Rationals and Radicals--Inverse Variation (Spanish Audio)
TopicRationals and Radicals DescriptionInverse variation describes a non-linear relationship where the product of two variables is a constant k. For instance, xy = k is graphically represented by a hyperbola, illustrating how one variable inversely depends on the other. Describes inverse variation as a key relationship in algebra, foundational for understanding non-linear equations. |
Rational Functions and Equations |

|
Video Definition 2--Rationals and Radicals--Asymptotes for a Rational Function | Video Definition 2--Rationals and Radicals--Asymptotes for a Rational Function
TopicRationals and Radicals DescriptionAsymptotes for a rational function are the values a function approaches but does not reach. An example is the function f(x) = 1 / x, where the graph has vertical and horizontal asymptotes at x = 0 and y = 0, respectively. This concept explains the behavior of rational functions at extreme values, helping understand their graphs and limits. |
Rational Functions and Equations |

|
Video Definition 2--Rationals and Radicals--Asymptotes for a Rational Function (Spanish Audio) | Video Definition 2--Rationals and Radicals--Asymptotes for a Rational Function (Spanish Audio)
TopicRationals and Radicals DescriptionAsymptotes for a rational function are the values a function approaches but does not reach. An example is the function f(x) = 1 / x, where the graph has vertical and horizontal asymptotes at x = 0 and y = 0, respectively. This concept explains the behavior of rational functions at extreme values, helping understand their graphs and limits. |
Rational Functions and Equations |