
Illustrative Math Alignment: Grade 8 Unit 3
Linear Relationships
Lesson 5: Introduction to Linear Relationships
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|
|
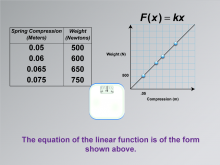
Math Clip Art--Applications of Linear Functions: Hooke's Law 06 | Math Clip Art--Applications of Linear Functions: Hooke's Law 06TopicLinear Functions DescriptionThis image is part of a series demonstrating applications of linear functions, specifically focusing on Hooke's Law. It builds upon the previous images by introducing the general form of the linear function equation that describes the relationship observed in the weight scale experiment. |
Graphs of Linear Functions, Slope-Intercept Form and Proportions |

|
Math Clip Art--Applications of Linear Functions: Hooke's Law 06 | Math Clip Art--Applications of Linear Functions: Hooke's Law 06TopicLinear Functions DescriptionThis image is part of a series demonstrating applications of linear functions, specifically focusing on Hooke's Law. It builds upon the previous images by introducing the general form of the linear function equation that describes the relationship observed in the weight scale experiment. |
Graphs of Linear Functions, Slope-Intercept Form and Proportions |
|
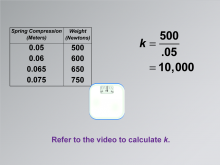
Math Clip Art--Applications of Linear Functions: Hooke's Law 07 | Math Clip Art--Applications of Linear Functions: Hooke's Law 07TopicLinear Functions DescriptionThis image is part of a series illustrating applications of linear functions, focusing on Hooke's Law. It builds upon previous images by demonstrating how to calculate the spring constant 'k' using the data collected from the weight scale experiment. Note: Here is the link to the video referenced: https://www.media4math.com/library/42143/asset-preview |
Graphs of Linear Functions, Slope-Intercept Form and Proportions |

|
Math Clip Art--Applications of Linear Functions: Hooke's Law 07 | Math Clip Art--Applications of Linear Functions: Hooke's Law 07TopicLinear Functions DescriptionThis image is part of a series illustrating applications of linear functions, focusing on Hooke's Law. It builds upon previous images by demonstrating how to calculate the spring constant 'k' using the data collected from the weight scale experiment. Note: Here is the link to the video referenced: https://www.media4math.com/library/42143/asset-preview |
Graphs of Linear Functions, Slope-Intercept Form and Proportions |

|
Math Clip Art--Applications of Linear Functions: Hooke's Law 07 | Math Clip Art--Applications of Linear Functions: Hooke's Law 07TopicLinear Functions DescriptionThis image is part of a series illustrating applications of linear functions, focusing on Hooke's Law. It builds upon previous images by demonstrating how to calculate the spring constant 'k' using the data collected from the weight scale experiment. Note: Here is the link to the video referenced: https://www.media4math.com/library/42143/asset-preview |
Graphs of Linear Functions, Slope-Intercept Form and Proportions |
|
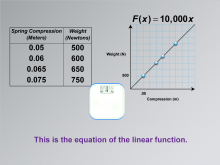
Math Clip Art--Applications of Linear Functions: Hooke's Law 08 | Math Clip Art--Applications of Linear Functions: Hooke's Law 08TopicLinear Functions DescriptionThis image represents the culmination of our exploration into applications of linear functions, specifically focusing on Hooke's Law. It serves as the final piece in a series of eight images, tying together all the concepts we've examined throughout this study of spring behavior and weight measurement. |
Graphs of Linear Functions, Slope-Intercept Form and Proportions |

|
Math Clip Art--Applications of Linear Functions: Hooke's Law 08 | Math Clip Art--Applications of Linear Functions: Hooke's Law 08TopicLinear Functions DescriptionThis image represents the culmination of our exploration into applications of linear functions, specifically focusing on Hooke's Law. It serves as the final piece in a series of eight images, tying together all the concepts we've examined throughout this study of spring behavior and weight measurement. |
Graphs of Linear Functions, Slope-Intercept Form and Proportions |

|
Math Clip Art--Applications of Linear Functions: Hooke's Law 08 | Math Clip Art--Applications of Linear Functions: Hooke's Law 08TopicLinear Functions DescriptionThis image represents the culmination of our exploration into applications of linear functions, specifically focusing on Hooke's Law. It serves as the final piece in a series of eight images, tying together all the concepts we've examined throughout this study of spring behavior and weight measurement. |
Graphs of Linear Functions, Slope-Intercept Form and Proportions |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 01 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 01TopicLinear Functions DescriptionThis image serves as an introduction to a series of math clip art illustrations demonstrating applications of linear functions, specifically focusing on temperature conversion. The title card presents "Applications of Linear Functions: Temperature Conversion," setting the stage for a comprehensive exploration of how linear models can be used to convert between Celsius and Fahrenheit temperatures. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 01 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 01TopicLinear Functions DescriptionThis image serves as an introduction to a series of math clip art illustrations demonstrating applications of linear functions, specifically focusing on temperature conversion. The title card presents "Applications of Linear Functions: Temperature Conversion," setting the stage for a comprehensive exploration of how linear models can be used to convert between Celsius and Fahrenheit temperatures. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 01 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 01TopicLinear Functions DescriptionThis image serves as an introduction to a series of math clip art illustrations demonstrating applications of linear functions, specifically focusing on temperature conversion. The title card presents "Applications of Linear Functions: Temperature Conversion," setting the stage for a comprehensive exploration of how linear models can be used to convert between Celsius and Fahrenheit temperatures. |
Graphs of Linear Functions and Slope-Intercept Form |
|
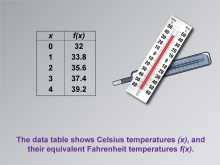
Math Clip Art--Applications of Linear Functions: Temperature Conversion 02 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 02TopicLinear Functions DescriptionThis image is the second in a series illustrating applications of linear functions, focusing on temperature conversion. It presents a visual representation of thermometers alongside a data table that displays Celsius temperatures (x) and their corresponding Fahrenheit temperatures f(x). This pairing of visual and tabular data helps students connect the abstract concept of linear functions to the concrete idea of temperature scales. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 02 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 02TopicLinear Functions DescriptionThis image is the second in a series illustrating applications of linear functions, focusing on temperature conversion. It presents a visual representation of thermometers alongside a data table that displays Celsius temperatures (x) and their corresponding Fahrenheit temperatures f(x). This pairing of visual and tabular data helps students connect the abstract concept of linear functions to the concrete idea of temperature scales. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 02 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 02TopicLinear Functions DescriptionThis image is the second in a series illustrating applications of linear functions, focusing on temperature conversion. It presents a visual representation of thermometers alongside a data table that displays Celsius temperatures (x) and their corresponding Fahrenheit temperatures f(x). This pairing of visual and tabular data helps students connect the abstract concept of linear functions to the concrete idea of temperature scales. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 03 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 03TopicLinear Functions DescriptionThis image is the third in a series demonstrating applications of linear functions through temperature conversion. It builds upon the previous image by highlighting a key observation: for every degree Celsius increase, there is a corresponding 1.8° increase in the Fahrenheit temperature. This insight is crucial in understanding the linear relationship between Celsius and Fahrenheit scales. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 03 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 03TopicLinear Functions DescriptionThis image is the third in a series demonstrating applications of linear functions through temperature conversion. It builds upon the previous image by highlighting a key observation: for every degree Celsius increase, there is a corresponding 1.8° increase in the Fahrenheit temperature. This insight is crucial in understanding the linear relationship between Celsius and Fahrenheit scales. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 03 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 03TopicLinear Functions DescriptionThis image is the third in a series demonstrating applications of linear functions through temperature conversion. It builds upon the previous image by highlighting a key observation: for every degree Celsius increase, there is a corresponding 1.8° increase in the Fahrenheit temperature. This insight is crucial in understanding the linear relationship between Celsius and Fahrenheit scales. |
Graphs of Linear Functions and Slope-Intercept Form |
|
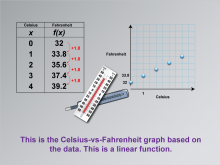
Math Clip Art--Applications of Linear Functions: Temperature Conversion 04 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 04TopicLinear Functions DescriptionThis image is part of a series illustrating applications of linear functions, focusing on temperature conversion. It builds upon previous images by introducing a data graph that visually represents the relationship between Celsius and Fahrenheit temperatures. The graph reinforces the key observation that for every degree Celsius increase, there is a corresponding 1.8° increase in the Fahrenheit temperature. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 04 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 04TopicLinear Functions DescriptionThis image is part of a series illustrating applications of linear functions, focusing on temperature conversion. It builds upon previous images by introducing a data graph that visually represents the relationship between Celsius and Fahrenheit temperatures. The graph reinforces the key observation that for every degree Celsius increase, there is a corresponding 1.8° increase in the Fahrenheit temperature. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 04 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 04TopicLinear Functions DescriptionThis image is part of a series illustrating applications of linear functions, focusing on temperature conversion. It builds upon previous images by introducing a data graph that visually represents the relationship between Celsius and Fahrenheit temperatures. The graph reinforces the key observation that for every degree Celsius increase, there is a corresponding 1.8° increase in the Fahrenheit temperature. |
Graphs of Linear Functions and Slope-Intercept Form |
|
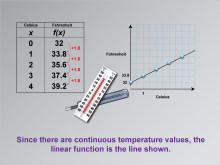
Math Clip Art--Applications of Linear Functions: Temperature Conversion 05 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 05TopicLinear Functions DescriptionThis image continues the series on applications of linear functions in temperature conversion. It builds upon the previous graph by connecting the data points with a straight line. This visual representation emphasizes the continuous nature of temperature values and clearly illustrates the linear relationship between Celsius and Fahrenheit scales. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 05 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 05TopicLinear Functions DescriptionThis image continues the series on applications of linear functions in temperature conversion. It builds upon the previous graph by connecting the data points with a straight line. This visual representation emphasizes the continuous nature of temperature values and clearly illustrates the linear relationship between Celsius and Fahrenheit scales. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 05 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 05TopicLinear Functions DescriptionThis image continues the series on applications of linear functions in temperature conversion. It builds upon the previous graph by connecting the data points with a straight line. This visual representation emphasizes the continuous nature of temperature values and clearly illustrates the linear relationship between Celsius and Fahrenheit scales. |
Graphs of Linear Functions and Slope-Intercept Form |

|
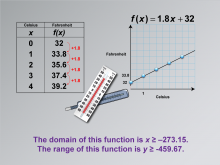
Math Clip Art--Applications of Linear Functions: Temperature Conversion 06 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 06TopicLinear Functions DescriptionThis image is a crucial part of the series on applications of linear functions in temperature conversion. It introduces the mathematical equation f(x) = 1.8x + 32, which represents the temperature conversion function from Celsius to Fahrenheit. This equation is the culmination of the observations and data analysis from the previous images in the series. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 06 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 06TopicLinear Functions DescriptionThis image is a crucial part of the series on applications of linear functions in temperature conversion. It introduces the mathematical equation f(x) = 1.8x + 32, which represents the temperature conversion function from Celsius to Fahrenheit. This equation is the culmination of the observations and data analysis from the previous images in the series. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 06 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 06TopicLinear Functions DescriptionThis image is a crucial part of the series on applications of linear functions in temperature conversion. It introduces the mathematical equation f(x) = 1.8x + 32, which represents the temperature conversion function from Celsius to Fahrenheit. This equation is the culmination of the observations and data analysis from the previous images in the series. |
Graphs of Linear Functions and Slope-Intercept Form |
|
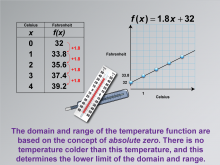
Math Clip Art--Applications of Linear Functions: Temperature Conversion 07 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 07TopicLinear Functions DescriptionThis image continues the exploration of linear functions in temperature conversion by introducing the concepts of domain and range. It specifies that the domain of the temperature conversion function is x ≥ -273.15, and the range is y ≥ -459.67. These limits are crucial in understanding the real-world constraints on the mathematical model. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 07 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 07TopicLinear Functions DescriptionThis image continues the exploration of linear functions in temperature conversion by introducing the concepts of domain and range. It specifies that the domain of the temperature conversion function is x ≥ -273.15, and the range is y ≥ -459.67. These limits are crucial in understanding the real-world constraints on the mathematical model. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 07 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 07TopicLinear Functions DescriptionThis image continues the exploration of linear functions in temperature conversion by introducing the concepts of domain and range. It specifies that the domain of the temperature conversion function is x ≥ -273.15, and the range is y ≥ -459.67. These limits are crucial in understanding the real-world constraints on the mathematical model. |
Graphs of Linear Functions and Slope-Intercept Form |
|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 08 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 08TopicLinear Functions DescriptionThis image delves deeper into the concept of domain and range in the context of temperature conversion. It explains that the limits of the temperature function are based on the concept of absolute zero, the lowest theoretically possible temperature. This fundamental principle of physics determines the lower bounds of both the domain and range of the temperature conversion function. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 08 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 08TopicLinear Functions DescriptionThis image delves deeper into the concept of domain and range in the context of temperature conversion. It explains that the limits of the temperature function are based on the concept of absolute zero, the lowest theoretically possible temperature. This fundamental principle of physics determines the lower bounds of both the domain and range of the temperature conversion function. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 08 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 08TopicLinear Functions DescriptionThis image delves deeper into the concept of domain and range in the context of temperature conversion. It explains that the limits of the temperature function are based on the concept of absolute zero, the lowest theoretically possible temperature. This fundamental principle of physics determines the lower bounds of both the domain and range of the temperature conversion function. |
Graphs of Linear Functions and Slope-Intercept Form |
|
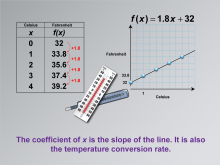
Math Clip Art--Applications of Linear Functions: Temperature Conversion 09 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 09TopicLinear Functions DescriptionThis final image in the series on applications of linear functions in temperature conversion focuses on the significance of the coefficient of x in the linear equation. It emphasizes that this coefficient, 1.8 in the equation f(x) = 1.8x + 32, represents both the slope of the line and the temperature conversion rate. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 09 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 09TopicLinear Functions DescriptionThis final image in the series on applications of linear functions in temperature conversion focuses on the significance of the coefficient of x in the linear equation. It emphasizes that this coefficient, 1.8 in the equation f(x) = 1.8x + 32, represents both the slope of the line and the temperature conversion rate. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Applications of Linear Functions: Temperature Conversion 09 | Math Clip Art--Applications of Linear Functions: Temperature Conversion 09TopicLinear Functions DescriptionThis final image in the series on applications of linear functions in temperature conversion focuses on the significance of the coefficient of x in the linear equation. It emphasizes that this coefficient, 1.8 in the equation f(x) = 1.8x + 32, represents both the slope of the line and the temperature conversion rate. |
Graphs of Linear Functions and Slope-Intercept Form |
|
Math Clip Art--Linear Function Tables 01 | Math Clip Art--Linear Function Concepts--Linear Function Tables 01TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, specifically focusing on linear function tables. It serves as a title card for a set of 10 clip art images that explore linear functions in tabular and graph form. This visual introduction sets the stage for understanding how linear functions can be represented and analyzed using tables. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 01 | Math Clip Art--Linear Function Concepts--Linear Function Tables 01TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, specifically focusing on linear function tables. It serves as a title card for a set of 10 clip art images that explore linear functions in tabular and graph form. This visual introduction sets the stage for understanding how linear functions can be represented and analyzed using tables. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 01 | Math Clip Art--Linear Function Concepts--Linear Function Tables 01TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, specifically focusing on linear function tables. It serves as a title card for a set of 10 clip art images that explore linear functions in tabular and graph form. This visual introduction sets the stage for understanding how linear functions can be represented and analyzed using tables. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 01 | Math Clip Art--Linear Function Concepts--Linear Function Tables 01TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, specifically focusing on linear function tables. It serves as a title card for a set of 10 clip art images that explore linear functions in tabular and graph form. This visual introduction sets the stage for understanding how linear functions can be represented and analyzed using tables. |
Graphs of Linear Functions and Slope-Intercept Form |
|
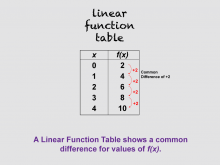
Math Clip Art--Linear Function Tables 02 | Math Clip Art--Linear Function Concepts--Linear Function Tables 02TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, focusing on linear function tables. It presents a data table demonstrating that a linear function table shows a common difference for values of f(x). This visual representation helps students understand the fundamental characteristic of linear functions - the constant rate of change. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 02 | Math Clip Art--Linear Function Concepts--Linear Function Tables 02TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, focusing on linear function tables. It presents a data table demonstrating that a linear function table shows a common difference for values of f(x). This visual representation helps students understand the fundamental characteristic of linear functions - the constant rate of change. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 02 | Math Clip Art--Linear Function Concepts--Linear Function Tables 02TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, focusing on linear function tables. It presents a data table demonstrating that a linear function table shows a common difference for values of f(x). This visual representation helps students understand the fundamental characteristic of linear functions - the constant rate of change. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 02 | Math Clip Art--Linear Function Concepts--Linear Function Tables 02TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, focusing on linear function tables. It presents a data table demonstrating that a linear function table shows a common difference for values of f(x). This visual representation helps students understand the fundamental characteristic of linear functions - the constant rate of change. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 03 | Math Clip Art--Linear Function Concepts--Linear Function Tables 03TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, specifically linear function tables. It builds on the previous image by showing that the common difference in a linear function can also be negative. This concept is crucial for understanding decreasing linear functions and their representation in tabular form. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 03 | Math Clip Art--Linear Function Concepts--Linear Function Tables 03TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, specifically linear function tables. It builds on the previous image by showing that the common difference in a linear function can also be negative. This concept is crucial for understanding decreasing linear functions and their representation in tabular form. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 03 | Math Clip Art--Linear Function Concepts--Linear Function Tables 03TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, specifically linear function tables. It builds on the previous image by showing that the common difference in a linear function can also be negative. This concept is crucial for understanding decreasing linear functions and their representation in tabular form. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 03 | Math Clip Art--Linear Function Concepts--Linear Function Tables 03TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, specifically linear function tables. It builds on the previous image by showing that the common difference in a linear function can also be negative. This concept is crucial for understanding decreasing linear functions and their representation in tabular form. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 04 | Math Clip Art--Linear Function Concepts--Linear Function Tables 04TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, focusing on linear function tables. It presents a new version of the previous table, this time demonstrating that the common difference can also be zero. This concept introduces students to constant functions, a special case of linear functions where the output remains the same regardless of the input. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 04 | Math Clip Art--Linear Function Concepts--Linear Function Tables 04TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, focusing on linear function tables. It presents a new version of the previous table, this time demonstrating that the common difference can also be zero. This concept introduces students to constant functions, a special case of linear functions where the output remains the same regardless of the input. |
Graphs of Linear Functions and Slope-Intercept Form |

|
Math Clip Art--Linear Function Tables 04 | Math Clip Art--Linear Function Concepts--Linear Function Tables 04TopicLinear Functions DescriptionThis image is part of a series illustrating key concepts in linear functions, focusing on linear function tables. It presents a new version of the previous table, this time demonstrating that the common difference can also be zero. This concept introduces students to constant functions, a special case of linear functions where the output remains the same regardless of the input. |
Graphs of Linear Functions and Slope-Intercept Form |