
Illustrative Math Alignment: Grade 8 Unit 3
Linear Relationships
Lesson 9: Slopes Don't Have to be Positive
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|
|
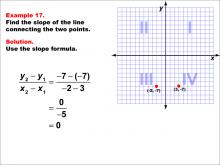
Math Example--Coordinate Geometry--Slope Formula: Example 17 | Math Example--Coordinate Geometry--Slope Formula: Example 17TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a horizontal line connecting points (-2, -7) and (3, -7) on a Cartesian plane. When we apply the slope formula, we find that the slope is (-7 - (-7)) / (-2 - 3) = 0 / -5 = 0. The slope formula is a key concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is horizontal, resulting in a slope of zero, even when the points have different x-coordinates. |
Slope |
|
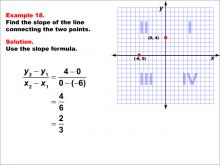
Math Example--Coordinate Geometry--Slope Formula: Example 18 | Math Example--Coordinate Geometry--Slope Formula: Example 18TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a line connecting two points: (-6, 0) and (0, 4) on a Cartesian plane. Applying the slope formula, we find that the slope is (4 - 0) / (0 - (-6)) = 4 / 6 = 2 / 3. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This example shows how to handle points with both positive and negative coordinates when calculating slope, resulting in a positive fraction. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 18 | Math Example--Coordinate Geometry--Slope Formula: Example 18TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a line connecting two points: (-6, 0) and (0, 4) on a Cartesian plane. Applying the slope formula, we find that the slope is (4 - 0) / (0 - (-6)) = 4 / 6 = 2 / 3. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This example shows how to handle points with both positive and negative coordinates when calculating slope, resulting in a positive fraction. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 19 | Math Example--Coordinate Geometry--Slope Formula: Example 19TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a line connecting two points: (1, 0) and (0, 6) on a Cartesian plane. Applying the slope formula, we find that the slope is (6 - 0) / (0 - 1) = 6 / -1 = -6. The slope formula is a crucial concept in coordinate geometry, helping us understand the steepness and direction of lines. This example demonstrates how to handle points with both positive and negative coordinates when calculating slope, resulting in a negative integer slope. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 19 | Math Example--Coordinate Geometry--Slope Formula: Example 19TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a line connecting two points: (1, 0) and (0, 6) on a Cartesian plane. Applying the slope formula, we find that the slope is (6 - 0) / (0 - 1) = 6 / -1 = -6. The slope formula is a crucial concept in coordinate geometry, helping us understand the steepness and direction of lines. This example demonstrates how to handle points with both positive and negative coordinates when calculating slope, resulting in a negative integer slope. |
Slope |
|
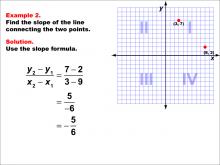
Math Example--Coordinate Geometry--Slope Formula: Example 2 | Math Example--Coordinate Geometry--Slope Formula: Example 2TopicSlope Formula DescriptionThis example illustrates the calculation of slope between two points (3, 7) and (9, 2) on a coordinate grid. The slope formula is applied to find that the slope is (7 - 2) / (3 - 9) = 5 / -6 = -5 / 6. Understanding the slope formula is crucial in coordinate geometry as it helps describe the steepness and direction of a line. This concept is widely used in various mathematical applications and real-world scenarios. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 2 | Math Example--Coordinate Geometry--Slope Formula: Example 2TopicSlope Formula DescriptionThis example illustrates the calculation of slope between two points (3, 7) and (9, 2) on a coordinate grid. The slope formula is applied to find that the slope is (7 - 2) / (3 - 9) = 5 / -6 = -5 / 6. Understanding the slope formula is crucial in coordinate geometry as it helps describe the steepness and direction of a line. This concept is widely used in various mathematical applications and real-world scenarios. |
Slope |
|
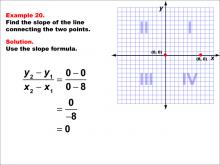
Math Example--Coordinate Geometry--Slope Formula: Example 20 | Math Example--Coordinate Geometry--Slope Formula: Example 20TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a horizontal line connecting points (0, 0) and (8, 0) on a Cartesian plane. When we apply the slope formula, we find that the slope is (0 - 0) / (8 - 0) = 0 / 8 = 0. The slope formula is a key concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is horizontal and lies on the x-axis, resulting in a slope of zero. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 20 | Math Example--Coordinate Geometry--Slope Formula: Example 20TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a horizontal line connecting points (0, 0) and (8, 0) on a Cartesian plane. When we apply the slope formula, we find that the slope is (0 - 0) / (8 - 0) = 0 / 8 = 0. The slope formula is a key concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is horizontal and lies on the x-axis, resulting in a slope of zero. |
Slope |
|
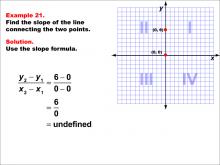
Math Example--Coordinate Geometry--Slope Formula: Example 21 | Math Example--Coordinate Geometry--Slope Formula: Example 21TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a vertical line connecting points (0, 0) and (0, 6) on a Cartesian plane. When we apply the slope formula, we find that the slope is (6 - 0) / (0 - 0) = 6 / 0, which is undefined. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is vertical and lies on the y-axis, resulting in an undefined slope due to division by zero. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 21 | Math Example--Coordinate Geometry--Slope Formula: Example 21TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a vertical line connecting points (0, 0) and (0, 6) on a Cartesian plane. When we apply the slope formula, we find that the slope is (6 - 0) / (0 - 0) = 6 / 0, which is undefined. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is vertical and lies on the y-axis, resulting in an undefined slope due to division by zero. |
Slope |
|
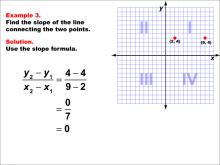
Math Example--Coordinate Geometry--Slope Formula: Example 3 | Math Example--Coordinate Geometry--Slope Formula: Example 3TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a horizontal line connecting points (2, 4) and (9, 4) on a coordinate grid. When we apply the slope formula, we find that the slope is (4 - 4) / (9 - 2) = 0 / 7 = 0. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is horizontal, resulting in a slope of zero. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 3 | Math Example--Coordinate Geometry--Slope Formula: Example 3TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a horizontal line connecting points (2, 4) and (9, 4) on a coordinate grid. When we apply the slope formula, we find that the slope is (4 - 4) / (9 - 2) = 0 / 7 = 0. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is horizontal, resulting in a slope of zero. |
Slope |
|
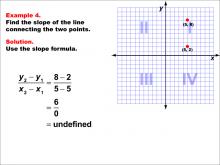
Math Example--Coordinate Geometry--Slope Formula: Example 4 | Math Example--Coordinate Geometry--Slope Formula: Example 4TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a vertical line passing through points (5, 8) and (5, 2) on a coordinate grid. When we apply the slope formula, we find that the slope is (8 - 2) / (5 - 5) = 6 / 0, which is undefined. The slope formula is a key concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is vertical, resulting in an undefined slope. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 4 | Math Example--Coordinate Geometry--Slope Formula: Example 4TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a vertical line passing through points (5, 8) and (5, 2) on a coordinate grid. When we apply the slope formula, we find that the slope is (8 - 2) / (5 - 5) = 6 / 0, which is undefined. The slope formula is a key concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is vertical, resulting in an undefined slope. |
Slope |
|
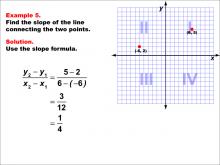
Math Example--Coordinate Geometry--Slope Formula: Example 5 | Math Example--Coordinate Geometry--Slope Formula: Example 5TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a line connecting two points in different quadrants: (-6, -2) in Quadrant III and (6, 5) in Quadrant I. Applying the slope formula, we find that the slope is (5 - (-2)) / (6 - (-6)) = 7 / 12 = 1 / 4. The slope formula is a crucial concept in coordinate geometry, helping us understand the steepness and direction of lines. This example shows how to handle negative coordinates and points in different quadrants when calculating slope. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 5 | Math Example--Coordinate Geometry--Slope Formula: Example 5TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a line connecting two points in different quadrants: (-6, -2) in Quadrant III and (6, 5) in Quadrant I. Applying the slope formula, we find that the slope is (5 - (-2)) / (6 - (-6)) = 7 / 12 = 1 / 4. The slope formula is a crucial concept in coordinate geometry, helping us understand the steepness and direction of lines. This example shows how to handle negative coordinates and points in different quadrants when calculating slope. |
Slope |
|
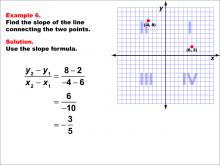
Math Example--Coordinate Geometry--Slope Formula: Example 6 | Math Example--Coordinate Geometry--Slope Formula: Example 6TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a line connecting two points in different quadrants: (-4, 8) in Quadrant II and (6, 2) in Quadrant I. Applying the slope formula, we find that the slope is (8 - 2) / (-4 - 6) = 6 / -10 = -3 / 5. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This example demonstrates how to handle points in different quadrants and interpret a negative slope. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 6 | Math Example--Coordinate Geometry--Slope Formula: Example 6TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a line connecting two points in different quadrants: (-4, 8) in Quadrant II and (6, 2) in Quadrant I. Applying the slope formula, we find that the slope is (8 - 2) / (-4 - 6) = 6 / -10 = -3 / 5. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This example demonstrates how to handle points in different quadrants and interpret a negative slope. |
Slope |
|
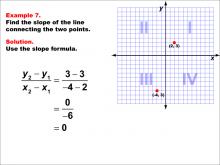
Math Example--Coordinate Geometry--Slope Formula: Example 7 | Math Example--Coordinate Geometry--Slope Formula: Example 7TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a horizontal line connecting two points: (-4, 3) in Quadrant II and (2, 3) in Quadrant I. When we apply the slope formula, we find that the slope is (3 - 3) / (-4 - 2) = 0 / -6 = 0. The slope formula is a key concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is horizontal, resulting in a slope of zero, even when the points are in different quadrants. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 7 | Math Example--Coordinate Geometry--Slope Formula: Example 7TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a horizontal line connecting two points: (-4, 3) in Quadrant II and (2, 3) in Quadrant I. When we apply the slope formula, we find that the slope is (3 - 3) / (-4 - 2) = 0 / -6 = 0. The slope formula is a key concept in coordinate geometry, helping us understand the steepness and direction of lines. This particular example highlights a special case where the line is horizontal, resulting in a slope of zero, even when the points are in different quadrants. |
Slope |
|
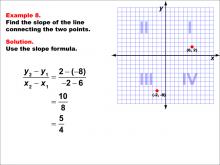
Math Example--Coordinate Geometry--Slope Formula: Example 8 | Math Example--Coordinate Geometry--Slope Formula: Example 8TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a line connecting two points in different quadrants: (-2, -8) in Quadrant III and (6, 2) in Quadrant I. Applying the slope formula, we find that the slope is (2 - (-8)) / (6 - (-2)) = 10 / 8 = 5 / 4. The slope formula is a crucial concept in coordinate geometry, helping us understand the steepness and direction of lines. This example shows how to handle negative coordinates and points in different quadrants when calculating slope. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 8 | Math Example--Coordinate Geometry--Slope Formula: Example 8TopicSlope Formula DescriptionThis example illustrates the calculation of slope for a line connecting two points in different quadrants: (-2, -8) in Quadrant III and (6, 2) in Quadrant I. Applying the slope formula, we find that the slope is (2 - (-8)) / (6 - (-2)) = 10 / 8 = 5 / 4. The slope formula is a crucial concept in coordinate geometry, helping us understand the steepness and direction of lines. This example shows how to handle negative coordinates and points in different quadrants when calculating slope. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 9 | Math Example--Coordinate Geometry--Slope Formula: Example 9TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a line connecting two points: (9, 6) and (2, -8) on a Cartesian plane. Applying the slope formula, we find that the slope is (6 - (-8)) / (9 - 2) = 14 / 7 = 2. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This example shows how to handle points with both positive and negative coordinates when calculating slope. |
Slope |

|
Math Example--Coordinate Geometry--Slope Formula: Example 9 | Math Example--Coordinate Geometry--Slope Formula: Example 9TopicSlope Formula DescriptionThis example demonstrates the calculation of slope for a line connecting two points: (9, 6) and (2, -8) on a Cartesian plane. Applying the slope formula, we find that the slope is (6 - (-8)) / (9 - 2) = 14 / 7 = 2. The slope formula is a fundamental concept in coordinate geometry, helping us understand the steepness and direction of lines. This example shows how to handle points with both positive and negative coordinates when calculating slope. |
Slope |

|
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 1 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 1TopicLinear Functions DescriptionThis example demonstrates how to graph a linear function with a slope of 2 and a y-intercept of 3. The process involves three key steps: first, plotting the y-intercept at (0, 3); second, using the slope to find another point on the line; and finally, connecting these points to form the line. |
Slope-Intercept Form |
|
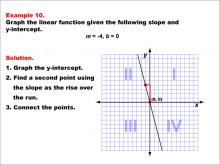
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 10 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 10TopicLinear Functions DescriptionThis example illustrates the process of graphing a linear function with a slope of -4 and a y-intercept of 0. The method involves three main steps: plotting the y-intercept at the origin (0, 0), using the slope to determine a second point on the line, and connecting these points to create the linear graph. |
Slope-Intercept Form |
|
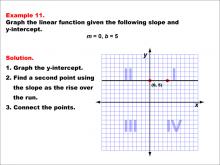
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 11 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 11TopicLinear Functions DescriptionThis example demonstrates the process of graphing a linear function with a slope of 0 and a y-intercept of 5. The procedure involves three key steps: plotting the y-intercept at (0, 5), recognizing that a slope of 0 results in a horizontal line, and drawing the line parallel to the x-axis through the y-intercept. |
Slope-Intercept Form |
|
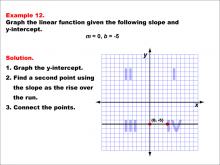
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 12 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 12TopicLinear Functions DescriptionThis example illustrates the process of graphing a linear function with a slope of 0 and a y-intercept of -5. The method involves three main steps: plotting the y-intercept at (0, -5), recognizing that a slope of 0 results in a horizontal line, and drawing the line parallel to the x-axis through the y-intercept. |
Slope-Intercept Form |
|
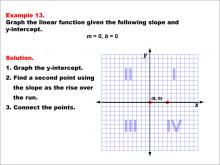
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 13 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 13TopicLinear Functions DescriptionThis example demonstrates how to graph a linear function with a slope of 0 and a y-intercept of 0. The process involves recognizing that this special case results in a horizontal line coinciding with the x-axis. The line passes through the origin (0, 0) and extends infinitely in both directions along the x-axis. |
Slope-Intercept Form |
|
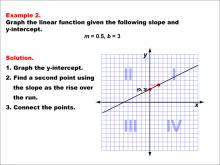
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 2 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 2TopicLinear Functions DescriptionThis example illustrates the process of graphing a linear function with a slope of 0.5 and a y-intercept of 3. The method involves three main steps: plotting the y-intercept at (0, 3), using the slope to determine a second point on the line, and connecting these points to create the linear graph. |
Slope-Intercept Form |
|
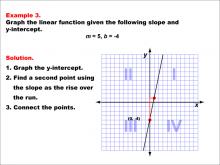
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 3 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 3TopicLinear Functions DescriptionThis example demonstrates the process of graphing a linear function with a slope of 5 and a y-intercept of -4. The procedure involves three key steps: plotting the y-intercept at (0, -4), using the slope to determine a second point on the line, and connecting these points to form the linear graph. |
Slope-Intercept Form |

|
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 4 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 4TopicLinear Functions DescriptionThis example illustrates the process of graphing a linear function with a slope of 0.1 and a y-intercept of -4. The method involves three main steps: plotting the y-intercept at (0, -4), using the slope to determine a second point on the line, and connecting these points to create the linear graph. |
Slope-Intercept Form |
|
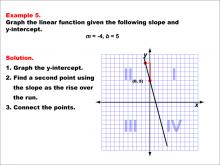
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 5 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 5TopicLinear Functions DescriptionThis example demonstrates how to graph a linear function with a slope of -4 and a y-intercept of 5. The process involves three key steps: first, plotting the y-intercept at (0, 5); second, using the slope to find another point on the line; and finally, connecting these points to form the line. |
Slope-Intercept Form |
|
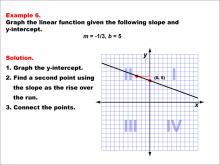
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 6 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 6TopicLinear Functions DescriptionThis example illustrates the process of graphing a linear function with a slope of -1/3 and a y-intercept of 5. The method involves three main steps: plotting the y-intercept at (0, 5), using the slope to determine a second point on the line, and connecting these points to create the linear graph. |
Slope-Intercept Form |

|
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 7 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 7TopicLinear Functions DescriptionThis example demonstrates the process of graphing a linear function with a slope of -3 and a y-intercept of -2. The procedure involves three key steps: plotting the y-intercept at (0, -2), using the slope to determine a second point on the line, and connecting these points to form the linear graph. |
Slope-Intercept Form |
|
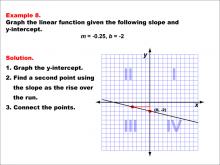
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 8 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 8TopicLinear Functions DescriptionThis example illustrates the process of graphing a linear function with a slope of -0.25 and a y-intercept of -2. The method involves three main steps: plotting the y-intercept at (0, -2), using the slope to determine a second point on the line, and connecting these points to create the linear graph. |
Slope-Intercept Form |

|
Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 9 | Math Example--Linear Function Concepts--Graphs of Linear Functions in Slope-Intercept Form: Example 9TopicLinear Functions DescriptionThis example demonstrates how to graph a linear function with a slope of 0.25 and a y-intercept of 0. The process involves three key steps: first, plotting the y-intercept at the origin (0, 0); second, using the slope to find another point on the line; and finally, connecting these points to form the line. |
Slope-Intercept Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 1 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 1TopicLinear Functions DescriptionThis example demonstrates the process of converting a linear equation from standard form to slope-intercept form. The equation 2x + 4y = 8 is solved step-by-step, isolating y and dividing by its coefficient. The result is y = -1/2 x + 2, clearly showing the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 10 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 10TopicLinear Functions DescriptionThis example illustrates the conversion of the linear equation x + y = 1 from standard form to slope-intercept form. The process involves isolating y, resulting in y = -x + 1. This simple transformation clearly reveals the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 11 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 11TopicLinear Functions DescriptionThis example showcases the transformation of the linear equation x + y = -1 from standard form to slope-intercept form. The process involves isolating y, resulting in y = -x - 1. This step-by-step solution clearly reveals the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 12 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 12TopicLinear Functions DescriptionThis example demonstrates the conversion of the linear equation x - y = 1 from standard form to slope-intercept form. The solution process involves isolating y and changing the sign of both sides, resulting in y = x - 1. This transformation clearly reveals the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 13 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 13TopicLinear Functions DescriptionThis example illustrates the process of converting the linear equation -x + y = 1 from standard form to slope-intercept form. The solution involves rearranging the equation to isolate y, resulting in y = x + 1. This transformation clearly reveals the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 14 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 14TopicLinear Functions DescriptionThis example demonstrates the conversion of the linear equation -x - y = -1 from standard form to slope-intercept form. The process involves manipulating the equation to solve for y, yielding y = -x + 1. This transformation clearly reveals the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 15 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 15TopicLinear Functions DescriptionThis example showcases the transformation of the linear equation -x - y = 1 from standard form to slope-intercept form. The solution process involves isolating y, resulting in y = -x - 1. This step-by-step conversion clearly reveals the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 16 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 16TopicLinear Functions DescriptionThis example demonstrates the conversion of the linear equation -x + y = -1 from standard form to slope-intercept form. The process involves isolating y, resulting in y = x - 1. This transformation clearly reveals the slope and y-intercept of the line. Linear functions are fundamental mathematical concepts that describe relationships between two variables. The examples in this collection, such as showing step-by-step transformations from standard form to slope-intercept form, help in understanding how each part of the equation affects the graph and the relationship itself. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 17 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 17TopicLinear Functions DescriptionThis example illustrates the conversion of the linear equation x - y = -1 from standard form to slope-intercept form. The solution involves isolating y, resulting in y = x + 1. This process clearly reveals the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 18 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 18TopicLinear Functions DescriptionThis example demonstrates the conversion of the linear equation -x - y = 1 from standard form to slope-intercept form. The process involves rearranging the equation to isolate y, resulting in y = -x - 1. This transformation clearly reveals the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 19 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 19TopicLinear Functions DescriptionThis example illustrates the conversion of the linear equation 12x + 28y = 0 from standard form to slope-intercept form. The solution involves isolating y and dividing by its coefficient, resulting in y = -3/7 x. This process clearly reveals the slope and y-intercept of the line. |
Standard Form |

|
Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 2 | Math Example--Linear Function Concepts--Linear Equations in Standard Form: Example 2TopicLinear Functions DescriptionThis example illustrates the conversion of the linear equation 3x + 6y = -18 from standard form to slope-intercept form. The solution involves isolating y and dividing by its coefficient, resulting in y = -1/2 x - 3. This process clearly reveals the slope and y-intercept of the line. |
Standard Form |