Illustrative Math Alignment: Grade 6 Unit 9

Putting it All Together

Lesson 4: How Do We Choose?

Use the following Media4Math resources with this Illustrative Math lesson.

Title	Body	Curriculum Topics
Math Example: Fraction Operations--Subtracting Fractions Example 9	Subtracting Fractions Example 9 Topic Fraction Operations	Add and Subtract Fractions
Math Example: Fraction Operations--Subtracting Fractions Example 10	Subtracting Fractions Example 10 Topic Fraction Operations	Add and Subtract Fractions
Math Example: Fraction Operations--Subtracting Fractions Example 11	Subtracting Fractions Example 11 Topic Fraction Operations	Add and Subtract Fractions
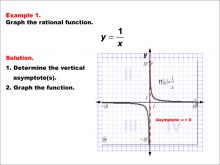
Math Example--Rational Concepts--Graphs of Rational Functions: Example 1	Math Example--Rational Concepts--Graphs of Rational Functions: Example 1 Topic Rational Functions Description This example demonstrates the graph of the rational function y = 1 / x. The graph is a hyperbola with vertical and horizontal asymptotes, with the vertical asymptote occurring at x = 0. Students are tasked with graphing the function and determining its vertical asymptote.	Rational Functions and Equations
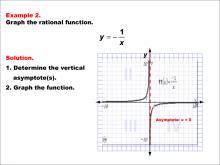
Math Example--Rational Concepts--Graphs of Rational Functions: Example 2	Math Example--Rational Concepts--Graphs of Rational Functions: Example 2 Topic Rational Functions Description This example illustrates the graph of the rational function y = -1 / x. The graph is a hyperbola with vertical and horizontal asymptotes, with the vertical asymptote at x = 0. Students are asked to graph the function and identify its vertical asymptote.	Rational Functions and Equations
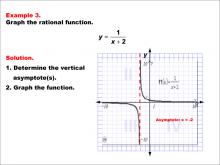
Math Example--Rational Concepts--Graphs of Rational Functions: Example 3	Math Example--Rational Concepts--Graphs of Rational Functions: Example 3 Topic Rational Functions Description This example showcases the graph of the rational function y = 1 / (x + 2). The graph is a hyperbola with a vertical asymptote at x = -2. Students are tasked with graphing the function and determining its vertical asymptote.	Rational Functions and Equations
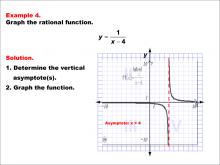
Math Example--Rational Concepts--Graphs of Rational Functions: Example 4	Math Example--Rational Concepts--Graphs of Rational Functions: Example 4 Topic Rational Functions Description This example presents the graph of the rational function y = 1 / (x - 4). The graph is a hyperbola with a vertical asymptote at x = 4. Students are asked to graph the function and identify its vertical asymptote.	Rational Functions and Equations
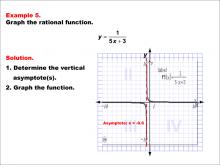
Math Example--Rational Concepts--Graphs of Rational Functions: Example 5	Math Example--Rational Concepts--Graphs of Rational Functions: Example 5 Topic Rational Functions Description This example illustrates the graph of the rational function y = 1 / (5x + 3). The graph features a vertical asymptote at x = -0.6, indicated by a dashed red line. Students are tasked with graphing the function and determining its vertical asymptote.	Rational Functions and Equations
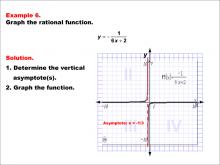
Math Example--Rational Concepts--Graphs of Rational Functions: Example 6	Math Example--Rational Concepts--Graphs of Rational Functions: Example 6 Topic Rational Functions Description This example demonstrates the graph of the rational function y = -1 / (6x + 2). The graph features a vertical asymptote at x = -1/3, shown with a dashed red line on the grid. Students are asked to graph the function and identify its vertical asymptote.	Rational Functions and Equations
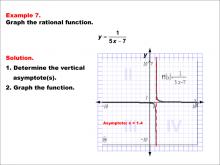
Math Example--Rational Concepts--Graphs of Rational Functions: Example 7	Math Example--Rational Concepts--Graphs of Rational Functions: Example 7 Topic Rational Functions Description This example showcases the graph of the rational function y = 1 / (5x - 7). The graph features a vertical asymptote at x = 1.4, marked by a dashed red line on the grid. Students are tasked with graphing the function and determining its vertical asymptote.	Rational Functions and Equations
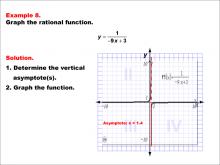
Math Example--Rational Concepts--Graphs of Rational Functions: Example 8	Math Example--Rational Concepts--Graphs of Rational Functions: Example 8 Topic Rational Functions Description This example presents the graph of the rational function y = 1 / (-9x + 3). The graph is plotted on a grid with a vertical asymptote at x = 1/3, marked by a dashed red line. Students are asked to graph the function and identify its vertical asymptote.	Rational Functions and Equations
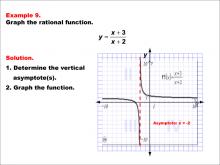
Math Example--Rational Concepts--Graphs of Rational Functions: Example 9	Math Example--Rational Concepts--Graphs of Rational Functions: Example 9 Topic Rational Functions Description This example illustrates the graph of the rational function y = (x + 3) / (x + 2). The graph features a vertical asymptote at x = -2 and shows two branches, one in the second quadrant and one in the fourth quadrant. Students are tasked with graphing the function and determining its vertical asymptote.	Rational Functions and Equations
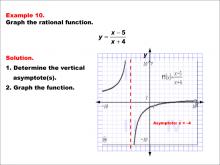
Math Example--Rational Concepts--Graphs of Rational Functions: Example 10	Math Example--Rational Concepts--Graphs of Rational Functions: Example 10 Topic Rational Functions Description This example showcases the graph of the rational function y = (x - 5) / (x + 4). The graph features a vertical asymptote at x = -4 and displays two branches, one in the first quadrant and one in the third quadrant. Students are asked to graph the function and identify its vertical asymptote.	Rational Functions and Equations
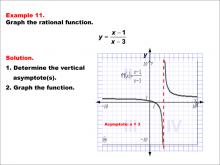
Math Example--Rational Concepts--Graphs of Rational Functions: Example 11	Math Example--Rational Concepts--Graphs of Rational Functions: Example 11 Topic Rational Functions Description This example showcases the graph of the rational function y = (x - 1) / (x - 3). The graph features a vertical asymptote at x = 3 and displays two distinct branches, one in the first quadrant and another in the third quadrant. Students are tasked with graphing the function and identifying its vertical asymptote.	Rational Functions and Equations
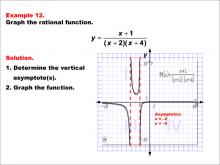
Math Example--Rational Concepts--Graphs of Rational Functions: Example 12	Math Example--Rational Concepts--Graphs of Rational Functions: Example 12 Topic Rational Functions Description This example illustrates the graph of the rational function y = (x + 1) / ((x + 2)(x + 4)). The graph features two vertical asymptotes at x = -2 and x = -4, and displays three distinct branches, with one in each of the first, second, and third quadrants. Students are asked to graph the function and identify its vertical asymptotes.	Rational Functions and Equations
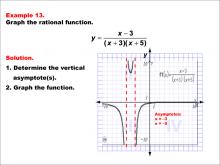
Math Example--Rational Concepts--Graphs of Rational Functions: Example 13	Math Example--Rational Concepts--Graphs of Rational Functions: Example 13 Topic Rational Functions Description This example presents the graph of the rational function y = (x - 3) / ((x + 3)(x + 5)). The graph features vertical asymptotes at x = -3 and x = -5, illustrating how the function behaves near these lines. Students are tasked with graphing the function and determining its vertical asymptotes.	Rational Functions and Equations
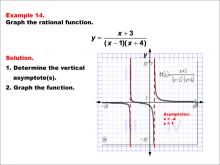
Math Example--Rational Concepts--Graphs of Rational Functions: Example 14	Math Example--Rational Concepts--Graphs of Rational Functions: Example 14 Topic Rational Functions Description This example illustrates the graph of the rational function y = (x + 3) / ((x - 1)(x + 4)). The graph features vertical asymptotes at x = -4 and x = 1, displaying how the function behaves near these lines. Students are asked to graph the function and identify its vertical asymptotes.	Rational Functions and Equations
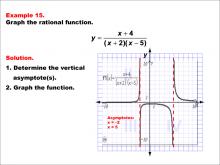
Math Example--Rational Concepts--Graphs of Rational Functions: Example 15	Math Example--Rational Concepts--Graphs of Rational Functions: Example 15 Topic Rational Functions Description This example showcases the graph of the rational function y = (x + 4) / ((x + 2)(x - 5)). The graph features vertical asymptotes at x = -2 and x = 5, demonstrating the behavior of the function near these asymptotes. Students are tasked with graphing the function and determining its vertical asymptotes.	Rational Functions and Equations
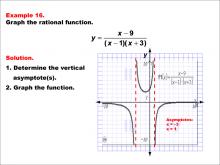
Math Example--Rational Concepts--Graphs of Rational Functions: Example 16	Math Example--Rational Concepts--Graphs of Rational Functions: Example 16 Topic Rational Functions Description This example illustrates the graph of the rational function y = (x - 9) / ((x - 1)(x + 3)). The graph features vertical asymptotes at x = -3 and x = 1, showing the function's behavior around these asymptotes. Students are asked to graph the function and identify its vertical asymptotes.	Rational Functions and Equations
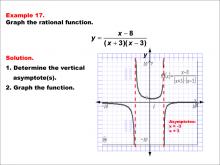
Math Example--Rational Concepts--Graphs of Rational Functions: Example 17	Math Example--Rational Concepts--Graphs of Rational Functions: Example 17 Topic Rational Functions Description This example presents the graph of the rational function y = (x - 1) / ((x - 2)(x - 3)). The graph features vertical asymptotes at x = 2 and x = 3, marked with dashed red lines. Students are tasked with graphing the function and determining its vertical asymptotes.	Rational Functions and Equations
Math Example--Rational Concepts--Graphs of Rational Functions: Example 18	Math Example--Rational Concepts--Graphs of Rational Functions: Example 18 Topic Rational Functions Description This example illustrates the graph of the rational function y = (x - 8) / ((x + 3)(x - 3)). The graph features vertical asymptotes at x = -3 and x = 3, highlighted by dashed red lines. Students are asked to graph the function and identify its vertical asymptotes.	Rational Functions and Equations
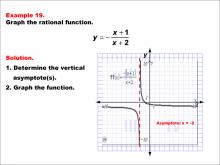
Math Example--Rational Concepts--Graphs of Rational Functions: Example 19	Math Example--Rational Concepts--Graphs of Rational Functions: Example 19 Topic Rational Functions Description This example presents the graph of the rational function y = -(x + 1) / (x + 2). The graph features a single vertical asymptote at x = -2, indicated by a dashed red line. Students are tasked with graphing the function and determining its vertical asymptote.	Rational Functions and Equations
Math Example--Rational Concepts--Graphs of Rational Functions: Example 20	Math Example--Rational Concepts--Graphs of Rational Functions: Example 20 Topic Rational Functions Description This example showcases the graph of the rational function y = -(x - 3) / (x + 4). The graph features a vertical asymptote at x = -4, but it is incorrectly labeled as x = -2. Students are asked to graph the function and identify its vertical asymptote.	Rational Functions and Equations
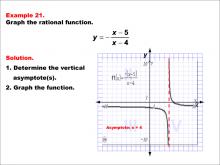
Math Example--Rational Concepts--Graphs of Rational Functions: Example 21	Math Example--Rational Concepts--Graphs of Rational Functions: Example 21 Topic Rational Functions Description This example illustrates the graph of the rational function y = -(x - 5) / (x - 4). The graph features a vertical asymptote at x = 4, which is correctly labeled. Students are tasked with graphing the function and determining its vertical asymptote.	Rational Functions and Equations
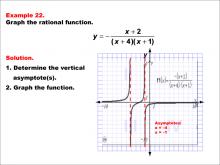
Math Example--Rational Concepts--Graphs of Rational Functions: Example 22	Math Example--Rational Concepts--Graphs of Rational Functions: Example 22 Topic Rational Functions Description This example presents the graph of the rational function y = -(x + 2) / ((x + 4)(x + 1)). The graph features vertical asymptotes at x = -4 and x = -1. Students are asked to graph the function and identify its vertical asymptotes.	Rational Functions and Equations
Math Example--Rational Concepts--Graphs of Rational Functions: Example 23	Math Example--Rational Concepts--Graphs of Rational Functions: Example 23 Topic Rational Functions Description This example illustrates the graph of the rational function y = -(x - 4) / ((x + 5)(x + 2)). The graph features vertical asymptotes at x = -5 and x = -2. Students are tasked with graphing the function and determining its vertical asymptotes.	Rational Functions and Equations
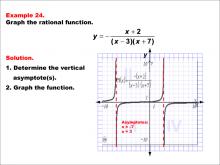
Math Example--Rational Concepts--Graphs of Rational Functions: Example 24	Math Example--Rational Concepts--Graphs of Rational Functions: Example 24 Topic Rational Functions Description This example showcases the graph of the rational function y = -(x + 2) / ((x - 3)(x + 7)). The graph features vertical asymptotes at x = -7 and x = 3. Students are asked to graph the function and identify its vertical asymptotes.	Rational Functions and Equations
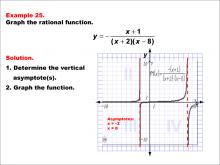
Math Example--Rational Concepts--Graphs of Rational Functions: Example 25	Math Example--Rational Concepts--Graphs of Rational Functions: Example 25 Topic Rational Functions Description This example illustrates the graph of the rational function y = -(x + 1) / ((x + 2)(x - 8)). The graph features vertical asymptotes at x = -2 and x = 8. Students are tasked with graphing the function and determining its vertical asymptotes.	Rational Functions and Equations
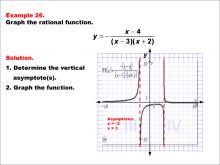
Math Example--Rational Concepts--Graphs of Rational Functions: Example 26	Math Example--Rational Concepts--Graphs of Rational Functions: Example 26 Topic Rational Functions Description This example showcases the graph of the rational function y = -(x - 4) / ((x - 3)(x + 2)). The graph features vertical asymptotes at x = -2 and x = 3. Students are asked to graph the function and identify its vertical asymptotes.	Rational Functions and Equations
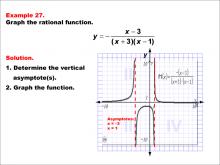
Math Example--Rational Concepts--Graphs of Rational Functions: Example 27	Math Example--Rational Concepts--Graphs of Rational Functions: Example 27 Topic Rational Functions Description This example presents the graph of the rational function y = -(x - 3) / ((x + 3)(x - 1)). The graph features vertical asymptotes at x = -3 and x = 1. Students are tasked with graphing the function and determining its vertical asymptotes.	Rational Functions and Equations
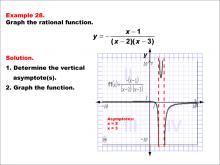
Math Example--Rational Concepts--Graphs of Rational Functions: Example 28	Math Example--Rational Concepts--Graphs of Rational Functions: Example 28 Topic Rational Functions Description This example illustrates the graph of the rational function y = -(x - 1) / ((x - 2)(x - 3)). The graph features vertical asymptotes at x = 2 and x = 3, marked with dashed red lines on the coordinate plane. Students are asked to graph the function and identify its vertical asymptotes.	Rational Functions and Equations
Math Example: Fraction Operations--Multiplying Fractions: Example 1	Multiplying Fractions: Example 1 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 2	Multiplying Fractions: Example 2 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 3	Multiplying Fractions: Example 3 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 4	Multiplying Fractions: Example 4 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 5	Multiplying Fractions: Example 5 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 6	Multiplying Fractions: Example 6 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 7	Multiplying Fractions: Example 7 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 8	Multiplying Fractions: Example 8 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 9	Multiplying Fractions: Example 9 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 10	Multiplying Fractions: Example 10 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 11	Multiplying Fractions: Example 11 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 12	Multiplying Fractions: Example 12 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 13	Multiplying Fractions: Example 13 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 14	Multiplying Fractions: Example 14 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Multiplying Fractions: Example 15	Multiplying Fractions: Example 15 Topic Fraction Operations	Fractions and Mixed Numbers
Math Example: Fraction Operations--Adding Fractions: Example 1	Adding Fractions: Example 1 Topic Fraction Operations Description This example demonstrates the concept of adding fractions with like denominators. The image likely shows two fractions that need to be added together. Since there is a common denominator, the numerators are then added, and the resulting fraction does not need to be simplified. This process involves skills such as recognizing common denominators, adding numerators, and identifying a fraction in simplest form.. For a complete collection of math examples related to Adding Fractions click on this link: Math Examples: Adding Fractions Collection.	Add and Subtract Fractions
Math Example: Fraction Operations--Adding Fractions: Example 2	Adding Fractions: Example 2 Topic Fraction Operations Description This example illustrates the addition of fractions with common denominators. The key concept is to identify the common denominator. The numerators are summed, and the final fraction is simplified. Skills required include recognizing common denominators, adding numerators, and simplifying the result. For a complete collection of math examples related to Adding Fractions click on this link: Math Examples: Adding Fractions Collection.	Add and Subtract Fractions
Math Example: Fraction Operations--Adding Fractions: Example 3	Adding Fractions: Example 3 Topic Fraction Operations Description This example focuses on adding fractions that have different denominators. The image depicts the fractions and the steps needed to add them. The process involves finding a common denominator, converting the fractions to have this common denominator, adding the numerators, and simplifying the resulting fraction. In this example, one denominator is a multiple of the other denominator. Skills involved include finding the least common multiple, converting fractions, adding numerators, and simplifying the final fraction.	Add and Subtract Fractions
Math Example: Fraction Operations--Adding Fractions: Example 4	Adding Fractions: Example 4 Topic Fraction Operations Description This example demonstrates the addition of fractions with unlike denominators. The image shows the fractions and the method to add them. One denominator is a multiple of the other. The key steps include identifying a common denominator, rewriting the fractions with this denominator, summing the numerators, and simplifying the resulting fraction. Skills required include determining the least common multiple, adjusting fractions, performing addition, and simplifying the fraction.	Add and Subtract Fractions