Illustrative Math Alignment: Grade 7 Unit 7

Angles, Triangles, and Prisms

Lesson 2: Adjacent Angles

Use the following Media4Math resources with this Illustrative Math lesson.

Thumbnail Image	Title	Body	Curriculum Nodes
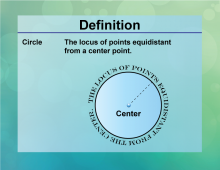
	Definition--Circle Concepts--Circle	Circle Topic Circles Definition A circle is a set of all points in a plane equidistant from a given point, the center. Description The circle is one of the most fundamental shapes in geometry, characterized by its symmetry and uniformity. It is used extensively in various fields, including engineering, design, and astronomy, where its properties are applied to create wheels, gears, and orbits. Mathematically, a circle is defined by its center and radius, and its equation in a plane is (x − h)2 + (y − k)2 = r2	Definition of a Circle
	Definition--Circle Concepts--Circle Inscribed in a Square	Circle Inscribed in a Square Topic Circles Definition A circle inscribed in a square touches all four sides of the square at exactly one point each.	Definition of a Circle
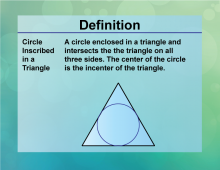
	Definition--Circle Concepts--Circle Inscribed in a Triangle	Circle Inscribed in a Triangle Topic Circles Definition An inscribed circle in a triangle is tangent to each of the triangle's sides.	Definition of a Circle
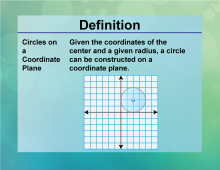
	Definition--Circle Concepts--Circles on a Coordinate Plane	Circles on a Coordinate Plane Topic Circles Definition Circles on a coordinate plane are defined by their center coordinates and radius. Description Understanding circles on a coordinate plane is essential for analyzing geometric shapes in a mathematical context. This concept is widely used in computer graphics, navigation systems, and physics simulations, where precise positioning and movement of circular objects are required. The standard equation of a circle in the coordinate plane is (x − h)2 + (y − k)2 = r2	Definition of a Circle
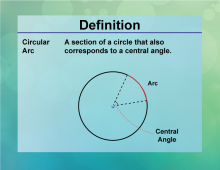
	Definition--Circle Concepts--Circular Arc	Circular Arc Topic Circles Definition A circular arc is a portion of the circumference of a circle. Description Circular arcs are segments of a circle's circumference, used extensively in design, architecture, and engineering to create curved structures and paths. The length of an arc is determined by the central angle and the circle's radius, calculated using the formula L = rθ	Definition of a Circle
	Definition--Circle Concepts--Circular Composite Figures	Circular Composite Figures Topic Circles Definition Circular composite figures are shapes that include circles or parts of circles combined with other geometric figures.	Definition of a Circle
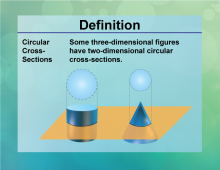
	Definition--Circle Concepts--Circular Cross-Sections	Circular Cross-Sections Topic Circles Definition Circular cross-sections are the intersections of a plane with a solid that result in a circle.	Definition of a Circle
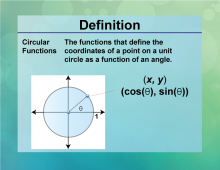
	Definition--Circle Concepts--Circular Functions	Circular Functions Topic Circles Definition Circular functions are trigonometric functions that relate angles to ratios of sides in a right triangle.	Definition of a Circle
	Definition--Circle Concepts--Circular Models	Circular Models Topic Circles Definition Circular models are representations of circular phenomena using mathematical equations and diagrams.	Definition of a Circle
	Definition--Circle Concepts--Circular Numerical Models	Circular Numerical Models Topic Circles Definition Circular numerical models use numerical methods to analyze and simulate circular phenomena.	Definition of a Circle
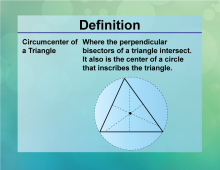
	Definition--Circle Concepts--Circumcenter of Triangle	Circumcenter of Triangle Topic Circles Definition The circumcenter of a triangle is the point where the perpendicular bisectors of the sides intersect, equidistant from the vertices.	Definition of a Circle
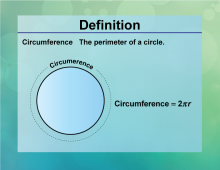
	Definition--Circle Concepts--Circumference	Circumference Topic Circles Definition The circumference of a circle is the distance around the circle, calculated as C = 2πr. Description The circumference is a fundamental concept in geometry, representing the perimeter of a circle. It is widely used in fields such as engineering, design, and manufacturing, where precise measurements of circular objects are required. The formula C = 2πr	Definition of a Circle
	Definition--Circle Concepts--Circumscribed Angle	Circumscribed Angle Topic Circles Definition A circumscribed angle is an angle formed outside a circle by two intersecting tangents.	Definition of a Circle
	Definition--Circle Concepts--Concentric Circles	Concentric Circles Topic Circles Definition Concentric circles are circles that share the same center but have different radii.	Definition of a Circle
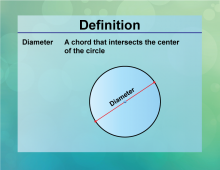
	Definition--Circle Concepts--Diameter	Diameter Topic Circles Definition The diameter of a circle is a line segment that passes through the center and has its endpoints on the circle, calculated as D = 2πr. Description The diameter is a fundamental concept in geometry, representing the longest distance across a circle. It is widely used in fields such as engineering, design, and manufacturing, where precise measurements of circular objects are required. The formula D = 2πr	Definition of a Circle
	Definition--Circle Concepts--Equation of a Circles	Equation of a Circle Topic Circles Definition The equation of a circle in a plane is (x − h)2 + (y − k)2 = r2, where (h , k) is the center and r is the radius.	Definition of a Circle
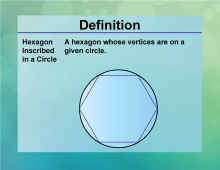
	Definition--Circle Concepts--Hexagon Inscribed in a Circle	Hexagon Inscribed in a Circle Topic Circles Definition A hexagon inscribed in a circle is a six-sided polygon where each vertex touches the circle.	Definition of a Circle
	Definition--Circle Concepts--Incenter of a Triangle	Incenter of a Triangle Topic Circles Definition The incenter of a triangle is the point where the angle bisectors intersect, equidistant from the sides.	Definition of a Circle
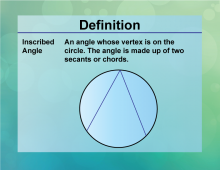
	Definition--Circle Concepts--Inscribed Angle	Inscribed Angle Topic Circles Definition An inscribed angle is an angle formed by two chords in a circle that share an endpoint.	Definition of a Circle
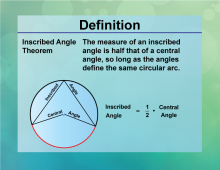
	Definition--Circle Concepts--Inscribed Angle Theorem	Inscribed Angle Theorem Topic Circles Definition The inscribed angle theorem states that an inscribed angle is half the measure of the central angle that subtends the same arc.	Definition of a Circle
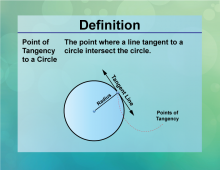
	Definition--Circle Concepts--Point of Tangency to a Circle	Point of Tangency to a Circle Topic Circles Definition The point of tangency is the point where a tangent line touches the circle.	Definition of a Circle
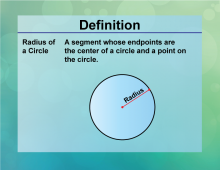
	Definition--Circle Concepts--Radius of a Circle	Radius of a Circle Topic Circles Definition The radius of a circle is a line segment from the center to any point on the circle.	Definition of a Circle
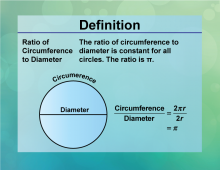
	Definition--Circle Concepts--Ratio of Circumference to Diameter	Ratio of Circumference to Diameter Topic Circles Definition The ratio of the circumference to the diameter of a circle is the constant π.	Definition of a Circle
	Definition--Circle Concepts--Secant to a Circle	Secant to a Circle Topic Circles Definition A secant is a line that intersects a circle at two points. Description Secants are significant in geometry, representing lines that intersect a circle at two distinct points. These lines are used in various applications, such as in design and architecture, where precise measurements of angles and distances are essential. In mathematics, secants are explored in the context of circle theorems, providing insights into the properties of lines and circles. In education, understanding secants helps students develop geometric reasoning and problem-solving skills, which are essential for advanced studies in geometry and trigonometry.	Definition of a Circle
	Definition--Circle Concepts--Sector	Sector Topic Circles Definition A sector is a portion of a circle enclosed by two radii and the arc between them. Description Sectors are fundamental in the study of circles, representing a portion of the circle's area. These shapes are used in various applications, such as in design and architecture, where precise measurements of angles and areas are essential. The area of a sector is calculated using the formula A = 1/2r2θ	Definition of a Circle
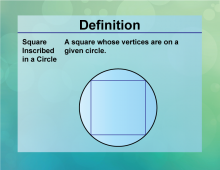
	Definition--Circle Concepts--Square Inscribed in a Circle	Square Inscribed in a Circle Topic Circles Definition A square inscribed in a circle has its vertices touching the circle.	Definition of a Circle
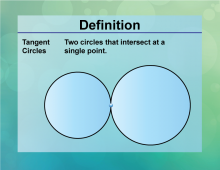
	Definition--Circle Concepts--Tangent Circles	Tangent Circles Topic Circles Definition Tangent circles are two or more circles that intersect at exactly one point.	Definition of a Circle
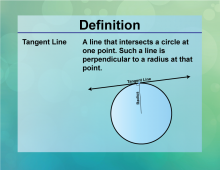
	Definition--Circle Concepts--Tangent Line	Tangent Line Topic Circles Definition A tangent line is a straight line that touches a circle at exactly one point.	Definition of a Circle
	Definition--Circle Concepts--Triangle Inscribed in a Circle	Triangle Inscribed in a Circle Topic Circles Definition A triangle inscribed in a circle has its vertices on the circle.	Definition of a Circle
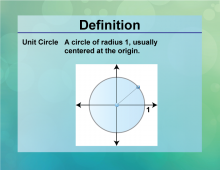
	Definition--Circle Concepts--Unit Circle	Unit Circle Topic Circles Definition The unit circle is a circle with a radius of one, centered at the origin of the coordinate plane.	Definition of a Circle
	Definition--Geometry Basics--Antiprism	Antiprism Topic Geometry Basics Definition A polyhedron with two parallel faces and other faces that are triangular. Description An antiprism is a type of polyhedron that has two parallel faces connected by an alternating band of triangles. This geometric shape is significant in advanced geometry and has applications in fields such as crystallography and molecular chemistry. Understanding antiprisms helps in comprehending the properties of polyhedra and their role in three-dimensional space. For example, the volume of an antiprism can be calculated using specific geometric formulas, making it a useful concept in various scientific and mathematical applications.	3-Dimensional Figures
	Definition--Geometry Basics--Prism	Prism Topic Geometry Basics Definition A prism is a solid shape with two parallel, congruent bases connected by rectangular or parallelogram faces. Description Prisms are significant in the study of three-dimensional shapes. They have a uniform cross-section, allowing for easy calculations of volume and surface area. The volume can be calculated with the formula V = B⋅h where B is the area of the base and h is the height. Prisms are commonly encountered in real-world applications such as packaging and structural designs, making them an essential concept in geometry.	3-Dimensional Figures
	Formulas--Surface Area of a Cube	Formulas--Surface Area of a Cube The formula for the Surface Area of a Cube. This is part of a collection of math formulas. To see the complete collection of formulas, click on this link. Note: The download is a JPG file. Related Resources To see resources related to this topic click on the Related Resources tab above.	Surface Area
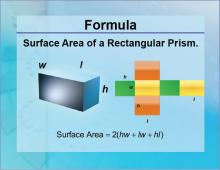
	Formulas--Surface Area of a Rectangular Prism	Formulas--Surface Area of a Rectangular Prism The formula for the Surface Area of a Rectangular Prism. This is part of a collection of math formulas. To see the complete collection of formulas, click on this link. Note: The download is a JPG file. Related Resources To see resources related to this topic click on the Related Resources tab above.	Surface Area
	Geometry Applications Teachers Guide: 3D Geometry	Geometry Applications Teachers Guide: 3D Geometry This is the Teacher's Guide that accompanies Geometry Applications: 3D Geometry. This is part of a collection of teacher's guides. To see the complete collection of teacher's guides, click on this link. Note: The download is a PDF file. Related Resources To see resources related to this topic click on the Related Resources tab above.	Applications of 3D Geometry
	Google Earth Voyager Story: Architectural Prisms, Part 1	Google Earth Voyager Story: Architectural Prisms, Part 1 Topic Geometric Models	Triangular Prisms
	Google Earth Voyager Story: Architectural Prisms, Part 2	Google Earth Voyager Story: Architectural Prisms, Part 2 Topic Geometric Models	Rectangular Prisms
	Google Earth Voyager Story: Architectural Prisms, Part 3	Google Earth Voyager Story: Architectural Prisms, Part 3 In this Google Earth Voyager story visit architectural landmarks in New York City to learn about the geometry of prisms. In this lesson, visit the Freedom Tower in Lower Manhattan and learn about antiprisms. Then build a scale model of the Freedom Tower. This is part of a collection of Google Earth Voyager Stories that use Google's mapping technology to explore relevant math stories. To see the complete collection of the Google Earth Voyager Stories, click on this link. Note: The download is the teacher's guide. Viewing Media4Math Stories on Google Earth These Google Earth Voyager Stories are also available on Google Earth. Best viewed in Chrome, click on the links below to view these dynamic stories using Google's technology:	3-Dimensional Figures
	Google Earth Voyager Story: Polygon Architecture, Part 1	Google Earth Voyager Story: Polygon Architecture, Part 1 Topic Geometric Models	Applications of Polygons
	Google Earth Voyager Story: Polygon Architecture, Part 2	Google Earth Voyager Story: Polygon Architecture, Part 2 Topic Geometric Models	Applications of Polygons
	Instructional Resource--Geometric Construction--Constructing an Egg Shape	Instructional Resource \| Geometric Construction \| An Egg Shape In this instructional resource, we show the steps in constructing an egg shape. In the process, students learn about using common points of tangency to create smooth curves from two different shapes. This activity can be done with pencil, compass, ruler, and grid paper. —PRESS PREVIEW TO VIEW THE ACTIVITY— To see the complete collection of Instructional Resource, click on this link.	Geometric Constructions with Circles
	INSTRUCTIONAL RESOURCE: Algebra Application: Interior Angles of a Polygon	In this Slide Show, apply concepts of linear functions to the context of the interior angles of a polygon. Note: The download is a PPT file.	Definition of a Polygon
	INSTRUCTIONAL RESOURCE: Math Examples 1	INSTRUCTIONAL RESOURCE: Math Examples--Circular Area and Circumference This set of tutorials provides 23 examples of solving for the area and circumferences of circles and sections of circles. This is part of a collection of math examples for a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Area and Circumference
	INSTRUCTIONAL RESOURCE: Math Examples 37	INSTRUCTIONAL RESOURCE: Math Examples--Polygon Classification This set of tutorials provides 36 examples of how to classify polygons based on side and angle characteristics. This is part of a collection of math examples for a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Definition of a Polygon
	INSTRUCTIONAL RESOURCE: Math Examples 42	INSTRUCTIONAL RESOURCE: Math Examples--Quadrilateral Area and Perimeter This set of tutorials provides an overview of the 24 worked-out examples that show how to calculate the area and perimeter of different quadrilaterals. This is part of a collection of math examples for a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Area and Perimeter of Quadrilaterals
	INSTRUCTIONAL RESOURCE: Math Examples 43	INSTRUCTIONAL RESOURCE: Math Examples--Quadrilateral Classification This set of tutorials provides 34 examples of the types of quadrilaterals and how to classify quadrilaterals based on the sides and angles. This is part of a collection of math examples for a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Definition of a Quadrilateral
	INSTRUCTIONAL RESOURCE: Math Examples 55	INSTRUCTIONAL RESOURCE: Math Examples--Surface Area This set of tutorials provides an overview of the 24 worked-out examples that show how to calculate the surface area of different three-dimensional figures. This is part of a collection of math examples for a variety of math topics. To see the complete collection of these resources, click on this link. Note: The download is a PPT file. Library of Instructional Resources To see the complete library of Instructional Resources , click on this link.	Surface Area
	Instructional Resource: TI-Nspire Activity: Constructing and Measuring the Area of a Polygon	In this TI-Nspire Activity, use the Geometry Tools to construct a regular polygon and measure its area using the apothem-perimeter formula. Note: The Preview is a Google Slide Show and the download is a PPT. Subscribers to Media4Math can download resources. To see the complete collection of TI graphing calculator resources, click on this Link.	Geometric Constructions with Polygons
	Interactive Crossword Puzzle--Circles	Interactive Crossword Puzzle--Circles This interactive crossword puzzle tests knowledge of key terms on the topic of circles. This is part of a collection of math games and interactives. To see the complete collection of the games, click on this link. Note: The download is the teacher's guide. Related Resources To see additional resources on this topic, click on the Related Resources tab.	Definition of a Circle
$Math Clip Art--Adjacent Angles--Labeled$	Math Clip Art--Angle Illustrations--Adjacent Angles--Labeled	Math Clip Art--Angle Illustrations--Adjacent Angles--Labeled This is part of a collection of clip art images showing different types of angles. Each type of angle measure includes a labeled and unlabeled version.	Angles and Applications of Angles and Planes