
Illustrative Math Alignment: Grade 8 Unit 2
Dilations, Similarity, and Introducing Slope
Lesson 13: The Shadow Knows
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|
|
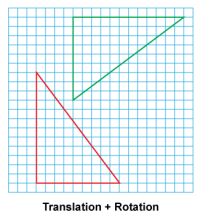
Math Clip Art--Geometry Concepts--Similarity with Transformations--07 | Math Clip Art--Similarity with Transformations--07TopicGeometry Concepts DescriptionThis image illustrates a triangle undergoing a combination of translation and rotation. The translation moves the triangle to a new position, and the rotation changes its orientation. This composite transformation demonstrates how multiple transformations can be applied sequentially. The resulting triangle is congruent to the original but different in both position and orientation. |
Proportions |
|
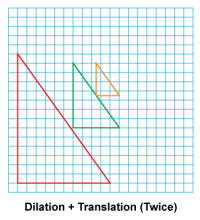
Math Clip Art--Geometry Concepts--Similarity with Transformations--08 | Math Clip Art--Similarity with Transformations--08TopicGeometry Concepts DescriptionThis image shows a triangle undergoing a combination of dilation and translation performed twice. The dilation changes the size of the triangle while maintaining its shape, and the translations move it to new positions. This complex transformation demonstrates how multiple transformations can be applied repeatedly. The resulting triangles are similar to the original but differ in size and position. |
Proportions |
|
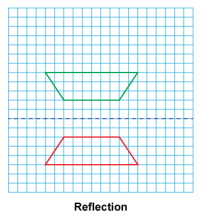
Math Clip Art--Geometry Concepts--Similarity with Transformations--09 | Math Clip Art--Similarity with Transformations--09TopicGeometry Concepts DescriptionThis image depicts a trapezoid reflected across a horizontal line. Reflection creates a mirror image of the original figure across a line of reflection. This transformation demonstrates how a figure can be flipped over a line to create its mirror image. The resulting trapezoid is congruent to the original but inverted vertically. Teacher's Script: "Observe how the trapezoid is reflected across the horizontal line. What properties of the trapezoid remain unchanged? How does the orientation of the trapezoid change after reflection? Can you identify the line of reflection?" |
Proportions |
|
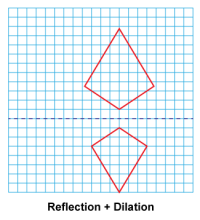
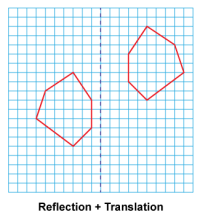
Math Clip Art--Geometry Concepts--Similarity with Transformations--10 | Math Clip Art--Similarity with Transformations--10TopicGeometry Concepts DescriptionThis image shows a kite reflected across a horizontal line, followed by a dilation. Reflection creates a mirror image of the original figure across a line of reflection. Dilation changes the size of the original figure but keeps it proportional. This transformation demonstrates how a figure can be flipped over a line to create its mirror image. The resulting kite is similar to the original but inverted vertically. |
Proportions |
|
Math Clip Art--Geometry Concepts--Similarity with Transformations--11 | Math Clip Art--Similarity with Transformations--11TopicGeometry Concepts DescriptionThis image illustrates an irregular hexagon reflected across a vertical line. Reflection creates a mirror image of the original figure across a line of reflection. This transformation demonstrates how a complex figure can be flipped over a vertical line to create its mirror image. The resulting hexagon is congruent to the original but reversed horizontally. |
Proportions |
|
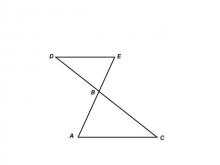
Math Clip Art--Triangles--Two Triangles Share Vertical Angles Similar--Labeled | Math Clip Art--Triangles--Two Triangles Share Vertical Angles Similar--Labeled
This is from a collection of triangular shapes. They come labeled and unlabeled. |
Applications of Triangles |

|
Math Clip Art: Parallel Lines Cut by a Transversal 1 | Math Clip Art: Parallel Lines Cut by a Transversal 1TopicGeometry DescriptionThis math clip art image is part of a series that illustrates the properties of parallel lines cut by a transversal. In this particular image, we see two parallel lines without a transversal, serving as an introduction to the concept. The image is designed to help students visualize and understand the fundamental idea of parallel lines before exploring more complex relationships. |
Parallel Lines |
|
Math Clip Art: Parallel Lines Cut by a Transversal 10 | Math Clip Art: Parallel Lines Cut by a Transversal 10TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It showcases a fourth pair of corresponding angles, solidifying the concept that these angles are congruent when parallel lines are cut by a transversal. The image uses a distinct color coding to identify this final pair of corresponding angles, providing students with a comprehensive view of all possible corresponding angle pairs in this configuration. |
Parallel Lines |
|
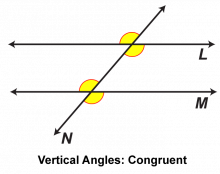
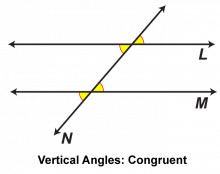
Math Clip Art: Parallel Lines Cut by a Transversal 11 | Math Clip Art: Parallel Lines Cut by a Transversal 11TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights vertical angles, demonstrating that these angles are congruent when any two lines intersect. The image uses color coding to clearly identify a pair of vertical angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
|
Math Clip Art: Parallel Lines Cut by a Transversal 12 | Math Clip Art: Parallel Lines Cut by a Transversal 12TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of vertical angles, reinforcing the concept that these angles are congruent when any two lines intersect. The image uses distinct color coding to identify this second pair of vertical angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
|
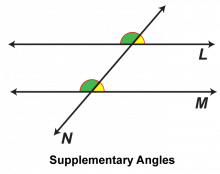
Math Clip Art: Parallel Lines Cut by a Transversal 13 | Math Clip Art: Parallel Lines Cut by a Transversal 13TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights supplementary angles, demonstrating that certain pairs of angles formed by parallel lines and a transversal are supplementary (their measures add up to 180°). The image uses color coding to clearly identify a pair of supplementary angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
|
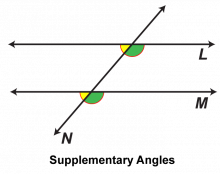
Math Clip Art: Parallel Lines Cut by a Transversal 14 | Math Clip Art: Parallel Lines Cut by a Transversal 14TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of supplementary angles, reinforcing the concept that certain pairs of angles formed by parallel lines and a transversal are supplementary. The image uses distinct color coding to identify this second pair of supplementary angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
|
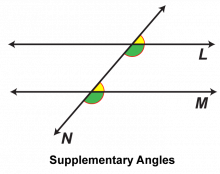
Math Clip Art: Parallel Lines Cut by a Transversal 15 | Math Clip Art: Parallel Lines Cut by a Transversal 15TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It showcases a third pair of supplementary angles, solidifying the concept that certain pairs of angles formed by parallel lines and a transversal are supplementary. The image uses a unique color coding to identify this pair of supplementary angles, providing students with multiple examples of this geometric relationship. |
Parallel Lines |

|
Math Clip Art: Parallel Lines Cut by a Transversal 16 | Math Clip Art: Parallel Lines Cut by a Transversal 16TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It showcases a fourth pair of supplementary angles, reinforcing the concept that certain pairs of angles formed by parallel lines and a transversal are supplementary (their measures add up to 180°). The image uses distinct color coding to identify this pair of supplementary angles, providing students with a comprehensive view of all possible supplementary angle pairs in this configuration. |
Parallel Lines |
|
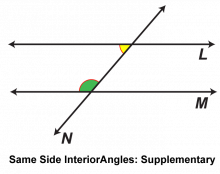
Math Clip Art: Parallel Lines Cut by a Transversal 17 | Math Clip Art: Parallel Lines Cut by a Transversal 17TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights same side interior angles, demonstrating that these angles are supplementary (their measures add up to 180°) when parallel lines are cut by a transversal. The image uses color coding to clearly identify a pair of same side interior angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
|
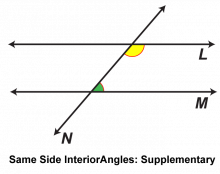
Math Clip Art: Parallel Lines Cut by a Transversal 18 | Math Clip Art: Parallel Lines Cut by a Transversal 18TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of same side interior angles, reinforcing the concept that these angles are supplementary when parallel lines are cut by a transversal. The image uses distinct color coding to identify this second pair of same side interior angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
|
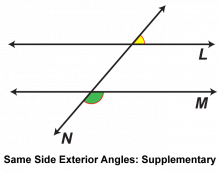
Math Clip Art: Parallel Lines Cut by a Transversal 19 | Math Clip Art: Parallel Lines Cut by a Transversal 19TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights same side exterior angles, demonstrating that these angles are supplementary (their measures add up to 180°) when parallel lines are cut by a transversal. The image uses color coding to clearly identify a pair of same side exterior angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
|
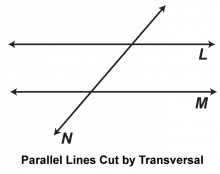
Math Clip Art: Parallel Lines Cut by a Transversal 2 | Math Clip Art: Parallel Lines Cut by a Transversal 2TopicGeometry DescriptionThis math clip art image is the second in a series illustrating the properties of parallel lines cut by a transversal. It shows two parallel lines intersected by a third line, known as a transversal. This visual representation is crucial for introducing students to the concept of parallel lines cut by a transversal and the various angle relationships that result from this configuration. |
Parallel Lines |

|
Math Clip Art: Parallel Lines Cut by a Transversal 20 | Math Clip Art: Parallel Lines Cut by a Transversal 20TopicGeometry DescriptionThis math clip art image is the final in a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of same side exterior angles, reinforcing the concept that these angles are supplementary when parallel lines are cut by a transversal. The image uses distinct color coding to identify this second pair of same side exterior angles, providing students with a comprehensive view of all angle relationships in this configuration. |
Parallel Lines |
|
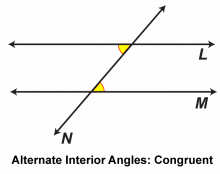
Math Clip Art: Parallel Lines Cut by a Transversal 3 | Math Clip Art: Parallel Lines Cut by a Transversal 3TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It specifically highlights alternate interior angles, showing that they are congruent when parallel lines are cut by a transversal. The image uses color coding to clearly identify the alternate interior angles, making it easier for students to visualize this important geometric relationship. |
Parallel Lines |
|
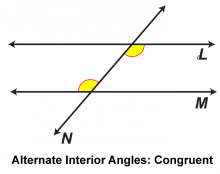
Math Clip Art: Parallel Lines Cut by a Transversal 4 | Math Clip Art: Parallel Lines Cut by a Transversal 4TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of alternate interior angles, reinforcing the concept that these angles are congruent when parallel lines are cut by a transversal. The image uses distinct color coding to identify this second pair of alternate interior angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
|
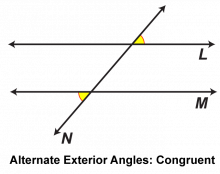
Math Clip Art: Parallel Lines Cut by a Transversal 5 | Math Clip Art: Parallel Lines Cut by a Transversal 5TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights alternate exterior angles, demonstrating that these angles are congruent when parallel lines are cut by a transversal. The image uses color coding to clearly identify the alternate exterior angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |
|
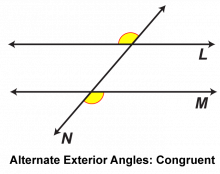
Math Clip Art: Parallel Lines Cut by a Transversal 6 | Math Clip Art: Parallel Lines Cut by a Transversal 6TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on alternate exterior angles, demonstrating that these angles are congruent when parallel lines are cut by a transversal. The image uses color coding to clearly identify another pair of alternate exterior angles, reinforcing the concept introduced in the previous image. |
Parallel Lines |
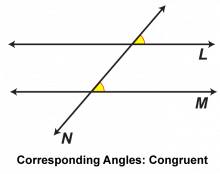
|
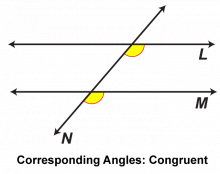
Math Clip Art: Parallel Lines Cut by a Transversal 7 | Math Clip Art: Parallel Lines Cut by a Transversal 7TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights corresponding angles, demonstrating that these angles are congruent when parallel lines are cut by a transversal. The image uses color coding to clearly identify a pair of corresponding angles, making it easier for students to visualize and understand this geometric relationship. |
Parallel Lines |

|
Math Clip Art: Parallel Lines Cut by a Transversal 8 | Math Clip Art: Parallel Lines Cut by a Transversal 8TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It focuses on another pair of corresponding angles, reinforcing the concept that these angles are congruent when parallel lines are cut by a transversal. The image uses distinct color coding to identify this second pair of corresponding angles, providing students with a clear visual representation of this geometric relationship. |
Parallel Lines |
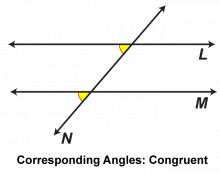
|
Math Clip Art: Parallel Lines Cut by a Transversal 9 | Math Clip Art: Parallel Lines Cut by a Transversal 9TopicGeometry DescriptionThis math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights yet another pair of corresponding angles, further reinforcing the concept that these angles are congruent when parallel lines are cut by a transversal. The image uses a unique color coding to identify this third pair of corresponding angles, providing students with multiple examples of this geometric relationship. |
Parallel Lines |
|
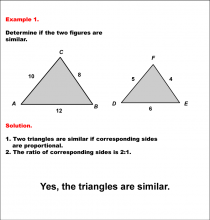
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 1 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 1TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are similar. Triangle ABC has sides 10, 8, and 12, while triangle DEF has sides 5, 4, and 6. Triangles are similar if corresponding sides are proportional. Here, the ratio of corresponding sides is 2:1 (10:5, 8:4, 12:6). Thus, the triangles are similar. Therefore, the answer is Yes, the triangles are similar. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 10 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 10TopicGeometric Shapes DescriptionDetermine if two right triangles are similar. The triangle on the left has angle 40°, and the other triangle has a 60° angle. For similarity, all corresponding angles must be congruent. The angles in each triangle do not match, so the triangles aren't similar. Therefore, the answer is No, the triangles aren't similar. |
Definition of a Triangle |
|
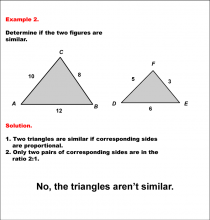
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 2 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 2TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are similar. Triangle ABC has sides 10, 8, and 12, while triangle DEF has sides 5, 3, and 6. Triangles are similar if all corresponding sides are proportional. Only two pairs of sides here have a 2:1 ratio, so the triangles are not similar. Therefore, the answer is No, the triangles arenÕt similar. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 3 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 3TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are similar. Both triangles have angles 60°, 57°, and 63°. Triangles are similar if corresponding angles are equal. Since all corresponding angles match, the triangles are similar. Therefore, the answer is Yes, the triangles are similar. Understanding the concept of geometric shapes is essential for developing geometric reasoning. These examples demonstrate how to analyze and verify similarity between shapes by comparing corresponding sides and angles. The worked-out examples provided help students visualize and understand this key concept. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 4 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 4TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are similar. Triangle ABC has angles 75°, 42°, and an unknown angle, and triangle DEF has angles 75° and 63° and an unknown angles. Triangles are similar if all corresponding angles are congruent. Solving for the unknown angles confirms angle congruency, so the triangles are similar. Therefore, the answer is Yes, the triangles are similar. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 5 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 5TopicGeometric Shapes DescriptionDetermine if two right triangles, ABC and DEF, are similar. Triangle ABC has angles 90° and 40°, and triangle DEF has angles 90° and 50°. Triangles are similar if all corresponding angles are congruent. Solving reveals congruent corresponding angles. Therefore, the answer is Yes, the triangles are similar. Understanding the concept of geometric shapes is essential for developing geometric reasoning. These examples demonstrate how to analyze and verify similarity between shapes by comparing corresponding sides and angles. The worked-out examples provided help students visualize and understand this key concept. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 6 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 6TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are similar. Triangle ABC has a right angle and an angle of 40°, and triangle DEF has a right angle and 45°. Not all corresponding angles are congruent, so the triangles are not similar. Therefore, the answer is No, the triangles aren't similar. Understanding the concept of geometric shapes is essential for developing geometric reasoning. These examples demonstrate how to analyze and verify similarity between shapes by comparing corresponding sides and angles. The worked-out examples provided help students visualize and understand this key concept. |
Definition of a Triangle |
|
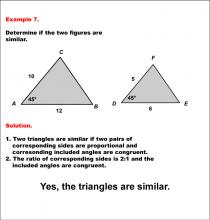
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 7 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 7TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are similar. Triangle ABC has sides 10 and 12 with a 45° angle, and triangle DEF has sides 5 and 6 with a 45° angle. Triangles are similar if two pairs of sides are proportional, and the included angle is congruent. The side ratio is 2:1, and both included angles are 45°. Therefore, the answer is Yes, the triangles are similar. |
Definition of a Triangle |
|
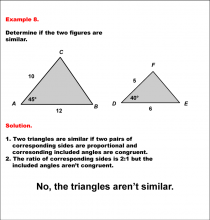
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 8 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 8TopicGeometric Shapes DescriptionDetermine if two triangles, ABC and DEF, are similar. Triangle ABC has sides 10 and 12 with a 45° angle, and triangle DEF has sides 5 and 6 with a 40° angle. Triangles are similar if two pairs of sides are proportional, and the included angles are congruent. Here, the included angles differ (45° vs. 40°), so the triangles are not similar. Therefore, the answer is No, the triangles aren't similar. |
Definition of a Triangle |

|
Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 9 | Math Example--Geometric Shapes--Analyzing Similar Shapes--Example 9TopicGeometric Shapes DescriptionDetermine if two right triangles are similar. The triangle on the left has sides 10 and 15 and an included right angle, while the other triangle has sides 5 and 7.5 and an included right angle. Triangles are similar if two pairs of corresponding sides are proportional, and corresponding included angles are congruent. The side ratio is 2:1, and the included right angles are congruent. Therefore, the answer is Yes, the triangles are similar. |
Definition of a Triangle |

|
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 1 | Equations Using the Exterior Angle Theorem--Example 1TopicEquations |
Applications of Triangles |

|
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 10 | Equations Using the Exterior Angle Theorem--Example 10TopicEquations DescriptionThis example illustrates a more complex application of the Exterior Angle Theorem in solving triangle-related equations. In this scenario, we have a triangle with one known interior angle of 35°, an unknown interior angle y, and an unknown exterior angle x. The Exterior Angle Theorem states that an exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles. Here, the equation is set up as x = 35° + y. Furthermore, the angles 3x and x are supplementary, allowing you to solve for x. Having solved for x, you can then solve for y. |
Applications of Triangles |

|
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 2 | Equations Using the Exterior Angle Theorem--Example 2TopicEquations |
Applications of Triangles |
|
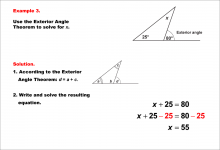
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 3 | Equations Using the Exterior Angle Theorem--Example 3TopicEquations |
Applications of Triangles |
|
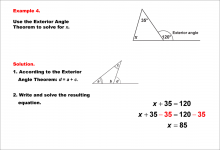
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 4 | Equations Using the Exterior Angle Theorem--Example 4TopicEquations |
Applications of Triangles |
|
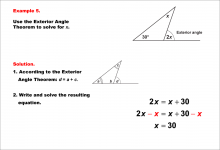
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 5 | Equations Using the Exterior Angle Theorem--Example 5TopicEquations |
Applications of Triangles |

|
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 6 | Equations Using the Exterior Angle Theorem--Example 6TopicEquations |
Applications of Triangles |

|
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 7 | Equations Using the Exterior Angle Theorem--Example 7TopicEquations |
Applications of Triangles |

|
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 8 | Equations Using the Exterior Angle Theorem--Example 8TopicEquations |
Applications of Triangles |

|
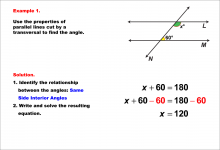
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 9 | Equations Using the Exterior Angle Theorem--Example 9TopicEquations DescriptionThis example presents a more challenging application of the Exterior Angle Theorem in solving triangle-related equations. In this scenario, we have a triangle with one known interior angle of 25°, an unknown interior angle y, and an unknown exterior angle x. The Exterior Angle Theorem states that an exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles. Here, the equation is set up as x = 25° + y. You can also use the fact that x and 2x are supplementary, allowing you to solve for x. By solving for x, you can then solve for y using the triangle equation. |
Applications of Triangles |
|
Math Example--Solving Equations--Equations with Angles from Parallel Lines Cut by a Transversal--Example 1 | Equations with Angles from Parallel Lines Cut by a Transversal--Example 1TopicEquations |
Parallel Lines, Applications of Angles and Planes and Applications of Equations and Inequalities |
|
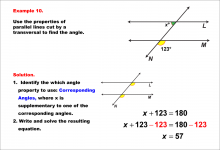
Math Example--Solving Equations--Equations with Angles from Parallel Lines Cut by a Transversal--Example 10 | Equations with Angles from Parallel Lines Cut by a Transversal--Example 10TopicEquations |
Parallel Lines, Applications of Angles and Planes and Applications of Equations and Inequalities |
|
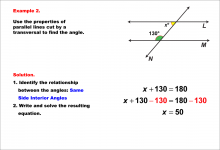
Math Example--Solving Equations--Equations with Angles from Parallel Lines Cut by a Transversal--Example 2 | Equations with Angles from Parallel Lines Cut by a Transversal--Example 2TopicEquations |
Parallel Lines, Applications of Angles and Planes and Applications of Equations and Inequalities |
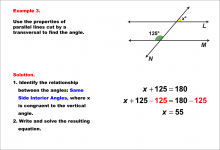
|
Math Example--Solving Equations--Equations with Angles from Parallel Lines Cut by a Transversal--Example 3 | TopicEquations |
Parallel Lines, Applications of Angles and Planes and Applications of Equations and Inequalities |