Illustrative Math Alignment: Grade 7 Unit 9

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "12 is what percent of 8?" The solution involves setting up the equation 8 * (x / 100) = 12, then solving for x to get x = 12 * (100 / 8), which equals 150%. This example demonstrates how to calculate a percentage when the first number is larger than the second, resulting in a percentage greater than 100%.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "What is 7% of 9.5?" The solution involves converting 7% to its decimal equivalent, 0.07, and then multiplying it by 9.5 to obtain the result of 0.665. This example builds upon the previous one by introducing a decimal number as the base value.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "32 is what percent of 1.5?" The solution involves setting up the equation 1.5 * (x / 100) = 32, then solving for x to get x = 32 * (100 / 1.5), which equals 2133.3%. This example introduces a scenario where the resulting percentage is significantly larger than 100% and involves a decimal base number less than 1.

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "48 is what percent of 55?" The solution involves setting up the equation 55 * (x / 100) = 48, then solving for x to get x = 48 * (100 / 55), which equals 87.27%. This example demonstrates how to calculate a percentage when the two numbers are relatively close in value, resulting in a percentage close to but less than 100%.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "52.2 is what percent of 98.5?" The solution involves setting up the equation 98.5 * (x / 100) = 52.2, then solving for x to get x = 52.2 * (100 / 98.5), which is approximately 52.99%. This example introduces a scenario where both the numerator and denominator are decimal numbers, resulting in a percentage that is also close to the original numerator.

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "68 is what percent of 320?" The solution involves setting up the equation 320 * (x / 100) = 68, then solving for x to get x = 68 * (100 / 320), which equals 21.25%. This example demonstrates how to calculate a percentage when dealing with whole numbers, resulting in a percentage that's less than 25%.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "75.5 is what percent of 555.25?" The solution involves setting up the equation 555.25 * (x / 100) = 75.5, then solving for x to get x = 75.5 * (100 / 555.25), which is approximately 13.59%. This example introduces a scenario where both the numerator and denominator are decimal numbers, resulting in a percentage that's less than 15%.

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "125 is what percent of 2?" The solution involves setting up the equation 2 * (x / 100) = 125, then solving for x to get x = 125 * (100 / 2), which equals 6250%. This example demonstrates how to calculate a percentage when the first number is significantly larger than the second, resulting in a percentage well over 100%.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "300 is what percent of 3.5?" The solution involves setting up the equation 3.5 * (x / 100) = 300, then solving for x to get x = 300 * (100 / 3.5), which equals 8571.43%. This example introduces a scenario where the resulting percentage is extremely large, over 8000%, due to the first number being significantly larger than the small decimal base number.

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "278 is what percent of 99?" The solution involves setting up the equation 99 * (x / 100) = 278, then solving for x to get x = 278 * (100 / 99), which equals 280.80%. This example demonstrates how to calculate a percentage when the first number is significantly larger than the second, resulting in a percentage greater than 200%.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "300 is what percent of 75.5?" The solution involves setting up the equation 75.5 * (x / 100) = 300, then solving for x to get x = 300 * (100 / 75.5), which equals 397.35%. This example introduces a scenario where the resulting percentage is close to 400%, with the first number being significantly larger than the decimal base number.

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "400 is what percent of 220?" The solution involves setting up the equation 220 * (x / 100) = 400, then solving for x to get x = 400 * (100 / 220), which equals 181.81%. This example demonstrates how to calculate a percentage when the first number is nearly double the second, resulting in a percentage between 150% and 200%.

Topic

Solving Equations

Description

This math example focuses on solving percent equations, specifically asking "What is 8% of 58?" The solution involves converting 8% to its decimal form, 0.08, and then multiplying it by 58 to arrive at the answer of 4.64. This example introduces a larger whole number as the base value, demonstrating the scalability of the percent-to-decimal conversion method.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "333.5 is what percent of 500.25?" The solution involves setting up the equation 500.25 * (x / 100) = 333.5, then solving for x to get x = 333.5 * (100 / 500.25), which is approximately 66.67%. This example introduces a scenario where both numbers are decimals and the resulting percentage is less than 100%, showing how to handle more complex decimal calculations.

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "4 is 0.1% of what number?" The solution involves setting up the equation 4 = 0.001 * x, then solving for x to get x = 4 / 0.001, which equals 4000. This example demonstrates how to calculate the whole when given a very small percentage of it, resulting in a much larger number.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "7 is 1% of what number?" The solution involves setting up the equation 7 = 0.01 * x, then solving for x to get x = 7 / 0.01, which equals 700. This example introduces a scenario where we need to find the whole when given a small percentage of it, resulting in a number 100 times larger than the given value.

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "9 is 30% of what number?" The solution involves setting up the equation 9 = 0.3 * x, then solving for x to get x = 9 / 0.3, which equals 30. This example demonstrates how to calculate the whole when given a larger percentage of it, resulting in a number that is only slightly larger than the given value.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "1 is 400% of what number?" The solution involves setting up the equation 1 = 4.0 * x, then solving for x to get x = 1 / 4, which equals 0.25. This example introduces a scenario where we need to find a number that, when increased by 400%, results in 1, leading to a fraction or decimal less than 1.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "15 is 0.25% of what number?" The solution involves setting up the equation 15 = 0.0025 * x, then solving for x to get x = 15 / 0.0025, which equals 6000. This example introduces a scenario where we need to find the whole when given a very small percentage of it, resulting in a number that is significantly larger than the given value.

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "30 is 5% of what number?" The solution involves setting up the equation 30 = 0.05 * x, then solving for x to get x = 30 / 0.05, which equals 600. This example demonstrates how to calculate the whole when given a small percentage of it, resulting in a much larger number.

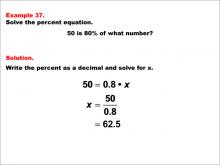
Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "50 is 80% of what number?" The solution involves setting up the equation 50 = 0.8 * x, then solving for x to get x = 50 / 0.8, which equals 62.5. This example introduces a scenario where we need to find the whole when given a large percentage of it, resulting in a number that is only slightly larger than the given value.

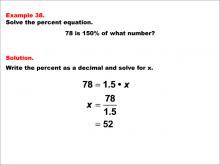
Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "78 is 150% of what number?" The solution involves setting up the equation 78 = 1.5 * x, then solving for x to get x = 78 / 1.5, which equals 52. This example demonstrates how to calculate the original value when given a percentage greater than 100%, resulting in a number that is smaller than the given value.

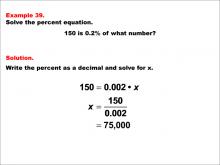
Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "150 is 0.2% of what number?" The solution involves setting up the equation 150 = 0.002 * x, then solving for x to get x = 150 / 0.002, which equals 75,000. This example introduces a scenario where we need to find the whole when given a very small percentage of it, resulting in a number that is significantly larger than the given value.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "What is 6.5% of 45.5?" The solution involves converting 6.5% to its decimal equivalent, 0.065, and then multiplying it by 45.5 to obtain the result of 2.9575. This example introduces both a decimal percentage and a decimal base number, adding complexity to the calculation.

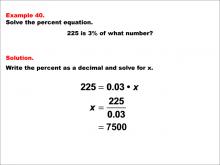
Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "225 is 3% of what number?" The solution involves setting up the equation 225 = 0.03 * x, then solving for x to get x = 225 / 0.03, which equals 7,500. This example demonstrates how to calculate the whole when given a small percentage of it, resulting in a number that is significantly larger than the given value.

Topic

Solving Equations

Description

This math example focuses on solving percent equations by asking "400 is 40% of what number?" The solution involves setting up the equation 400 = 0.4 * x, then solving for x to get x = 400 / 0.4, which equals 1000. This example demonstrates how to calculate the whole when given a significant percentage of it, resulting in a number that is larger than the given value.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "650 is 130% of what number?" The solution involves setting up the equation 650 = 1.3 * x, then solving for x to get x = 650 / 1.3, which equals 500. This example introduces a scenario where we need to find the original value when given a percentage greater than 100%, resulting in a number that is smaller than the given value.

Topic

Solving Equations

Description

This math example focuses on solving percent equations, specifically asking "What is 30% of 9?" The solution involves converting 30% to its decimal form, 0.3, and then multiplying it by 9 to get the result of 2.7. This example introduces a larger percentage, demonstrating how the method applies consistently across various percentage values.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "What is 28% of 7.2?" The solution involves converting 28% to its decimal equivalent, 0.28, and then multiplying it by 7.2 to obtain the result of 2.016. This example combines a whole number percentage with a decimal base number, further illustrating the versatility of the percent-to-decimal conversion method.

Topic

Solving Equations

Description

This math example focuses on solving percent equations, specifically asking "What is 45% of 68?" The solution involves converting 45% to its decimal form, 0.45, and then multiplying it by 68 to arrive at the answer of 30.6. This example introduces a larger percentage and a larger whole number as the base value, demonstrating the scalability of the percent-to-decimal conversion method.

Topic

Solving Equations

Description

This math example demonstrates solving percent equations by asking "What is 52.3% of 36.9?" The solution involves converting 52.3% to its decimal equivalent, 0.523, and then multiplying it by 36.9 to obtain the result of 19.2987. This example introduces both a decimal percentage and a decimal base number, adding complexity to the calculation and showcasing the versatility of the percent-to-decimal conversion method.

Topic

Solving Equations

Description

This math example focuses on solving percent equations, specifically asking "What is 150% of 8?" The solution involves converting 150% to its decimal form, 1.5, and then multiplying it by 8 to get the result of 12. This example introduces a percentage greater than 100%, demonstrating how the method applies consistently even when dealing with percentages that represent values larger than the whole.

This is part of a collection of math examples that focus on percents.

This is part of a collection of math examples that focus on percents.

This is part of a collection of math examples that focus on percents.

This is part of a collection of math examples that focus on percents.

This is part of a collection of math examples that focus on percents.

This is part of a collection of math examples that focus on percents.

This is part of a collection of math examples that focus on percents.

This is part of a collection of math examples that focus on percents.

This is part of a collection of math examples that focus on percents.

This is part of a collection of math examples that focus on percents.

Topic

Polygons

Description

This example showcases a pentagon with all sides labeled as 5. It demonstrates a regular pentagon, characterized by five sides of equal length. This visual representation helps students understand the concept of regular polygons and their defining characteristics.

The topic of polygon classification is crucial in geometry. This collection of examples provides a comprehensive look at various types of pentagons, both regular and irregular. By examining different configurations of sides and angles, students can develop a deeper understanding of polygon properties and classification criteria.

Topic

Polygons

Description

This example showcases a pentagon labeled with vertices A, B, C, D, E, where the angles are marked as 110°, 95°, 120°, 107°, and 108°. It demonstrates an irregular pentagon based on the variation in angle measures, highlighting that regularity depends on both side lengths and angle measures.

Understanding polygon classification is essential in geometry as it helps students recognize and categorize shapes based on their properties. This collection of examples emphasizes the importance of both side lengths and angle measures in determining the regularity of pentagons.

Topic

Polygons

Description

This example presents a pentagon labeled with vertices A, B, C, D, E, where the sides are marked with variables w, x, y, z, and v. It illustrates an irregular pentagon by using different variables to represent potentially different side lengths, emphasizing that side length variation results in irregularity.

Polygon classification is a fundamental concept in geometry that helps students analyze and categorize shapes based on their properties. This collection of examples provides a comprehensive look at various types of pentagons, highlighting the importance of side length equality in determining regularity.

Topic

Polygons

Description

This example features a pentagon labeled with vertices A, B, C, D, E, where the angles are marked as 108°, x, y, z, and 107°. It demonstrates an irregular pentagon based on the variation in angle measures, highlighting that regularity depends on all angles being equal.

Understanding polygon classification is essential in geometry as it helps students recognize and categorize shapes based on their properties. This collection of examples emphasizes the importance of angle measures in determining the regularity of pentagons, showing that even one different angle makes the polygon irregular.

Topic

Polygons

Description

This example showcases a hexagon with all sides labeled as 5, indicating equal length. It demonstrates a regular hexagon, characterized by six sides of equal length. This visual representation helps students understand the concept of regular polygons and their defining characteristics.

The topic of polygon classification is crucial in geometry. This collection of examples provides a comprehensive look at various types of hexagons, both regular and irregular. By examining different configurations of sides and angles, students can develop a deeper understanding of polygon properties and classification criteria.

Topic

Polygons

Description

This example presents a hexagon with all sides labeled as x, indicating equal length. It illustrates another instance of a regular hexagon, reinforcing the concept that a regular polygon has all sides of equal length, even when represented by a variable.

Polygon classification is a fundamental topic in geometry that helps students understand the properties and characteristics of different shapes. This collection of examples provides a comprehensive overview of various hexagon types, allowing students to distinguish between regular and irregular polygons based on their side lengths and angle measures.

Topic

Polygons

Description

This example features a hexagon with sides labeled y, x, and 5, showing that x = y = 5, making all sides equal in length. It demonstrates a regular hexagon by using a combination of variables and numeric values to represent equal side lengths.

Understanding polygon classification is crucial in geometry as it helps students recognize and categorize shapes based on their properties. This collection of examples highlights different ways to represent regular hexagons, emphasizing the importance of side length equality in classification.

Topic

Polygons

Description

This example showcases a hexagon with all interior angles labeled as 120 degrees, indicating equal angles. It demonstrates that a regular hexagon can be identified not only by equal side lengths but also by equal angle measures, reinforcing the dual criteria for regularity in polygons.

Polygon classification is a fundamental concept in geometry that helps students analyze and categorize shapes based on their properties. This collection of examples provides a comprehensive look at various aspects of regular hexagons, emphasizing both side length and angle measure as defining characteristics.