Illustrative Math Alignment: Grade 8 Unit 6

Associations in Data

Lesson 2: Plotting Data

Use the following Media4Math resources with this Illustrative Math lesson.

Title	Body	Curriculum Topic
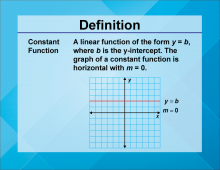
Definition--Linear Function Concepts--Constant Function	Constant Function Topic Linear Functions Definition A constant function is a linear function of the form f(x) = b, where b is a constant. The graph of a constant function is a horizontal line. Description Constant functions are a fundamental concept in linear functions. They represent scenarios where the output value remains unchanged, regardless of the input value. This is depicted graphically as a horizontal line, indicating that the function's rate of change is zero. In real-world applications, constant functions can model situations where a quantity remains steady over time. For example, a flat fee service charge that does not vary with usage can be represented as a constant function.	Slope-Intercept Form
Definition--Linear Function Concepts--Converting from Standard Form to Slope-Intercept Form	Converting from Standard Form to Slope-Intercept Form Topic Linear Functions Definition Converting from standard form to slope-intercept form involves rewriting a linear equation from the form Ax + By = C to the form y = mx + b, where m is the slope and b is the y-intercept. Description Converting linear equations from standard form to slope-intercept form is a key skill in algebra. This conversion allows for easier graphing and interpretation of the equation's slope and y-intercept.	Slope-Intercept Form
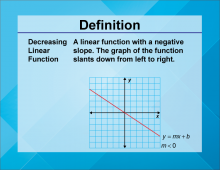
Definition--Linear Function Concepts--Decreasing Linear Function	Decreasing Linear Function Topic Linear Functions Definition A decreasing linear function is a linear function where the slope is negative, indicating that as the input value increases, the output value decreases. Description Decreasing linear functions are important in understanding how variables inversely relate to each other. The negative slope signifies a reduction in the dependent variable as the independent variable increases. Real-world examples include depreciation of assets over time or the decrease in temperature as altitude increases. These functions help model scenarios where an increase in one quantity results in a decrease in another.	Slope-Intercept Form
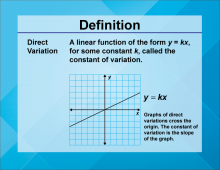
Definition--Linear Function Concepts--Direct Variation	Direct Variation Topic Linear Functions Definition Direct variation describes a linear relationship between two variables where one variable is a constant multiple of the other, expressed as y = kx, where k is the constant of variation. Description Direct variation is a fundamental concept in linear functions, illustrating how one variable changes proportionally with another. The constant of variation, k, represents the rate of change. In real-world scenarios, direct variation can model relationships such as speed and distance, where distance traveled varies directly with time at a constant speed. Understanding this concept is crucial in fields like physics and engineering.	Slope-Intercept Form
Definition--Linear Function Concepts--Equations of Parallel Lines	Equations of Parallel Lines Topic Linear Functions Definition Equations of parallel lines are linear equations that have the same slope but different y-intercepts, indicating that the lines never intersect. Description Understanding equations of parallel lines is crucial in geometry and algebra. Parallel lines have identical slopes, which means they run in the same direction and never meet. In real-world applications, parallel lines can model scenarios such as railway tracks or lanes on a highway, where maintaining a consistent distance is essential.	Slope-Intercept Form
Definition--Linear Function Concepts--Equations of Perpendicular Lines	Equations of Perpendicular Lines Topic Linear Functions Definition Equations of perpendicular lines are linear equations where the slopes are negative reciprocals of each other, indicating that the lines intersect at a right angle. Description Equations of perpendicular lines are significant in both geometry and algebra. The negative reciprocal relationship between their slopes ensures that the lines intersect at a 90-degree angle. In real-world applications, perpendicular lines are found in various structures, such as the intersection of streets or the corners of a building, where right angles are essential.	Slope-Intercept Form
Definition--Linear Function Concepts--Identity Function	Identity Function Topic Linear Functions Definition An identity function is a linear function of the form f(x) = x, where the output is equal to the input for all values of x. Description The identity function is a basic yet crucial concept in linear functions. It represents a scenario where the input value is always equal to the output value, graphically depicted as a 45-degree line passing through the origin. In real-world applications, the identity function can model situations where input and output are directly proportional and identical, such as converting units of the same measure. This also introduces the concept of identity, which is fundamental to mathematics.	Slope-Intercept Form
Definition--Linear Function Concepts--Increasing Linear Function	Increasing Linear Function Topic Linear Functions Definition An increasing linear function is a linear function where the slope is positive, indicating that as the input value increases, the output value also increases. Description Increasing linear functions are essential in understanding how variables positively relate to each other. The positive slope signifies an increase in the dependent variable as the independent variable increases. Real-world examples include income increasing with hours worked or the rise in temperature with the increase in daylight hours. These functions help model scenarios where an increase in one quantity results in an increase in another.	Slope-Intercept Form
Definition--Linear Function Concepts--Line of Best Fit	Line of Best Fit Topic Linear Functions Definition A line of best fit is a straight line that best represents the data on a scatter plot, showing the trend of the data points. Description The line of best fit is a crucial concept in statistics and data analysis. It helps in identifying the trend and making predictions based on the data. In real-world applications, the line of best fit is used in various fields such as economics, biology, and engineering to analyze trends and make forecasts. For example, it can be used to predict future sales based on past data.	Graphs of Linear Functions
Definition--Linear Function Concepts--Linear Equations in Standard Form	Linear Equations in Standard Form Topic Linear Functions Definition Linear equations in standard form are written as Ax + By = C, where A, B, and C are constants, and A and B are not both zero. Description Linear equations in standard form are a fundamental representation of linear functions. They provide a way to express linear relationships in a general form.	Standard Form
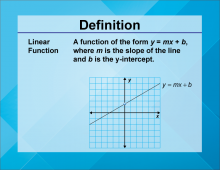
Definition--Linear Function Concepts--Linear Function	Linear Function Topic Linear Functions Definition A linear function is a function that can be graphed as a straight line, typically written in the form y = mx + b, where m is the slope and b is the y-intercept. Description Linear functions are one of the most fundamental concepts in mathematics. They describe relationships where the rate of change between variables is constant, represented graphically as a straight line. This simplicity makes them a central topic in algebra and calculus.	Slope-Intercept Form
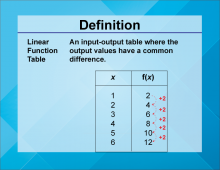
Definition--Linear Function Concepts--Linear Function Tables	Linear Function Tables Topic Linear Functions Definition Linear function tables display the input-output pairs of a linear function, showing how the dependent variable changes with the independent variable. Description Linear function tables are useful tools for understanding and analyzing linear relationships. They provide a clear way to see how changes in the input (independent variable) affect the output (dependent variable).	Applications of Linear Functions and Graphs of Linear Functions
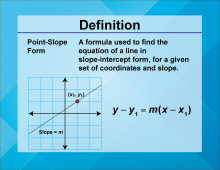
Definition--Linear Function Concepts--Point-Slope Form	Point-Slope Form Topic Linear Functions Definition Point-slope form of a linear equation is written as y − y1 = m(x−x1 ), where m is the slope and (x1 ,y1) is a point on the line. Description The point-slope form is a versatile way to express linear equations, especially useful when you know a point on the line and the slope. It allows for quick construction of the equation of a line.	Point-Slope Form
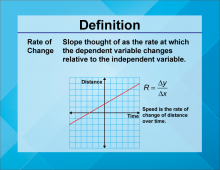
Definition--Linear Function Concepts--Rate of Change	Rate of Change Topic Linear Functions Definition Rate of change in a linear function is the ratio of the change in the dependent variable to the change in the independent variable, often represented as the slope m in the equation y = mx + b. Description Rate of change is a fundamental concept in understanding linear functions. It describes how one variable changes in relation to another, and is graphically represented by the slope of a line.	Slope
Definition--Linear Function Concepts--Slope-Intercept Form	Slope-Intercept Form Topic Linear Functions Definition Slope-intercept form of a linear equation is written as y = mx + b, where m is the slope and b is the y-intercept. Description Slope-intercept form is one of the most commonly used forms of linear equations. It provides a clear way to understand the slope and y-intercept of a line, making it easier to graph and interpret. In real-world applications, slope-intercept form is used in various fields such as economics, physics, and engineering to model linear relationships. For example, it can represent the relationship between cost and production levels in business.	Slope-Intercept Form
Definition--Linear Function Concepts--The Equation of a Line From Two Coordinates	The Equation of a Line From Two Coordinates Topic Linear Functions Definition The equation of a line from two coordinates can be determined by finding the slope between the two points and using it in the point-slope form of a linear equation. Description Finding the equation of a line from two coordinates is a fundamental skill in algebra. It involves calculating the slope between the two points and then using one of the points to form the equation in point-slope or slope-intercept form.	Point-Slope Form
Definition--Linear Function Concepts--x-Intercept	x-Intercept Topic Linear Functions Definition The x-intercept is the point where a graph crosses the x-axis, indicating the value of x when 𝑦 y is zero. Description The x-intercept is a key concept in understanding the behavior of linear functions. It represents the point where the function's output is zero, providing insight into the function's roots and behavior. In real-world applications, the x-intercept can be used to determine break-even points in business, where revenue equals costs, or to find the time at which a process starts or stops.	Slope-Intercept Form
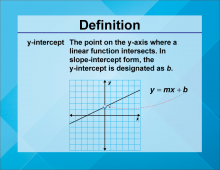
Definition--Linear Function Concepts--y-Intercept	y-Intercept Topic Linear Functions Definition The y-intercept is the point where a graph crosses the y-axis, indicating the value of y when x is zero. Description The y-intercept is a fundamental concept in understanding the behavior of linear functions. It represents the initial value of the function when the input is zero, providing insight into the function's starting point. In real-world applications, the y-intercept can be used to determine initial conditions in various scenarios, such as the starting balance in a bank account or the initial position of an object in motion.	Slope-Intercept Form
Definition--Sequences and Series Concepts--Arithmetic Sequence	Arithmetic Sequence Topic Sequences and Series Definition An arithmetic sequence is a sequence of numbers in which the difference between consecutive terms is constant. Description An arithmetic sequence is a fundamental concept in mathematics, particularly in the study of sequences and series. It is defined by the property that each term after the first is the sum of the previous term and a constant, known as the common difference. This concept is crucial in various mathematical applications, including solving problems related to linear growth and predicting future events based on past data.	Sequences
Definition--Sequences and Series Concepts--Arithmetic Series	Arithmetic Series Topic Sequences and Series Definition An arithmetic series is the sum of the terms of an arithmetic sequence. Description An arithmetic series is a significant concept in mathematics, especially in the study of sequences and series. It is formed by adding the terms of an arithmetic sequence. This concept is crucial for understanding how sums of linear patterns are calculated, which has applications in various fields such as finance, engineering, and computer science.	Series
Definition--Sequences and Series Concepts--Binomial Series	Binomial Series Topic Sequences and Series Definition The binomial series is the expansion of a binomial raised to any integer power. Description The binomial series is a powerful tool in mathematics, particularly in the study of sequences and series. It represents the expansion of a binomial expression raised to any integer power, which is essential in various mathematical and scientific applications, including probability, algebra, and calculus.	Series
Definition--Sequences and Series Concepts--Binomial Theorem	Binomial Theorem Topic Sequences and Series Definition The binomial theorem describes the algebraic expansion of powers of a binomial. Description The binomial theorem is a fundamental theorem in algebra that provides a formula for expanding binomials raised to any positive integer power. This theorem is essential in various mathematical disciplines, including algebra, calculus, and probability theory.	Series
Definition--Sequences and Series Concepts--Common Difference	Common Difference Topic Sequences and Series Definition The common difference is the difference between consecutive terms in an arithmetic sequence. Description The common difference is a key concept in arithmetic sequences, representing the consistent interval between consecutive terms. This concept is fundamental in understanding linear patterns and is widely used in various mathematical applications.	Sequences
Definition--Sequences and Series Concepts--Common Ratio	Common Ratio Topic Sequences and Series Definition The common ratio is the ratio between consecutive terms in a geometric sequence. Description The common ratio is a fundamental concept in geometric sequences, representing the consistent factor by which each term is multiplied to obtain the next term. This concept is crucial in understanding exponential growth and decay, which are prevalent in various mathematical and scientific applications.	Sequences
Definition--Sequences and Series Concepts--Convergent Series	Convergent Series Topic Sequences and Series Definition A convergent series is a series whose terms approach a specific value as the number of terms increases. Description A convergent series is a critical concept in the study of sequences and series, where the sum of its terms approaches a finite limit as the number of terms increases. This concept is essential in various mathematical and scientific applications, including calculus and analysis.	Series
Definition--Sequences and Series Concepts--Divergent Series	Divergent Series Topic Sequences and Series Definition A divergent series is a series whose terms do not approach a specific value as the number of terms increases. Description A divergent series is a significant concept in the study of sequences and series, where the sum of its terms does not approach a finite limit as the number of terms increases. This concept is essential in various mathematical and scientific applications, including calculus and analysis.	Series
Definition--Sequences and Series Concepts--Explicit Formula for a Sequence	Explicit Formula for a Sequence Topic Sequences and Series Definition An explicit formula for a sequence provides a direct way to calculate any term in the sequence. Description An explicit formula for a sequence is a mathematical expression that allows for the direct computation of any term in the sequence without needing to know the previous terms. This concept is fundamental in the study of sequences and series and is widely used in various mathematical applications.	Sequences
Definition--Sequences and Series Concepts--Fibonacci Sequence	Fibonacci Sequence Topic Sequences and Series Definition The Fibonacci sequence is a sequence where each term is the sum of the two preceding ones, starting from 0 and 1. Description The Fibonacci sequence is a famous sequence in mathematics, where each term is the sum of the two preceding ones, starting from 0 and 1. This sequence is significant in various mathematical and scientific applications, including computer science, biology, and art.	Sequences
Definition--Sequences and Series Concepts--Finite Sequence	Finite Sequence Topic Sequences and Series Definition A finite sequence is a sequence that has a limited number of terms. Description A finite sequence is a sequence with a specific number of terms, making it a crucial concept in the study of sequences and series. This concept is fundamental in various mathematical applications where the number of elements is limited, such as in statistics and computer science. In real-world applications, finite sequences are used in data analysis, where datasets have a specific number of entries. They are also used in computer algorithms that process a fixed number of elements. Algebraically, a finite sequence can be represented as	Sequences
Definition--Sequences and Series Concepts--Finite Series	Finite Series Topic Sequences and Series Definition A finite series is the sum of the terms of a finite sequence. Description A finite series is a significant concept in mathematics, representing the sum of the terms of a finite sequence. This concept is essential in various mathematical and scientific applications, including statistics, finance, and computer science. In real-world applications, finite series are used in financial calculations, such as determining the total amount of payments over a fixed period. They are also used in data analysis to find the sum of a dataset. Algebraically, a finite series can be expressed as	Series
Definition--Sequences and Series Concepts--Geometric Sequence	Geometric Sequence Topic Sequences and Series Definition A geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous term by a fixed, non-zero number called the common ratio. Description A geometric sequence is a fundamental concept in mathematics, particularly in the study of sequences and series. It is defined by the property that each term after the first is the product of the previous term and a constant, known as the common ratio. This concept is crucial in various mathematical applications, including exponential growth and decay.	Sequences
Definition--Sequences and Series Concepts--Geometric Series	Geometric Series Topic Sequences and Series Definition A geometric series is the sum of the terms of a geometric sequence. Description A geometric series is a significant concept in mathematics, especially in the study of sequences and series. It is formed by adding the terms of a geometric sequence. This concept is crucial for understanding how sums of exponential patterns are calculated, which has applications in various fields such as finance, engineering, and computer science.	Series
Definition--Sequences and Series Concepts--Graphs of Arithmetic Sequences	Graphs of Arithmetic Sequences Topic Sequences and Series Definition Graphs of arithmetic sequences form a linear graph on a coordinate plane. Description Graphs of arithmetic sequences are a visual tool used in mathematics to represent the terms of an arithmetic sequence on a coordinate plane. This concept is crucial for understanding linear patterns and relationships in sequences and series.	Sequences
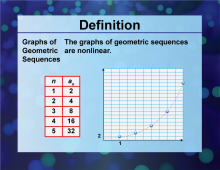
Definition--Sequences and Series Concepts--Graphs of Geometric Sequences	Graphs of Geometric Sequences Topic Sequences and Series Definition Graphs of geometric sequences visually represent the terms of a geometric sequence on a coordinate plane. These are nonlinear graphs. Description Graphs of geometric sequences are a visual tool used in mathematics to represent the terms of a geometric sequence on a coordinate plane. This concept is crucial for understanding exponential patterns and relationships in sequences and series.	Sequences
Definition--Sequences and Series Concepts--Infinite Sequence	Infinite Sequence Topic Sequences and Series Definition An infinite sequence is a sequence that continues indefinitely without terminating. Description An infinite sequence is a fundamental concept in mathematics, representing a sequence that continues indefinitely without terminating. This concept is essential in various mathematical and scientific applications, including calculus and analysis. In real-world applications, infinite sequences are used in mathematical modeling and simulations where processes continue indefinitely, such as in population dynamics or financial forecasting. Algebraically, an infinite sequence can be represented as	Sequences
Definition--Sequences and Series Concepts--Infinite Series	Infinite Series Topic Sequences and Series Definition An infinite series is the sum of the terms of an infinite sequence. Description An infinite series is a significant concept in mathematics, representing the sum of the terms of an infinite sequence. This concept is essential in various mathematical and scientific applications, including calculus and analysis. In real-world applications, infinite series are used in mathematical modeling and simulations where processes continue indefinitely, such as in signal processing or financial forecasting. Algebraically, an infinite series can be represented as	Series
Definition--Sequences and Series Concepts--Pascal's Triangle	Pascal's Triangle Topic Sequences and Series Definition Pascal's Triangle is a triangular array of binomial coefficients. Description Pascal's Triangle is a fundamental concept in mathematics, representing a triangular array of binomial coefficients. This concept is essential in various mathematical and scientific applications, including combinatorics, algebra, and probability theory. In real-world applications, Pascal's Triangle is used in probability calculations, particularly in binomial expansions and combinatorial problems. It is also used in computer science for algorithm design. Algebraically, the elements of Pascal's Triangle can be expressed as	Series
Definition--Sequences and Series Concepts--Recursive Formula	Recursive Formula Topic Sequences and Series Definition A recursive formula defines each term of a sequence using the preceding term(s). Description A recursive formula is a fundamental concept in mathematics, representing a way to define each term of a sequence using the preceding term(s). This concept is essential in various mathematical and scientific applications, including computer science and algorithm design.	Series
Definition--Sequences and Series Concepts--Sequences	Sequences Topic Sequences and Series Definition A sequence is an ordered list of numbers that follow a particular pattern or rule. Description Sequences are fundamental concepts in mathematics, representing ordered lists of numbers that follow a specific pattern or rule. This concept is crucial in various mathematical applications, including algebra, calculus, and computer science.	Sequences
Definition--Sequences and Series Concepts--Series	Series Topic Sequences and Series Definition A series is the sum of the terms of a sequence. Description Series are significant concepts in mathematics, representing the sum of the terms of a sequence. This concept is essential in various mathematical and scientific applications, including calculus, analysis, and financial modeling. In real-world applications, series are used in financial calculations, such as determining the total amount of payments or investments over time. They are also used in data analysis to find the sum of a dataset. Algebraically, series can be represented in various forms, such as arithmetic series, geometric series, and more.	Series
Definition--Sequences and Series Concepts--Square Numbers	Square Numbers Topic Sequences and Series Definition Square numbers are numbers that are the square of an integer. Description Square numbers are fundamental concepts in mathematics, representing numbers that are the square of an integer. This concept is crucial in various mathematical applications, including algebra, geometry, and number theory. In real-world applications, square numbers are used in area calculations, particularly in determining the area of squares. They are also used in computer algorithms and cryptography. Algebraically, square numbers can be represented as n2 , where n is an integer.	Sequences
Definition--Sequences and Series Concepts--Summation Notation	Summation Notation Topic Sequences and Series Definition Summation notation is a mathematical notation used to represent the sum of a sequence of terms. Description Summation notation is a powerful tool in mathematics, representing the sum of a sequence of terms in a compact form. This concept is essential in various mathematical and scientific applications, including calculus, statistics, and computer science.	Series
Definition--Sequences and Series Concepts--Triangular Numbers	Triangular Numbers Topic Sequences and Series Definition Triangular numbers are numbers that can be represented by an equilateral triangle. Description Triangular numbers are fundamental concepts in mathematics, representing numbers that can form an equilateral triangle. This concept is crucial in various mathematical applications, including algebra, geometry, and number theory. In real-world applications, triangular numbers are used in combinatorial problems and in determining the number of connections in a network. They are also used in computer algorithms and game design. Algebraically, triangular numbers can be represented as	Sequences
Definition--Statistics and Probability Concepts--Addition Rule of Probability	Addition Rule of Probability Topic Statistics and Probability Definition The Addition Rule of Probability is a key concept in statistics that helps in understanding the interaction between different events. Description The Addition Rule of Probability is crucial in the field of statistics because it allows us to make informed decisions based on the relationships between variables. For instance, in real-world applications, this concept is essential in various fields such as economics, social sciences, and health studies. Understanding how events affect each other can lead to better predictions and strategies.	Data Analysis
Definition--Statistics and Probability Concepts--Bivariate Data	Bivariate Data Topic Statistics and Probability Definition Bivariate data involves the analysis of two variables to determine relationships between them. Description Bivariate data is essential in statistics as it allows for the exploration of relationships between two variables, such as height and weight. This analysis is used in various fields, including economics, biology, and social sciences, to understand correlations and causations. For example, a scatter plot can be used to visually represent bivariate data, helping to identify trends or patterns.	Data Analysis
Definition--Statistics and Probability Concepts--Causation	Causation Topic Statistics and Probability Definition Causation refers to the relationship between two events where one event is affected by the other. Description Causation is a fundamental concept in statistics that distinguishes between correlation and causation. Understanding causation is vital in fields like medicine and social sciences to establish cause-effect relationships. For example, clinical trials are designed to establish causation between treatments and outcomes. Grasping causation is important for students to critically evaluate research findings and understand the implications of statistical analyses.	Data Analysis
Definition--Statistics and Probability Concepts--Combination 1	Combination 1 Topic Statistics and Probability Definition A combination is a selection of items from a larger pool where order does not matter. Description Combinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is where n is the total number of items, and r is the number of items to choose.	Data Analysis
Definition--Statistics and Probability Concepts--Combination 2	Combination 2 Topic Statistics and Probability Definition A combination is a selection of items from a larger pool where order does not matter. Description Combinations are used in probability to determine the number of ways to select items from a set, which is crucial in fields like cryptography and game theory. The formula for combinations is where n is the total number of items, and r is the number of items to choose.	Probability
Definition--Statistics and Probability Concepts--Compound Events	Compound Events Topic Statistics and Probability Definition A compound event in probability is an event that consists of two or more simple events. Description Compound events are important in probability as they allow for the calculation of the likelihood of multiple events occurring together, which is applicable in areas like risk assessment and decision-making. Understanding compound events helps students develop skills to analyze complex scenarios and calculate probabilities effectively.	Probability
Definition--Statistics and Probability Concepts--Conditional Probability	Conditional Probability Topic Statistics and Probability Definition Conditional probability is the probability of an event occurring given that another event has already occurred. Description Conditional probability is used in statistics to refine predictions and is essential in fields like epidemiology and finance. For example, the probability of drawing a red card given that is a face card is drawn is a conditional probability. Understanding conditional probability is crucial for students to analyze dependent events and make informed predictions.	Data Analysis