
Illustrative Math Alignment: Grade 7 Unit 7
Expressions, Equations, and Inequalities
Lesson 10: Different Options for Solving One Equation
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|

|
Math Example--Percents--Equations with Percents: Example 20 | Math Example--Percents--Equations with Percents: Example 20TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "32 is what percent of 1.5?" The solution involves setting up the equation 1.5 * (x / 100) = 32, then solving for x to get x = 32 * (100 / 1.5), which equals 2133.3%. This example introduces a scenario where the resulting percentage is significantly larger than 100% and involves a decimal base number less than 1. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 21 | Math Example--Percents--Equations with Percents: Example 21TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "48 is what percent of 55?" The solution involves setting up the equation 55 * (x / 100) = 48, then solving for x to get x = 48 * (100 / 55), which equals 87.27%. This example demonstrates how to calculate a percentage when the two numbers are relatively close in value, resulting in a percentage close to but less than 100%. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 22 | Math Example--Percents--Equations with Percents: Example 22TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "52.2 is what percent of 98.5?" The solution involves setting up the equation 98.5 * (x / 100) = 52.2, then solving for x to get x = 52.2 * (100 / 98.5), which is approximately 52.99%. This example introduces a scenario where both the numerator and denominator are decimal numbers, resulting in a percentage that is also close to the original numerator. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 23 | Math Example--Percents--Equations with Percents: Example 23TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "68 is what percent of 320?" The solution involves setting up the equation 320 * (x / 100) = 68, then solving for x to get x = 68 * (100 / 320), which equals 21.25%. This example demonstrates how to calculate a percentage when dealing with whole numbers, resulting in a percentage that's less than 25%. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 24 | Math Example--Percents--Equations with Percents: Example 24TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "75.5 is what percent of 555.25?" The solution involves setting up the equation 555.25 * (x / 100) = 75.5, then solving for x to get x = 75.5 * (100 / 555.25), which is approximately 13.59%. This example introduces a scenario where both the numerator and denominator are decimal numbers, resulting in a percentage that's less than 15%. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 25 | Math Example--Percents--Equations with Percents: Example 25TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "125 is what percent of 2?" The solution involves setting up the equation 2 * (x / 100) = 125, then solving for x to get x = 125 * (100 / 2), which equals 6250%. This example demonstrates how to calculate a percentage when the first number is significantly larger than the second, resulting in a percentage well over 100%. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 26 | Math Example--Percents--Equations with Percents: Example 26TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "300 is what percent of 3.5?" The solution involves setting up the equation 3.5 * (x / 100) = 300, then solving for x to get x = 300 * (100 / 3.5), which equals 8571.43%. This example introduces a scenario where the resulting percentage is extremely large, over 8000%, due to the first number being significantly larger than the small decimal base number. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 27 | Math Example--Percents--Equations with Percents: Example 27TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "278 is what percent of 99?" The solution involves setting up the equation 99 * (x / 100) = 278, then solving for x to get x = 278 * (100 / 99), which equals 280.80%. This example demonstrates how to calculate a percentage when the first number is significantly larger than the second, resulting in a percentage greater than 200%. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 28 | Math Example--Percents--Equations with Percents: Example 28TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "300 is what percent of 75.5?" The solution involves setting up the equation 75.5 * (x / 100) = 300, then solving for x to get x = 300 * (100 / 75.5), which equals 397.35%. This example introduces a scenario where the resulting percentage is close to 400%, with the first number being significantly larger than the decimal base number. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 29 | Math Example--Percents--Equations with Percents: Example 29TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "400 is what percent of 220?" The solution involves setting up the equation 220 * (x / 100) = 400, then solving for x to get x = 400 * (100 / 220), which equals 181.81%. This example demonstrates how to calculate a percentage when the first number is nearly double the second, resulting in a percentage between 150% and 200%. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 3 | Math Example--Percents--Equations with Percents: Example 3TopicSolving Equations DescriptionThis math example focuses on solving percent equations, specifically asking "What is 8% of 58?" The solution involves converting 8% to its decimal form, 0.08, and then multiplying it by 58 to arrive at the answer of 4.64. This example introduces a larger whole number as the base value, demonstrating the scalability of the percent-to-decimal conversion method. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 30 | Math Example--Percents--Equations with Percents: Example 30TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "333.5 is what percent of 500.25?" The solution involves setting up the equation 500.25 * (x / 100) = 333.5, then solving for x to get x = 333.5 * (100 / 500.25), which is approximately 66.67%. This example introduces a scenario where both numbers are decimals and the resulting percentage is less than 100%, showing how to handle more complex decimal calculations. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 31 | Math Example--Percents--Equations with Percents: Example 31TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "4 is 0.1% of what number?" The solution involves setting up the equation 4 = 0.001 * x, then solving for x to get x = 4 / 0.001, which equals 4000. This example demonstrates how to calculate the whole when given a very small percentage of it, resulting in a much larger number. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 32 | Math Example--Percents--Equations with Percents: Example 32TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "7 is 1% of what number?" The solution involves setting up the equation 7 = 0.01 * x, then solving for x to get x = 7 / 0.01, which equals 700. This example introduces a scenario where we need to find the whole when given a small percentage of it, resulting in a number 100 times larger than the given value. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 33 | Math Example--Percents--Equations with Percents: Example 33TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "9 is 30% of what number?" The solution involves setting up the equation 9 = 0.3 * x, then solving for x to get x = 9 / 0.3, which equals 30. This example demonstrates how to calculate the whole when given a larger percentage of it, resulting in a number that is only slightly larger than the given value. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 34 | Math Example--Percents--Equations with Percents: Example 34TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "1 is 400% of what number?" The solution involves setting up the equation 1 = 4.0 * x, then solving for x to get x = 1 / 4, which equals 0.25. This example introduces a scenario where we need to find a number that, when increased by 400%, results in 1, leading to a fraction or decimal less than 1. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 35 | Math Example--Percents--Equations with Percents: Example 35TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "15 is 0.25% of what number?" The solution involves setting up the equation 15 = 0.0025 * x, then solving for x to get x = 15 / 0.0025, which equals 6000. This example introduces a scenario where we need to find the whole when given a very small percentage of it, resulting in a number that is significantly larger than the given value. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 36 | Math Example--Percents--Equations with Percents: Example 36TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "30 is 5% of what number?" The solution involves setting up the equation 30 = 0.05 * x, then solving for x to get x = 30 / 0.05, which equals 600. This example demonstrates how to calculate the whole when given a small percentage of it, resulting in a much larger number. |
Solving Percent Equations |
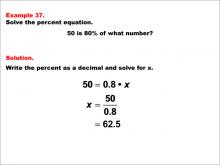
|
Math Example--Percents--Equations with Percents: Example 37 | Math Example--Percents--Equations with Percents: Example 37TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "50 is 80% of what number?" The solution involves setting up the equation 50 = 0.8 * x, then solving for x to get x = 50 / 0.8, which equals 62.5. This example introduces a scenario where we need to find the whole when given a large percentage of it, resulting in a number that is only slightly larger than the given value. |
Solving Percent Equations |
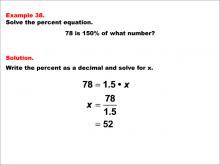
|
Math Example--Percents--Equations with Percents: Example 38 | Math Example--Percents--Equations with Percents: Example 38TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "78 is 150% of what number?" The solution involves setting up the equation 78 = 1.5 * x, then solving for x to get x = 78 / 1.5, which equals 52. This example demonstrates how to calculate the original value when given a percentage greater than 100%, resulting in a number that is smaller than the given value. |
Solving Percent Equations |
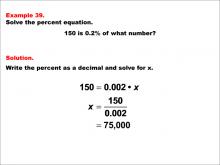
|
Math Example--Percents--Equations with Percents: Example 39 | Math Example--Percents--Equations with Percents: Example 39TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "150 is 0.2% of what number?" The solution involves setting up the equation 150 = 0.002 * x, then solving for x to get x = 150 / 0.002, which equals 75,000. This example introduces a scenario where we need to find the whole when given a very small percentage of it, resulting in a number that is significantly larger than the given value. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 4 | Math Example--Percents--Equations with Percents: Example 4TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "What is 6.5% of 45.5?" The solution involves converting 6.5% to its decimal equivalent, 0.065, and then multiplying it by 45.5 to obtain the result of 2.9575. This example introduces both a decimal percentage and a decimal base number, adding complexity to the calculation. |
Solving Percent Equations |
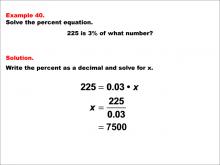
|
Math Example--Percents--Equations with Percents: Example 40 | Math Example--Percents--Equations with Percents: Example 40TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "225 is 3% of what number?" The solution involves setting up the equation 225 = 0.03 * x, then solving for x to get x = 225 / 0.03, which equals 7,500. This example demonstrates how to calculate the whole when given a small percentage of it, resulting in a number that is significantly larger than the given value. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 41 | Math Example--Percents--Equations with Percents: Example 41TopicSolving Equations DescriptionThis math example focuses on solving percent equations by asking "400 is 40% of what number?" The solution involves setting up the equation 400 = 0.4 * x, then solving for x to get x = 400 / 0.4, which equals 1000. This example demonstrates how to calculate the whole when given a significant percentage of it, resulting in a number that is larger than the given value. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 42 | Math Example--Percents--Equations with Percents: Example 42TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "650 is 130% of what number?" The solution involves setting up the equation 650 = 1.3 * x, then solving for x to get x = 650 / 1.3, which equals 500. This example introduces a scenario where we need to find the original value when given a percentage greater than 100%, resulting in a number that is smaller than the given value. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 5 | Math Example--Percents--Equations with Percents: Example 5TopicSolving Equations DescriptionThis math example focuses on solving percent equations, specifically asking "What is 30% of 9?" The solution involves converting 30% to its decimal form, 0.3, and then multiplying it by 9 to get the result of 2.7. This example introduces a larger percentage, demonstrating how the method applies consistently across various percentage values. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 6 | Math Example--Percents--Equations with Percents: Example 6TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "What is 28% of 7.2?" The solution involves converting 28% to its decimal equivalent, 0.28, and then multiplying it by 7.2 to obtain the result of 2.016. This example combines a whole number percentage with a decimal base number, further illustrating the versatility of the percent-to-decimal conversion method. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 7 | Math Example--Percents--Equations with Percents: Example 7TopicSolving Equations DescriptionThis math example focuses on solving percent equations, specifically asking "What is 45% of 68?" The solution involves converting 45% to its decimal form, 0.45, and then multiplying it by 68 to arrive at the answer of 30.6. This example introduces a larger percentage and a larger whole number as the base value, demonstrating the scalability of the percent-to-decimal conversion method. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 8 | Math Example--Percents--Equations with Percents: Example 8TopicSolving Equations DescriptionThis math example demonstrates solving percent equations by asking "What is 52.3% of 36.9?" The solution involves converting 52.3% to its decimal equivalent, 0.523, and then multiplying it by 36.9 to obtain the result of 19.2987. This example introduces both a decimal percentage and a decimal base number, adding complexity to the calculation and showcasing the versatility of the percent-to-decimal conversion method. |
Solving Percent Equations |

|
Math Example--Percents--Equations with Percents: Example 9 | Math Example--Percents--Equations with Percents: Example 9TopicSolving Equations DescriptionThis math example focuses on solving percent equations, specifically asking "What is 150% of 8?" The solution involves converting 150% to its decimal form, 1.5, and then multiplying it by 8 to get the result of 12. This example introduces a percentage greater than 100%, demonstrating how the method applies consistently even when dealing with percentages that represent values larger than the whole. |
Solving Percent Equations |
|
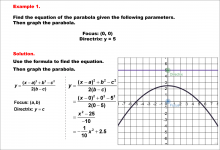
Math Example--Quadratics--Equations of Parabolas--Example 1 | Equations of Parabolas--Example 1TopicQuadratics DescriptionIn this example, we're given a parabola with its focus at (0, 0) and directrix y = 5. Using the locus definition, we can determine that the vertex is at (0, 2.5), halfway between the focus and directrix. The distance p from the vertex to the focus is 2.5. This example demonstrates how the focus and directrix directly determine the equation of the parabola. For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection. |
Graphs of Quadratic Functions |
|
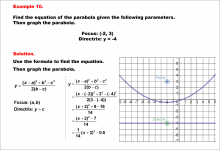
Math Example--Quadratics--Equations of Parabolas--Example 10 | Equations of Parabolas--Example 10TopicQuadratics |
Graphs of Quadratic Functions |
|
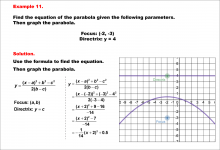
Math Example--Quadratics--Equations of Parabolas--Example 11 | Equations of Parabolas--Example 11TopicQuadratics |
Graphs of Quadratic Functions |
|
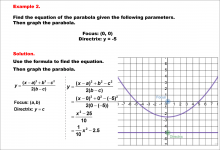
Math Example--Quadratics--Equations of Parabolas--Example 2 | Equations of Parabolas--Example 2TopicQuadratics DescriptionThis example presents a parabola with its focus at (0, 0) and directrix x = -4. The vertex is at (0, -2.5), midway between the focus and directrix. The distance p from the vertex to the focus is 2.5. This example illustrates how a vertically-oriented parabola's equation is derived from its focus and directrix. For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection. |
Graphs of Quadratic Functions |
|
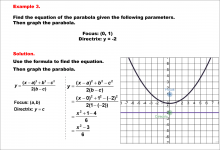
Math Example--Quadratics--Equations of Parabolas--Example 3 | Equations of Parabolas--Example 3TopicQuadratics DescriptionIn this example, the parabola has its focus at (0, 1) and directrix y = -2. The vertex is at (0, -0.5), and the parabola opens upward. The distance p from the vertex to the focus is 1.5. This example reinforces the relationship between the focus, directrix, and the resulting equation of the parabola. For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection. |
Graphs of Quadratic Functions |
|
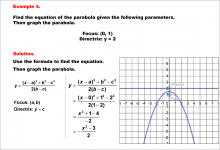
Math Example--Quadratics--Equations of Parabolas--Example 4 | Equations of Parabolas--Example 4TopicQuadratics DescriptionThis example shows a parabola with its focus at (0, 1) and directrix y = 2. The parabola opens downward with its vertex at (0, 1.5). The distance p from the vertex to the focus is 0.5. This example illustrates how the position of the focus and directrix determines the orientation and equation of the parabola. For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection. |
Graphs of Quadratic Functions |
|
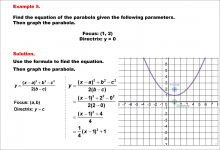
Math Example--Quadratics--Equations of Parabolas--Example 5 | Equations of Parabolas--Example 5TopicQuadratics DescriptionIn this example, the parabola has its focus at (1, 2) and directrix y = 0. The vertex is at (1, 1), and the parabola opens upward. The distance p from the vertex to the focus is 1. For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection. |
Graphs of Quadratic Functions |
|
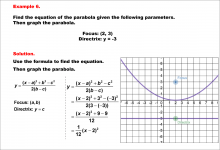
Math Example--Quadratics--Equations of Parabolas--Example 6 | Equations of Parabolas--Example 6TopicQuadratics |
Graphs of Quadratic Functions |
|
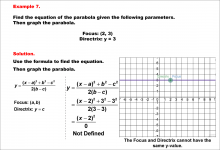
Math Example--Quadratics--Equations of Parabolas--Example 7 | Equations of Parabolas--Example 7TopicQuadratics DescriptionThis example presents a parabola with its focus at (2, 3) and directrix y = 3. This parabola is not defined because the focus and directrix cannot have the same y-value. For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection. |
Graphs of Quadratic Functions |
|
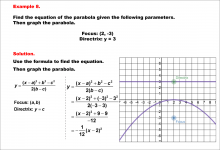
Math Example--Quadratics--Equations of Parabolas--Example 8 | Equations of Parabolas--Example 8TopicQuadratics |
Graphs of Quadratic Functions |

|
Math Example--Quadratics--Equations of Parabolas--Example 9 | Equations of Parabolas--Example 9TopicQuadratics |
Graphs of Quadratic Functions |
|
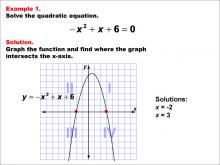
Math Example--Quadratics--Graphical Solutions to Quadratic Equations: Example 1 | Graphical Solutions to Quadratic Equations: Example 1TopicQuadratics |
Quadratic Equations and Functions |
|
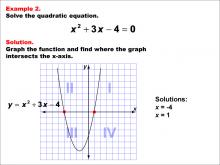
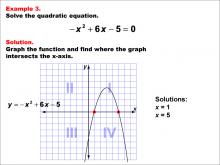
Math Example--Quadratics--Graphical Solutions to Quadratic Equations: Example 2 | Graphical Solutions to Quadratic Equations: Example 2TopicQuadratics |
Quadratic Equations and Functions |
|
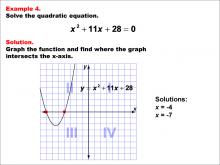
Math Example--Quadratics--Graphical Solutions to Quadratic Equations: Example 3 | Graphical Solutions to Quadratic Equations: Example 3TopicQuadratics |
Quadratic Equations and Functions |
|
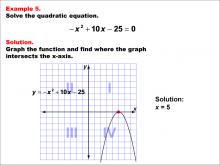
Math Example--Quadratics--Graphical Solutions to Quadratic Equations: Example 4 | Graphical Solutions to Quadratic Equations: Example 4TopicQuadratics DescriptionThis example illustrates a parabola intersecting the x-axis at two distinct points, indicating two real and distinct solutions. The graph provides a visual representation of the roots and helps in understanding the impact of the quadratic equation's coefficients on the shape and position of the parabola. Graphical solutions are advantageous for quickly identifying the number of solutions, but they may not offer precise numerical solutions without additional calculations. |
Quadratic Equations and Functions |
|
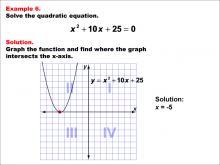
Math Example--Quadratics--Graphical Solutions to Quadratic Equations: Example 5 | Graphical Solutions to Quadratic Equations: Example 5TopicQuadratics DescriptionThis example shows a parabola that is tangent to the x-axis, indicating a single real solution. This scenario occurs when the quadratic equation has a perfect square trinomial, resulting in a discriminant of zero. The graphical approach provides an intuitive understanding of why there is only one solution. However, it may not be sufficient for finding the exact solution without algebraic methods, especially if the vertex is not at a simple coordinate. |
Quadratic Equations and Functions |
|
Math Example--Quadratics--Graphical Solutions to Quadratic Equations: Example 6 | Graphical Solutions to Quadratic Equations: Example 6TopicQuadratics DescriptionThis example shows a parabola that is tangent to the x-axis, indicating a single real solution. This scenario occurs when the quadratic equation has a perfect square trinomial, resulting in a discriminant of zero. The graphical approach provides an intuitive understanding of why there is only one solution. However, it may not be sufficient for finding the exact solution without algebraic methods, especially if the vertex is not at a simple coordinate. |
Quadratic Equations and Functions |
|
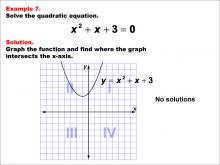
Math Example--Quadratics--Graphical Solutions to Quadratic Equations: Example 7 | Graphical Solutions to Quadratic Equations: Example 7TopicQuadratics DescriptionThis example illustrates a quadratic graph with no x-intercepts, indicating no real solutions. The graph provides a straightforward way to see where the solutions lie on the x-axis. This method is particularly advantageous for visual learners and for gaining a conceptual understanding of the roots. However, it may not always provide precise solutions, especially if the intercepts are not integers or easily readable from the graph. |
Quadratic Equations and Functions |
|
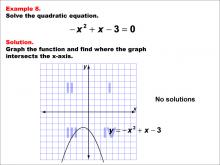
Math Example--Quadratics--Graphical Solutions to Quadratic Equations: Example 8 | Graphical Solutions to Quadratic Equations: Example 8TopicQuadratics DescriptionThis example shows a parabola not intersecting the x-axis, indicating no real solutions. The graph helps in visualizing the solutions and understanding how the quadratic equation's coefficients affect the graph's orientation and position. Graphical solutions are beneficial for quickly identifying the nature of the solutions, but they may not provide exact numerical answers without further analytical work. |
Quadratic Equations and Functions |

|
Math Example--Quadratics--Solving Simple Quadratic Equations--Example 1 | Solving Simple Quadratic Equations--Example 1TopicQuadratics DescriptionThis example demonstrates solving a simplified form of a quadratic equation. The equation is in the form ax² = c, where the linear term (bx) is absent. This simplification allows for a straightforward solution by isolating x² and then taking the square root of both sides. The process is more direct than using the quadratic formula, making it easier to solve mentally or with minimal calculations. This method highlights the importance of recognizing special forms of quadratic equations for efficient problem-solving. |
Quadratic Equations and Functions |