
Illustrative Math Alignment: Grade 7 Unit 7
Angles, Triangles, and Prisms
Lesson 8: Triangles with 3 Common Measures
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Nodes |
|---|---|---|---|
|
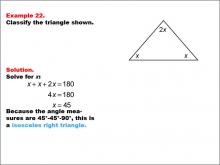
Math Example--Polygons--Triangle Classification: Example 22 | Math Example--Polygons--Triangle Classification: Example 22TopicTriangles DescriptionThis example shows a triangle with two equal angles labeled x and a third angle labeled 2x. Solving for x, we find the angles to be 45°, 45°, and 90°, classifying it as an isosceles right triangle. Triangles are classified by their angle measures or side lengths. Right triangles have a 90° angle, and isosceles triangles have two congruent angles. Reviewing examples like this one helps students understand combined classifications. |
Definition of a Triangle |
|
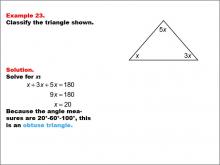
Math Example--Polygons--Triangle Classification: Example 23 | Math Example--Polygons--Triangle Classification: Example 23TopicTriangles DescriptionThis example shows a triangle with angles labeled x, 3x, and 5x. Solving for x, we find the angles to be 20°, 60°, and 100°, which classifies this triangle as obtuse because one of the angles is greater than 90°. Triangles can be classified by angle measures, with obtuse triangles defined as having one angle greater than 90°. Observing examples like this aids students in understanding how angle measures determine classifications. Providing multiple worked-out examples reinforces students' ability to consistently classify triangles based on angle properties. |
Definition of a Triangle |
|
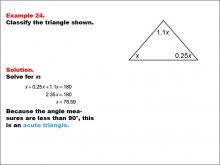
Math Example--Polygons--Triangle Classification: Example 24 | Math Example--Polygons--Triangle Classification: Example 24TopicTriangles DescriptionThis example shows a triangle with angles labeled as x, 0.25x, and 1.1x. Solving for x, we find that all angles are less than 90°, classifying the triangle as acute. Triangles are classified by their angle measures or side lengths, with acute triangles having all angles less than 90°. Reviewing examples like this helps students reinforce the criteria for classifying triangles based on angle sizes. Seeing multiple worked-out examples builds students' confidence in applying classification rules and identifying triangle types based on consistent properties. |
Definition of a Triangle |

|
Math Example--Polygons--Triangle Classification: Example 3 | Math Example--Polygons--Triangle Classification: Example 3TopicTriangles DescriptionThis example shows a triangle with side lengths of 12, 18, and x, where x is not equal to 12 or 18. The triangle is classified as scalene because all three side lengths are different. Triangles are categorized by side length or angle. Scalene triangles, with all sides unequal, demonstrate a key classification type. Multiple examples help students recognize classification patterns for triangles based on side length or angle measure. Observing various worked-out examples reinforces consistent application of classification criteria, building student confidence in identifying triangle types. |
Definition of a Triangle |
|
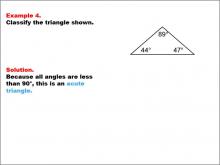
Math Example--Polygons--Triangle Classification: Example 4 | Math Example--Polygons--Triangle Classification: Example 4TopicTriangles DescriptionThis example displays a triangle with angles measuring 89°, 44°, and 47°. Since all angles are less than 90°, the triangle is classified as acute. Triangle classification can be based on side length or angle measures. Acute triangles have all angles less than 90°, as shown in this example. Reviewing multiple examples in this way helps students visually distinguish triangle types by angle. Providing varied worked-out examples solidifies students’ understanding of the criteria for each triangle type, enabling them to accurately identify triangles by their angle measures. |
Definition of a Triangle |
|
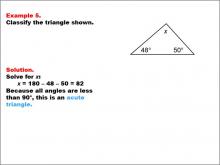
Math Example--Polygons--Triangle Classification: Example 5 | Math Example--Polygons--Triangle Classification: Example 5TopicTriangles DescriptionThis example presents a triangle with angles of 48°, 50°, and an unknown angle x. Solving for x, we find that x = 82°, meaning all angles are less than 90°, classifying it as acute. Triangles can be identified by their angles or side lengths. Acute triangles have all angles less than 90°, as seen here. Reviewing examples like this supports student understanding of angle-based classification. Seeing multiple worked examples helps students recognize patterns and confidently classify triangles based on established criteria. |
Definition of a Triangle |
|
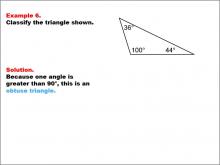
Math Example--Polygons--Triangle Classification: Example 6 | Math Example--Polygons--Triangle Classification: Example 6TopicTriangles DescriptionThis example shows a triangle with angles of 36°, 100°, and 44°. Because one of the angles is greater than 90°, this triangle is classified as obtuse. Triangles are classified by side lengths or angles. An obtuse triangle has one angle greater than 90°, as seen here. This collection of examples supports students in identifying and classifying triangles based on distinct angle measures. Seeing multiple worked-out examples reinforces students' understanding of classification rules, making it easier to recognize triangle types based on angle or side length. |
Definition of a Triangle |
|
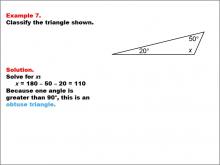
Math Example--Polygons--Triangle Classification: Example 7 | Math Example--Polygons--Triangle Classification: Example 7TopicTriangles DescriptionThis example presents a triangle with two known angles, 20° and 50°, and an unknown angle x. Solving for x, we find it to be 110°. Since one angle is greater than 90°, the triangle is classified as obtuse. Triangles can be classified by their angles, where obtuse triangles have one angle over 90°. Reviewing examples like this aids students in understanding classification based on angle measures. Seeing multiple examples reinforces the rules and helps students apply classification consistently and accurately. |
Definition of a Triangle |
|
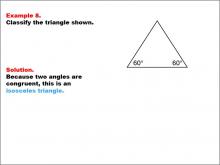
Math Example--Polygons--Triangle Classification: Example 8 | Math Example--Polygons--Triangle Classification: Example 8TopicTriangles DescriptionThis example shows a triangle with two angles, each measuring 60°. Since two angles are congruent, this triangle is classified as isosceles. Triangles can be categorized by side or angle properties. Isosceles triangles have two equal sides or two equal angles, as illustrated here. This collection of examples allows students to observe classifications and understand patterns in triangle properties. Examining multiple examples helps students confidently identify triangle types based on consistent characteristics. |
Definition of a Triangle |
|
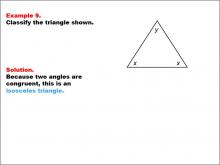
Math Example--Polygons--Triangle Classification: Example 9 | Math Example--Polygons--Triangle Classification: Example 9TopicTriangles DescriptionThis example shows a triangle with two angles labeled x and one angle labeled y. Since two angles are congruent, the triangle is classified as isosceles. Triangles can be classified by side lengths or angle measures. Isosceles triangles, with two equal sides or angles, are common. Observing various examples helps students understand these classification criteria. Providing multiple examples allows students to identify patterns in side and angle relationships, reinforcing accurate triangle classification. |
Definition of a Triangle |
|
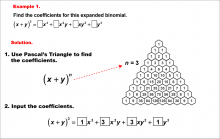
Math Example--Polynomial Concepts--Pascal's Triangle: Example 1 | Math Example--Polynomial Concepts--Pascal's Triangle: Example 1TopicPolynomials DescriptionIn this mathematical exploration, we delve into Pascal's Triangle to find coefficients for the binomial expansion of (x + y)^3. The example focuses on determining the coefficients for the expanded expression (x + y)3 = _x3 + _x2y + _xy2 + _y3, where the blanks represent the coefficients we need to find. |
Polynomial Expressions |
|
Math Example--Polynomial Concepts--Pascal's Triangle: Example 2 | Math Example--Polynomial Concepts--Pascal's Triangle: Example 2TopicPolynomials DescriptionThis example explores the binomial expansion of (x + y)4 using Pascal's Triangle. We aim to find the coefficients for the expanded expression (x + y)4 = _x4 + _x3y + _x2y2 + _xy3 + _y4, where the blanks represent the coefficients we need to determine. |
Polynomial Expressions |

|
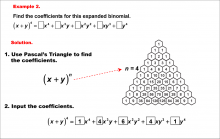
Math Example--Polynomial Concepts--Pascal's Triangle: Example 3 | Math Example--Polynomial Concepts--Pascal's Triangle: Example 3TopicPolynomials DescriptionIn this example, we explore the binomial expansion of (x + y)5 using Pascal's Triangle. Our goal is to determine the coefficients for the expanded expression (x + y)5 = _x5 + _x4y + _x3y2 + _x2y3 + _xy4 + _y5, where the blanks represent the coefficients we need to find. |
Polynomial Expressions |
|
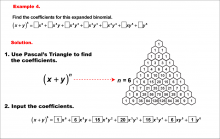
Math Example--Polynomial Concepts--Pascal's Triangle: Example 4 | Math Example--Polynomial Concepts--Pascal's Triangle: Example 4TopicPolynomials DescriptionThis example focuses on the binomial expansion of (x + y)^6 using Pascal's Triangle. We aim to find the coefficients for the expanded expression (x + y)6 = _x6 + _x5y + _x4y2 + _x3y3 + _x2y4 + _xy5 + _y6, where the blanks represent the coefficients we need to determine. |
Polynomial Expressions |

|
Math Example--Polynomial Concepts--Pascal's Triangle: Example 5 | Math Example--Polynomial Concepts--Pascal's Triangle: Example 5TopicPolynomials DescriptionIn this final example, we explore the binomial expansion of (x + y)7 using Pascal's Triangle. Our objective is to determine the coefficients for the expanded expression (x + y)7 = _x7 + _x6y + _x5y2 + _x4y3 + _x3y4 + _x2y5 + _xy6 + _y7, where the blanks represent the coefficients we need to find. |
Polynomial Expressions |

|
Math Example--Right Triangles-- Example 1 | Math Example--Right Triangles-- Example 1TopicRight Triangles DescriptionThis example presents a right triangle with sides of length 8 and 10, and an unknown hypotenuse labeled c. The task is to find the value of c using the Pythagorean Theorem. By applying the formula a2 + b2 = c2, we can calculate that c = √(102 + 82) = √(164) = 2 * √(41). |
Right Triangles |
|
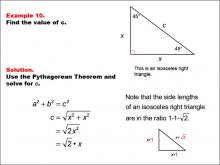
Math Example--Right Triangles-- Example 10 | Math Example--Right Triangles-- Example 10TopicRight Triangles |
Right Triangles |

|
Math Example--Right Triangles-- Example 11 | Math Example--Right Triangles-- Example 11TopicRight Triangles |
Right Triangles |

|
Math Example--Right Triangles-- Example 12 | Math Example--Right Triangles-- Example 12TopicRight Triangles |
Right Triangles |

|
Math Example--Right Triangles-- Example 13 | Math Example--Right Triangles-- Example 13TopicRight Triangles |
Right Triangles |
|
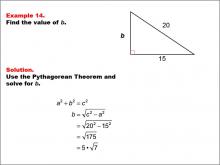
Math Example--Right Triangles-- Example 14 | Math Example--Right Triangles-- Example 14TopicRight Triangles |
Right Triangles |
|
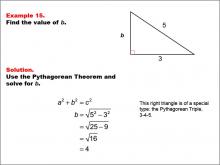
Math Example--Right Triangles-- Example 15 | Math Example--Right Triangles-- Example 15TopicRight Triangles |
Right Triangles |
|
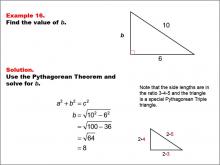
Math Example--Right Triangles-- Example 16 | Math Example--Right Triangles-- Example 16TopicRight Triangles |
Right Triangles |
|
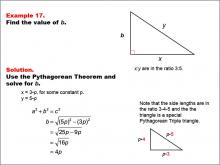
Math Example--Right Triangles-- Example 17 | Math Example--Right Triangles-- Example 17TopicRight Triangles |
Right Triangles |
|
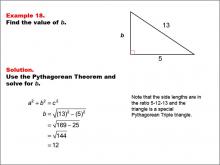
Math Example--Right Triangles-- Example 18 | Math Example--Right Triangles-- Example 18TopicRight Triangles |
Right Triangles |

|
Math Example--Right Triangles-- Example 19 | Math Example--Right Triangles-- Example 19TopicRight Triangles |
Right Triangles |
|
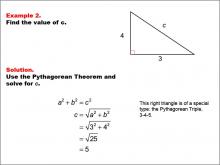
Math Example--Right Triangles-- Example 2 | Math Example--Right Triangles-- Example 2TopicRight Triangles DescriptionIn this example, we have a right triangle with sides of length 3 and 4, and an unknown hypotenuse labeled c. The goal is to determine the value of c using the Pythagorean Theorem. By applying the formula a2 + b2 = c2, we can calculate that c = √(32 + 42) = √(25) = 5. This example builds upon the previous one, reinforcing the application of the Pythagorean Theorem in right triangles. It demonstrates how the theorem can be used with different side lengths, helping students understand its versatility in solving various right triangle problems. |
Right Triangles |
|
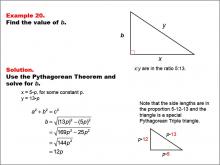
Math Example--Right Triangles-- Example 20 | Math Example--Right Triangles-- Example 20TopicRight Triangles |
Right Triangles |

|
Math Example--Right Triangles-- Example 21 | Math Example--Right Triangles-- Example 21TopicRight Triangles |
Right Triangles |
|
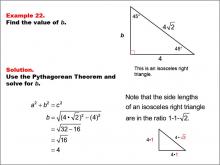
Math Example--Right Triangles-- Example 22 | Math Example--Right Triangles-- Example 22TopicRight Triangles |
Right Triangles |
|
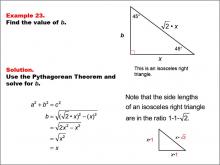
Math Example--Right Triangles-- Example 23 | Math Example--Right Triangles-- Example 23TopicRight Triangles |
Right Triangles |

|
Math Example--Right Triangles-- Example 24 | Math Example--Right Triangles-- Example 24TopicRight Triangles |
Right Triangles |

|
Math Example--Right Triangles-- Example 25 | Math Example--Right Triangles-- Example 25TopicRight Triangles |
Right Triangles |

|
Math Example--Right Triangles-- Example 26 | Math Example--Right Triangles-- Example 26TopicRight Triangles |
Right Triangles |

|
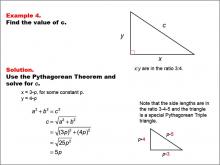
Math Example--Right Triangles-- Example 3 | Math Example--Right Triangles-- Example 3TopicRight Triangles |
Right Triangles |
|
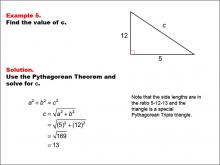
Math Example--Right Triangles-- Example 4 | Math Example--Right Triangles-- Example 4TopicRight Triangles |
Right Triangles |
|
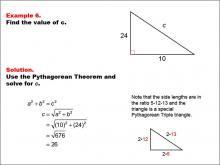
Math Example--Right Triangles-- Example 5 | Math Example--Right Triangles-- Example 5TopicRight Triangles |
Right Triangles |
|
Math Example--Right Triangles-- Example 6 | Math Example--Right Triangles-- Example 6TopicRight Triangles |
Right Triangles |

|
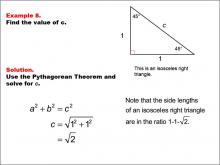
Math Example--Right Triangles-- Example 7 | Math Example--Right Triangles-- Example 7TopicRight Triangles |
Right Triangles |
|
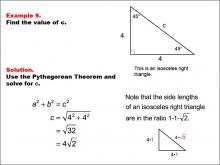
Math Example--Right Triangles-- Example 8 | Math Example--Right Triangles-- Example 8TopicRight Triangles |
Right Triangles |
|
Math Example--Right Triangles-- Example 9 | Math Example--Right Triangles-- Example 9TopicRight Triangles |
Right Triangles |
|
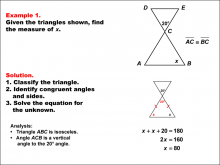
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 1 | Solving Equations Using Triangle Properties: Example 1TopicEquations DescriptionThis example focuses on solving equations using the properties of similar isosceles triangles. Isosceles triangles are characterized by having two equal sides and two equal base angles. In this case, we have two similar isosceles triangles, which means they share the same shape but may differ in size. The equation to be solved involves finding the unknown angle x, given that one of the angles is 20°. The property of vertical angles tells us that the angle vertical to the 20° angle is also 20° |
Applications of Equations and Inequalities and Applications of Triangles |
|
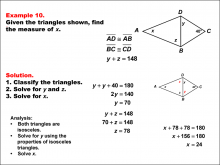
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 10 | Solving Equations Using Triangle Properties: Example 10TopicEquations DescriptionThis example, similar to Example 9, involves solving equations using the properties of a kite and applying the exterior angle theorem. We are again given one angle of 40° and two unknown angles, y and x. The goal is to set up and solve equations to find the values of y and x using the properties of kites and the exterior angle theorem. Key properties to consider: |
Applications of Equations and Inequalities and Applications of Triangles |
|
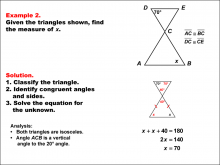
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 2 | Solving Equations Using Triangle Properties: Example 2TopicEquations DescriptionThis example explores solving equations using the properties of similar isosceles triangles, building upon the concepts introduced in Example 1. In this case, we have two similar isosceles triangles with one known angle of 70° and an unknown angle x. The goal is to determine the value of x using triangle properties and algebraic techniques. |
Applications of Equations and Inequalities and Applications of Triangles |
|
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 3 | Solving Equations Using Triangle Properties: Example 3TopicEquations DescriptionThis example focuses on solving equations involving parallel lines cut by a transversal, a fundamental concept in geometry. The problem presents two parallel lines intersected by two transversals that also form a triangle. We are given that one angle measures 120° and the corresponding angle can be expressed as (y + 40)°. The goal is to determine the value of y using the properties of angles formed by parallel lines and a transversal. When parallel lines are cut by a transversal, several important angle relationships are formed: |
Applications of Equations and Inequalities and Applications of Triangles |

|
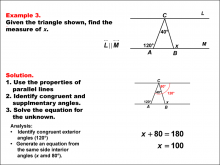
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 4 | Solving Equations Using Triangle Properties: Example 4TopicEquations DescriptionThis example demonstrates solving equations using the Exterior Angle Theorem in the context of parallel lines cut by a transversal, two crucial concepts in geometry. The problem presents a triangle with two known interior angles of 80° and y, and an unknown exterior angle x°. We are also given that 80 - y = 50, which simplifies to y = 30. The goal is to determine the value of x using the properties of triangles and the Exterior Angle Theorem. The Exterior Angle Theorem states that an exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles. Wo we get x = 80 + 30, or x = 110. |
Applications of Equations and Inequalities and Applications of Triangles |
|
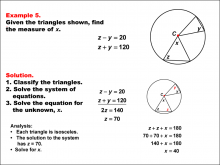
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 5 | Solving Equations Using Triangle Properties: Example 5TopicEquations DescriptionThis example focuses on solving equations involving isosceles triangles centered in a circle. The problem presents two equations: z - y = 20 and z + y = 120, where z and y represent angles in the isosceles triangles. The goal is to solve this system of equations to find the values of z and y, utilizing properties of isosceles triangles and circles. |
Applications of Equations and Inequalities and Applications of Triangles |

|
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 6 | Solving Equations Using Triangle Properties: Example 6TopicEquations DescriptionThis example explores solving equations involving triangles that share a vertex at the center of a circle. We are presented with two equations: x + y = 75 and z + y = 110, where x, y, and z represent angles in the triangle The goal is to solve this system of equations to find the values of x, y, and z, utilizing properties of isosceles triangles. Since each of the triangles is isosceles, we know that z + 55 + 55 = 180 and therefore, z = 70°. We substitute this into one of the equations: 70 + y = 110 7 = 40° |
Applications of Equations and Inequalities and Applications of Triangles |
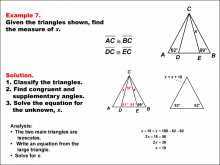
|
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 7 | Solving Equations Using Triangle Properties: Example 7TopicEquations DescriptionThis example focuses on solving equations involving isosceles triangles with a common vertex and base. We are given two angles, 62° and 99°, and need to find the unknown angle x. This problem demonstrates how to use the properties of isosceles triangles and the sum of angles in a triangle to solve for an unknown angle. Key properties to consider: |
Applications of Equations and Inequalities and Applications of Triangles |
|
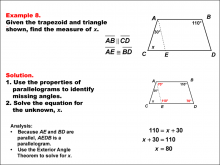
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 8 | Solving Equations Using Triangle Properties: Example 8TopicEquations DescriptionThis example involves solving an equation using the properties of a trapezoid with an embedded parallelogram and applying the exterior angle theorem. We are given two angles, 30° and 110°, and need to find the unknown angle x. This problem demonstrates the application of multiple geometric concepts to solve a complex equation. Key properties to consider: |
Applications of Equations and Inequalities and Applications of Triangles |